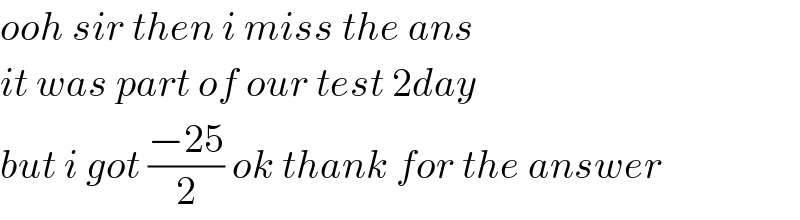Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 28341 by Cheyboy last updated on 24/Jan/18

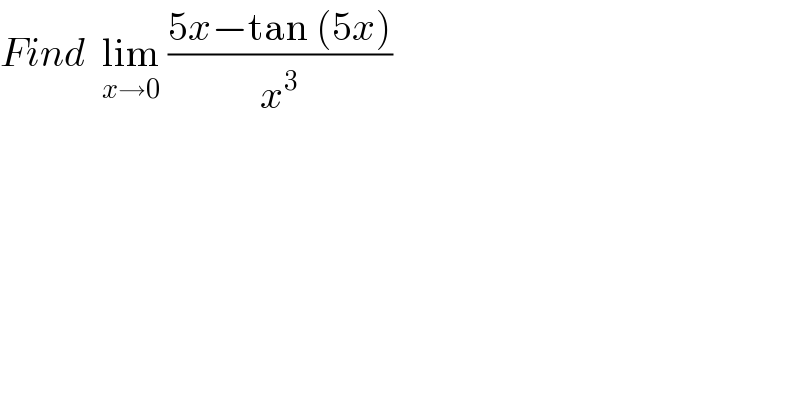
Find lim_(x→0) ((5x−tan (5x))/x^3 )

$${Find}\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{5}{x}−\mathrm{tan}\:\left(\mathrm{5}{x}\right)}{{x}^{\mathrm{3}} } \\ $$

Commented by Cheyboy last updated on 24/Jan/18

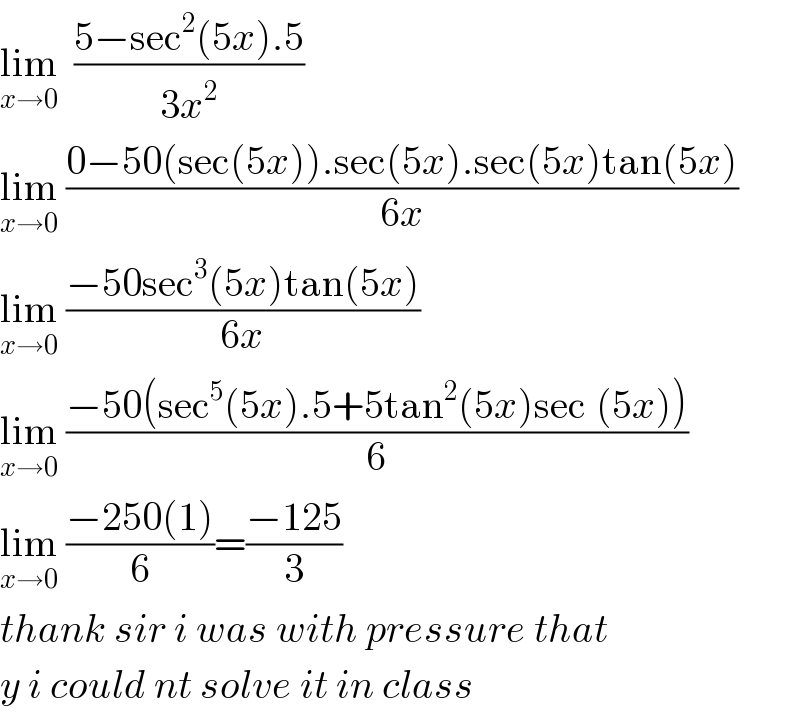
lim_(x→0) ((5−sec^2 (5x).5)/(3x^2 )) lim_(x→0) ((0−50(sec(5x)).sec(5x).sec(5x)tan(5x))/(6x)) lim_(x→0) ((−50sec^3 (5x)tan(5x))/(6x)) lim_(x→0) ((−50(sec^5 (5x).5+5tan^2 (5x)sec^ (5x)))/6) lim_(x→0) ((−250(1))/6)=((−125)/3) thank sir i was with pressure that y i could nt solve it in class

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\:\frac{\mathrm{5}−\mathrm{sec}^{\mathrm{2}} \left(\mathrm{5}{x}\right).\mathrm{5}}{\mathrm{3}{x}^{\mathrm{2}} } \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{0}−\mathrm{50}\left(\mathrm{sec}\left(\mathrm{5}{x}\right)\right).\mathrm{sec}\left(\mathrm{5}{x}\right).\mathrm{sec}\left(\mathrm{5}{x}\right)\mathrm{tan}\left(\mathrm{5}{x}\right)}{\mathrm{6}{x}} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{−\mathrm{50sec}^{\mathrm{3}} \left(\mathrm{5}{x}\right)\mathrm{tan}\left(\mathrm{5}{x}\right)}{\mathrm{6}{x}} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{−\mathrm{50}\left(\mathrm{sec}^{\mathrm{5}} \left(\mathrm{5}{x}\right).\mathrm{5}+\mathrm{5tan}^{\mathrm{2}} \left(\mathrm{5}{x}\right)\mathrm{sec}^{} \left(\mathrm{5}{x}\right)\right)}{\mathrm{6}} \\ $$$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{−\mathrm{250}\left(\mathrm{1}\right)}{\mathrm{6}}=\frac{−\mathrm{125}}{\mathrm{3}} \\ $$$${thank}\:{sir}\:{i}\:{was}\:{with}\:{pressure}\:{that} \\ $$$${y}\:{i}\:{could}\:{nt}\:{solve}\:{it}\:{in}\:{class} \\ $$

Commented by abdo imad last updated on 25/Jan/18

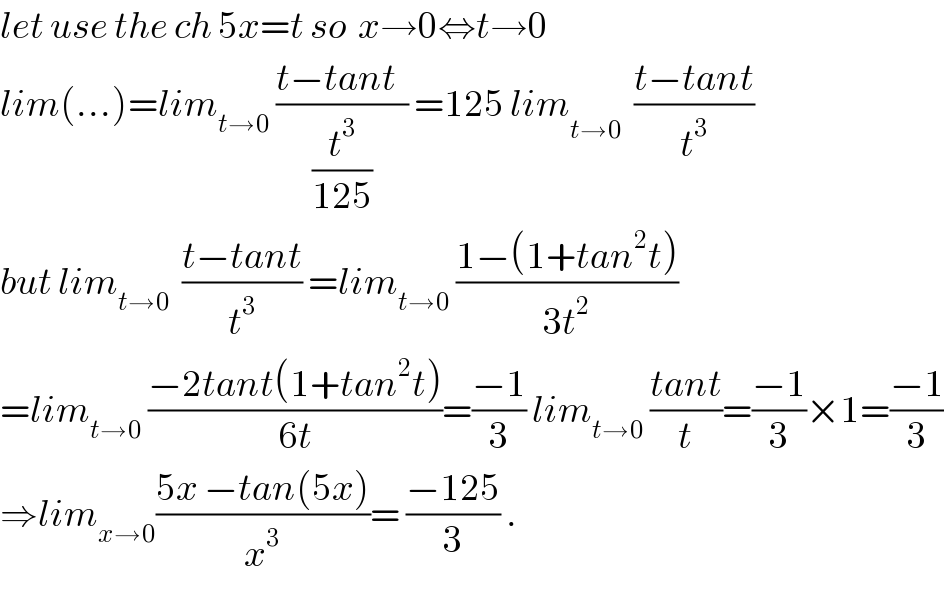
let use the ch 5x=t so x→0⇔t→0 lim(...)=lim_(t→0) ((t−tant )/(t^3 /(125))) =125 lim_(t→0^ ) ((t−tant)/t^3 ) but lim_(t→0) ((t−tant)/t^3 ) =lim_(t→0) ((1−(1+tan^2 t))/(3t^2 )) =lim_(t→0) ((−2tant(1+tan^2 t))/(6t))=((−1)/3) lim_(t→0) ((tant)/t)=((−1)/3)×1=((−1)/3) ⇒lim_(x→0) ((5x −tan(5x))/x^3 )= ((−125)/3) .

$${let}\:{use}\:{the}\:{ch}\:\mathrm{5}{x}={t}\:{so}\:\:{x}\rightarrow\mathrm{0}\Leftrightarrow{t}\rightarrow\mathrm{0} \\ $$$${lim}\left(...\right)={lim}_{{t}\rightarrow\mathrm{0}} \:\frac{{t}−{tant}\:\:}{\frac{{t}^{\mathrm{3}} }{\mathrm{125}}}\:=\mathrm{125}\:{lim}_{{t}\rightarrow\mathrm{0}^{\:} \:} \:\frac{{t}−{tant}}{{t}^{\mathrm{3}} } \\ $$$${but}\:{lim}_{{t}\rightarrow\mathrm{0}} \:\:\frac{{t}−{tant}}{{t}^{\mathrm{3}} }\:={lim}_{{t}\rightarrow\mathrm{0}} \:\frac{\mathrm{1}−\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}\right)}{\mathrm{3}{t}^{\mathrm{2}} } \\ $$$$={lim}_{{t}\rightarrow\mathrm{0}} \:\frac{−\mathrm{2}{tant}\left(\mathrm{1}+{tan}^{\mathrm{2}} {t}\right)}{\mathrm{6}{t}}=\frac{−\mathrm{1}}{\mathrm{3}}\:{lim}_{{t}\rightarrow\mathrm{0}} \:\frac{{tant}}{{t}}=\frac{−\mathrm{1}}{\mathrm{3}}×\mathrm{1}=\frac{−\mathrm{1}}{\mathrm{3}} \\ $$$$\Rightarrow{lim}_{{x}\rightarrow\mathrm{0}} \frac{\mathrm{5}{x}\:−{tan}\left(\mathrm{5}{x}\right)}{{x}^{\mathrm{3}} }=\:\frac{−\mathrm{125}}{\mathrm{3}}\:. \\ $$

Commented by abdo imad last updated on 25/Jan/18

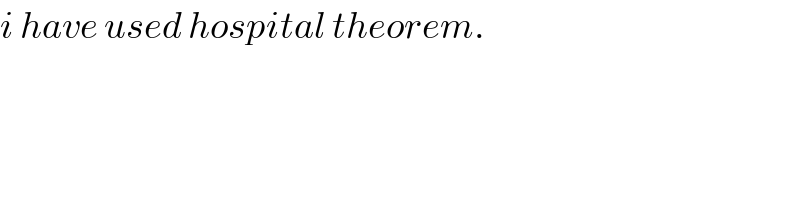
i have used hospital theorem.

$${i}\:{have}\:{used}\:{hospital}\:{theorem}. \\ $$

Commented by Cheyboy last updated on 25/Jan/18

Thank sir God bless you =

$${Thank}\:{sir}\:{God}\:{bless}\:{you} \\ $$$$= \\ $$$$ \\ $$$$ \\ $$

Answered by ajfour last updated on 24/Jan/18

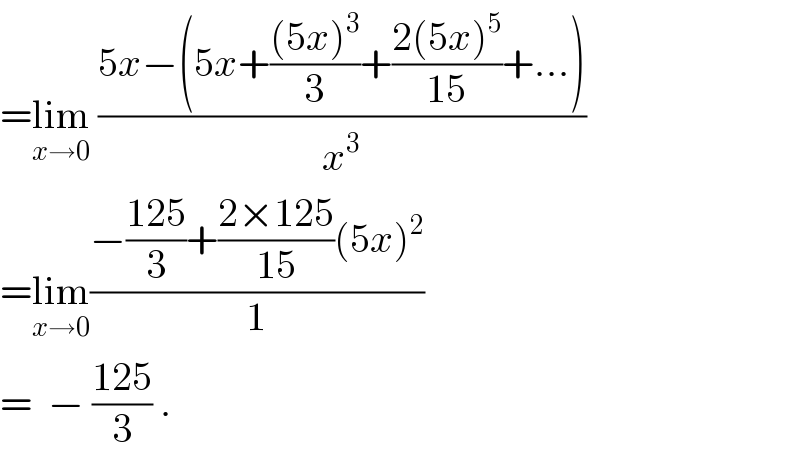
=lim_(x→0) ((5x−(5x+(((5x)^3 )/3)+((2(5x)^5 )/(15))+...))/x^3 ) =lim_(x→0) ((−((125)/3)+((2×125)/(15))(5x)^2 )/1) = − ((125)/3) .

$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{5}{x}−\left(\mathrm{5}{x}+\frac{\left(\mathrm{5}{x}\right)^{\mathrm{3}} }{\mathrm{3}}+\frac{\mathrm{2}\left(\mathrm{5}{x}\right)^{\mathrm{5}} }{\mathrm{15}}+...\right)}{{x}^{\mathrm{3}} } \\ $$$$=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{−\frac{\mathrm{125}}{\mathrm{3}}+\frac{\mathrm{2}×\mathrm{125}}{\mathrm{15}}\left(\mathrm{5}{x}\right)^{\mathrm{2}} }{\mathrm{1}}\: \\ $$$$=\:\:−\:\frac{\mathrm{125}}{\mathrm{3}}\:. \\ $$

Commented by Cheyboy last updated on 24/Jan/18

ooh sir then i miss the ans it was part of our test 2day but i got ((−25)/2) ok thank for the answer

$${ooh}\:{sir}\:{then}\:{i}\:{miss}\:{the}\:{ans} \\ $$$${it}\:{was}\:{part}\:{of}\:{our}\:{test}\:\mathrm{2}{day}\: \\ $$$${but}\:{i}\:{got}\:\frac{−\mathrm{25}}{\mathrm{2}}\:{ok}\:{thank}\:{for}\:{the}\:{answer} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com