Question and Answers Forum

All Questions      Topic List

Mechanics Questions

Previous in All Question      Next in All Question      

Previous in Mechanics      Next in Mechanics      

Question Number 28349 by ajfour last updated on 24/Jan/18

Commented by ajfour last updated on 24/Jan/18

$$Q.28339\left(answer\right)$$

Answered by ajfour last updated on 24/Jan/18

$$\mathit{\omega }=\frac{\mathit{d}\mathit{\theta }}{\mathit{d}\mathit{t}},$$$${\mathit{y}}_1=\mathit{a}\tan \mathit{\theta },{\mathit{y}}_2=\mathit{a}\sec \mathit{\theta }-\mathit{a}$$$$\frac{{dy}_1}{dt}=\omega a\sec {}^2\theta ,\frac{{dy}_2}{dt}=\omega a\sec \theta \tan \theta$$$${\mathit{m}\mathit{g}\mathit{y}}_1-{\mathit{m}\mathit{g}\mathit{y}}_2=\frac{1}{2}{\mathit{m}}_1{\left(\frac{{\mathit{d}\mathit{y}}_1}{\mathit{d}\mathit{t}}\right)}^2+$$$$\frac{1}{2}{\mathit{m}}_2{\left(\frac{{\mathit{d}\mathit{y}}_2}{\mathit{d}\mathit{t}}\right)}^2$$$$\Rightarrow 2m_{1}ga\tan \theta -2m_{2}ga(\sec \theta -1)$$$$=m_1{\omega }^2{a}^2\sec {}^4\theta +m_2{\omega }^2{a}^2\sec {}^2\theta \tan {}^2\theta$$$$or$$$$2ga(m_1\tan \theta -m_2\sec \theta +m_2)=$$$${\omega }^2{a}^2\sec {}^2\theta (m_1\sec {}^2\theta +m_2\tan {}^2\theta )$$$$\Rightarrow {\mathit{\omega }}^2=\frac{2\mathit{g}}{\mathit{a}}\frac{({\mathit{m}}_1\tan \mathit{\theta }-{\mathit{m}}_2\sec \mathit{\theta }+{\mathit{m}}_2)}{({\mathit{m}}_1\sec {}^2\mathit{\theta }+{\mathit{m}}_2\tan {}^2\mathit{\theta })}\cos {}^2\mathit{\theta }.$$$$\omega varieswith\theta ,$$$$at\theta =0$$$${\omega }^2=0\Rightarrow motionispossible$$$$T\to \mathrm{\infty }\Rightarrow tendencyofirreversible$$$$motionatthestart.$$

Commented by Tinkutara last updated on 24/Jan/18

This �� is answer in book. Please explain this.

Commented by Tinkutara last updated on 24/Jan/18

Commented by ajfour last updated on 24/Jan/18

$$forring:$$$$m_{1}g-T\sin \theta =m_1{a}_1$$$$forblock$$$$T-m_{2}g=m_2{a}_2$$$$forequilibrium{a}_1=a_2=0$$$$\Rightarrow m_{1}g-T\sin \theta =T-m_{2}g=0$$$$atequilibriumT=m_{2}g$$$$\sin \theta =\frac{m_1}{m_2}$$$$equilibriumcannotbepossible$$$$for{m}_1⩾m_2$$$$butinthecase{m}_1=\frac{m_2}{2}$$$$equilibriumat\mathit{\theta }={\sin }^{-1}\left(\frac{m_1}{m_2}\right)=\frac{\pi }{6}.$$

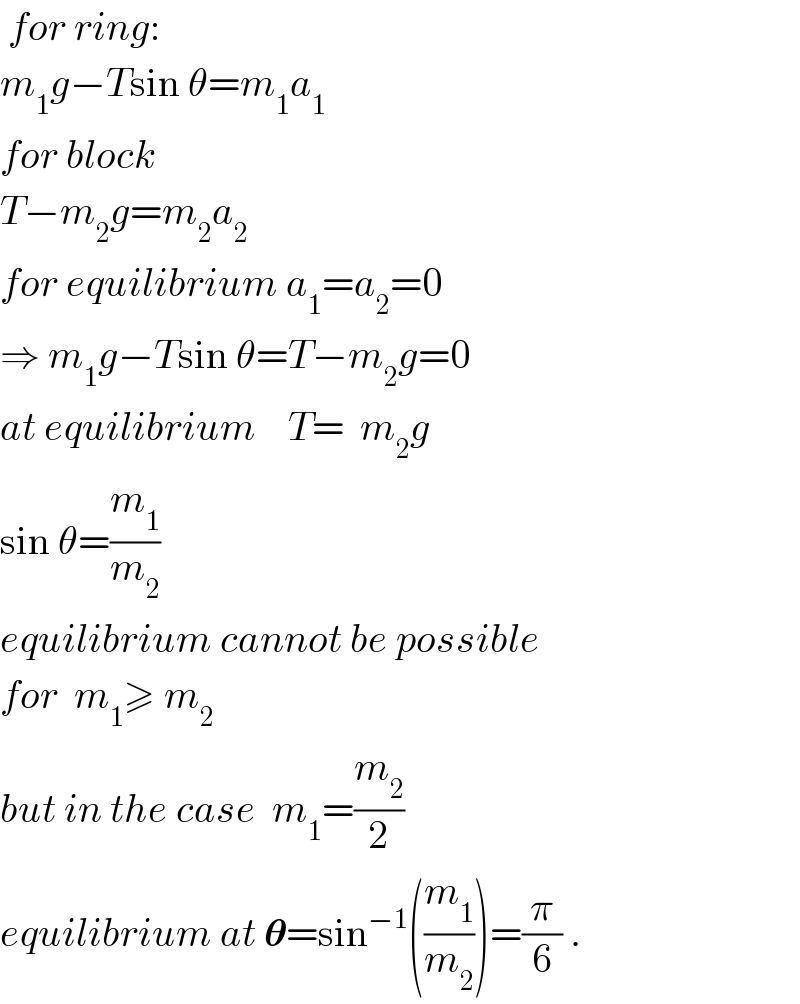
Commented by Tinkutara last updated on 25/Jan/18

Thank you very much Sir! I got the answer.

Terms of Service

Privacy Policy

Contact: info@tinkutara.com