Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 2845 by Rasheed Soomro last updated on 28/Nov/15

While you are in between the project I am trying to improve my digestiblity to digest the concept of ′analytical continuation′. First we make aformula to sum n terms of a powe series: ((x^n −1)/(x−1))=1+x+x^2 +...+x^n latter we change it for ∣x∣<1 and n→∞ [x^n →0] (1/(1−x))=1+x+x^2 +...+x^n and now insist to use this formula also for ∣x∣>1. WHY? [If ∣x∣ were greater than 1,then x^n ↛0 and ((x^n −1)/(x−1)) mustn′t be replaced by (1/(1−x)) ]

$$\mathcal{W}{hile}\:{you}\:{are}\:{in}\:{between}\:{the}\:{project} \\ $$$$\mathcal{I}\:{am}\:{trying}\:{to}\:{improve}\:{my}\:{digestiblity}\:{to} \\ $$$${digest}\:{the}\:{concept}\:{of}\:'{analytical}\:{continuation}'. \\ $$$$ \\ $$$${First}\:{we}\:{make}\:{aformula}\:{to}\:{sum}\:{n}\:{terms}\:{of}\:{a}\:{powe}\:{series}: \\ $$$$\frac{{x}^{{n}} −\mathrm{1}}{{x}−\mathrm{1}}=\mathrm{1}+{x}+{x}^{\mathrm{2}} +...+{x}^{{n}} \\ $$$${latter}\:{we}\:{change}\:{it}\:{for}\:\mid{x}\mid<\mathrm{1}\:{and}\:{n}\rightarrow\infty\:\left[{x}^{{n}} \rightarrow\mathrm{0}\right] \\ $$$$\:\frac{\mathrm{1}}{\mathrm{1}−{x}}=\mathrm{1}+{x}+{x}^{\mathrm{2}} +...+{x}^{{n}} \\ $$$${and}\:{now}\:{insist}\:{to}\:{use}\:{this}\:{formula}\:{also}\:{for}\:\mid{x}\mid>\mathrm{1}.\:\mathcal{WHY}? \\ $$$$\left[{If}\:\:\mid{x}\mid\:{were}\:{greater}\:{than}\:\mathrm{1},{then}\:{x}^{{n}} \nrightarrow\mathrm{0}\:{and}\:\:\frac{{x}^{{n}} −\mathrm{1}}{{x}−\mathrm{1}}\:{mustn}'{t}\right. \\ $$$$\left.{be}\:{replaced}\:{by}\:\frac{\mathrm{1}}{\mathrm{1}−{x}}\:\right] \\ $$$$ \\ $$

Answered by prakash jain last updated on 29/Nov/15

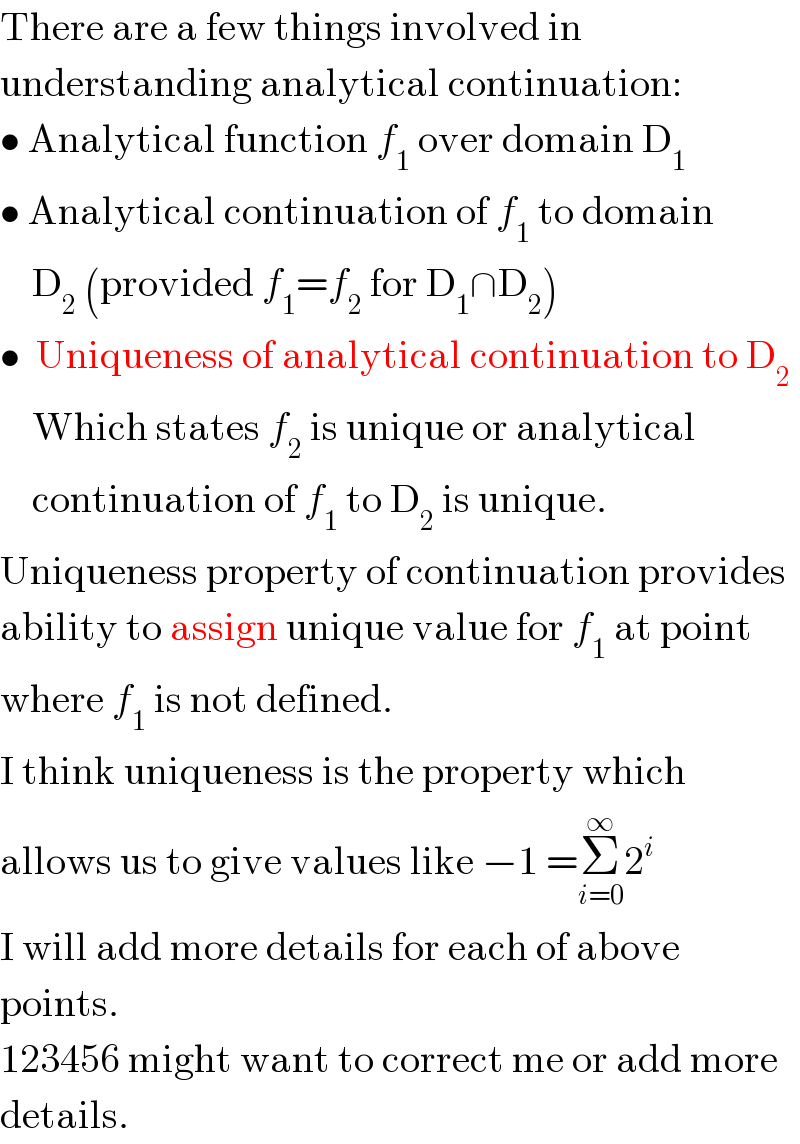
There are a few things involved in understanding analytical continuation: • Analytical function f_1 over domain D_1 • Analytical continuation of f_1 to domain D_2 (provided f_1 =f_2 for D_1 ∩D_2 ) • Uniqueness of analytical continuation to D_2 Which states f_2 is unique or analytical continuation of f_1 to D_2 is unique. Uniqueness property of continuation provides ability to assign unique value for f_1 at point where f_1 is not defined. I think uniqueness is the property which allows us to give values like −1 =Σ_(i=0) ^∞ 2^i I will add more details for each of above points. 123456 might want to correct me or add more details.

$$\mathrm{There}\:\mathrm{are}\:\mathrm{a}\:\mathrm{few}\:\mathrm{things}\:\mathrm{involved}\:\mathrm{in} \\ $$$$\mathrm{understanding}\:\mathrm{analytical}\:\mathrm{continuation}: \\ $$$$\bullet\:\mathrm{Analytical}\:\mathrm{function}\:{f}_{\mathrm{1}} \:\mathrm{over}\:\mathrm{domain}\:\mathrm{D}_{\mathrm{1}} \\ $$$$\bullet\:\mathrm{Analytical}\:\mathrm{continuation}\:\mathrm{of}\:{f}_{\mathrm{1}} \:\mathrm{to}\:\mathrm{domain} \\ $$$$\:\:\:\:\mathrm{D}_{\mathrm{2}} \:\left(\mathrm{provided}\:{f}_{\mathrm{1}} ={f}_{\mathrm{2}} \:\mathrm{for}\:\mathrm{D}_{\mathrm{1}} \cap\mathrm{D}_{\mathrm{2}} \right) \\ $$$$\bullet\:\:\mathrm{Uniqueness}\:\mathrm{of}\:\mathrm{analytical}\:\mathrm{continuation}\:\mathrm{to}\:\mathrm{D}_{\mathrm{2}} \\ $$$$\:\:\:\:\mathrm{Which}\:\mathrm{states}\:{f}_{\mathrm{2}} \:\mathrm{is}\:\mathrm{unique}\:\mathrm{or}\:\mathrm{analytical} \\ $$$$\:\:\:\:\mathrm{continuation}\:\mathrm{of}\:{f}_{\mathrm{1}} \:\mathrm{to}\:\mathrm{D}_{\mathrm{2}} \:\mathrm{is}\:\mathrm{unique}. \\ $$$$\mathrm{Uniqueness}\:\mathrm{property}\:\mathrm{of}\:\mathrm{continuation}\:\mathrm{provides} \\ $$$$\mathrm{ability}\:\mathrm{to}\:\mathrm{assign}\:\mathrm{unique}\:\mathrm{value}\:\mathrm{for}\:{f}_{\mathrm{1}} \:\mathrm{at}\:\mathrm{point} \\ $$$$\mathrm{where}\:{f}_{\mathrm{1}} \:\mathrm{is}\:\mathrm{not}\:\mathrm{defined}. \\ $$$$\mathrm{I}\:\mathrm{think}\:\mathrm{uniqueness}\:\mathrm{is}\:\mathrm{the}\:\mathrm{property}\:\mathrm{which} \\ $$$$\mathrm{allows}\:\mathrm{us}\:\mathrm{to}\:\mathrm{give}\:\mathrm{values}\:\mathrm{like}\:−\mathrm{1}\:=\underset{{i}=\mathrm{0}} {\overset{\infty} {\sum}}\mathrm{2}^{{i}} \\ $$$$\mathrm{I}\:\mathrm{will}\:\mathrm{add}\:\mathrm{more}\:\mathrm{details}\:\mathrm{for}\:\mathrm{each}\:\mathrm{of}\:\mathrm{above} \\ $$$$\mathrm{points}. \\ $$$$\mathrm{123456}\:\mathrm{might}\:\mathrm{want}\:\mathrm{to}\:\mathrm{correct}\:\mathrm{me}\:\mathrm{or}\:\mathrm{add}\:\mathrm{more} \\ $$$$\mathrm{details}. \\ $$

Commented by Rasheed Soomro last updated on 29/Nov/15

Why we should be ready for changing domain. Only for ′′Uniqueness property of continuation provides ability to assign unique value for f_1 at point where f_1 is not defined.′′ ? Why we accept unreasonable results only for this uniqueness?

$$\mathcal{W}{hy}\:{we}\:{should}\:{be}\:{ready}\:{for}\:{changing}\:{domain}. \\ $$$${Only}\:{for}\: \\ $$$$''\mathrm{Uniqueness}\:\mathrm{property}\:\mathrm{of}\:\mathrm{continuation}\:\mathrm{provides} \\ $$$$\mathrm{ability}\:\mathrm{to}\:\mathrm{assign}\:\mathrm{unique}\:\mathrm{value}\:\mathrm{for}\:{f}_{\mathrm{1}} \:\mathrm{at}\:\mathrm{point} \\ $$$$\mathrm{where}\:{f}_{\mathrm{1}} \:\mathrm{is}\:\mathrm{not}\:\mathrm{defined}.''\:\:? \\ $$$$\mathcal{W}{hy}\:{we}\:{accept}\:{unreasonable}\:{results}\:{only}\:{for} \\ $$$${this}\:\mathrm{uniqueness}? \\ $$

Commented by 123456 last updated on 29/Nov/15

i dont think this help, but suppose we have f:R→R and want to build f in form of a polinomy we can write (under certains conditions) f(x)=Σ_(n=0) ^(+∞) a_n (x−x_0 )^n a_n =((f^((n)) (x_0 ))/(n!)) this serie is a called taylor serie of f near point (x_0 =0) (we can extend it to C, but lets stay simple for now) this serie defines a power serie to any function (under certains condictions) like f(x)=sin x=x−(x^3 /(3!))+(x^5 /(5!))−∙∙∙ inside their comvergence radius, or more direct ∣x−x_0 ∣<R⇒f(x)=sin x=x−(x^3 /(3!))+∙∙∙ in case of sine, this radius is infinite however its not all function that have infinite radius, so in a gross way you can take the analytic continuations as a inverse way for this case

$$\mathrm{i}\:\mathrm{dont}\:\mathrm{think}\:\mathrm{this}\:\mathrm{help},\:\mathrm{but} \\ $$$$\mathrm{suppose}\:\mathrm{we}\:\mathrm{have} \\ $$$${f}:\mathbb{R}\rightarrow\mathbb{R} \\ $$$$\mathrm{and}\:\mathrm{want}\:\mathrm{to}\:\mathrm{build}\:{f}\:\mathrm{in}\:\mathrm{form}\:\mathrm{of}\:\mathrm{a}\:\mathrm{polinomy} \\ $$$$\mathrm{we}\:\mathrm{can}\:\mathrm{write}\:\left(\mathrm{under}\:\mathrm{certains}\:\mathrm{conditions}\right) \\ $$$${f}\left({x}\right)=\underset{{n}=\mathrm{0}} {\overset{+\infty} {\sum}}{a}_{{n}} \left({x}−{x}_{\mathrm{0}} \right)^{{n}} \\ $$$${a}_{{n}} =\frac{{f}^{\left({n}\right)} \left({x}_{\mathrm{0}} \right)}{{n}!} \\ $$$$\mathrm{this}\:\mathrm{serie}\:\mathrm{is}\:\mathrm{a}\:\mathrm{called}\:\mathrm{taylor}\:\mathrm{serie}\:\mathrm{of}\:{f} \\ $$$$\mathrm{near}\:\mathrm{point}\:\left({x}_{\mathrm{0}} =\mathrm{0}\right) \\ $$$$\left(\mathrm{we}\:\mathrm{can}\:\mathrm{extend}\:\mathrm{it}\:\mathrm{to}\:\mathbb{C},\:\mathrm{but}\:\mathrm{lets}\:\mathrm{stay}\right. \\ $$$$\left.\mathrm{simple}\:\mathrm{for}\:\mathrm{now}\right) \\ $$$$\mathrm{this}\:\mathrm{serie}\:\mathrm{defines}\:\mathrm{a}\:\mathrm{power}\:\mathrm{serie}\:\mathrm{to}\:\mathrm{any} \\ $$$$\mathrm{function}\:\left(\mathrm{under}\:\mathrm{certains}\:\mathrm{condictions}\right) \\ $$$$\mathrm{like} \\ $$$${f}\left({x}\right)=\mathrm{sin}\:{x}={x}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}+\frac{{x}^{\mathrm{5}} }{\mathrm{5}!}−\centerdot\centerdot\centerdot \\ $$$$\mathrm{inside}\:\boldsymbol{\mathrm{their}}\:\boldsymbol{\mathrm{comvergence}}\:\boldsymbol{\mathrm{radius}},\:\mathrm{or}\:\mathrm{more} \\ $$$$\mathrm{direct} \\ $$$$\mid{x}−{x}_{\mathrm{0}} \mid<\mathrm{R}\Rightarrow{f}\left({x}\right)=\mathrm{sin}\:{x}={x}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}+\centerdot\centerdot\centerdot \\ $$$$\mathrm{in}\:\mathrm{case}\:\mathrm{of}\:\mathrm{sine},\:\mathrm{this}\:\mathrm{radius}\:\mathrm{is}\:\mathrm{infinite} \\ $$$$\mathrm{however}\:\mathrm{its}\:\mathrm{not}\:\mathrm{all}\:\mathrm{function}\:\mathrm{that}\:\mathrm{have} \\ $$$$\mathrm{infinite}\:\mathrm{radius},\:\mathrm{so}\:\mathrm{in}\:\mathrm{a}\:\mathrm{gross}\:\mathrm{way}\:\mathrm{you} \\ $$$$\mathrm{can}\:\mathrm{take}\:\mathrm{the}\:\mathrm{analytic}\:\mathrm{continuations}\:\mathrm{as} \\ $$$$\mathrm{a}\:\mathrm{inverse}\:\mathrm{way}\:\mathrm{for}\:\mathrm{this}\:\mathrm{case} \\ $$

Commented by Rasheed Soomro last updated on 29/Nov/15

T h a n k S to BOTH of YOU !

$$\mathcal{T}\:\boldsymbol{{h}}\:\boldsymbol{{a}}\:\boldsymbol{{n}}\:\boldsymbol{{k}}\:\mathcal{S}\:\:{to}\:\mathcal{BOTH}\:{of}\:\mathcal{YOU}\:! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com