
Question and Answers Forum
Question Number 28808 by ajfour last updated on 30/Jan/18

Answered by ajfour last updated on 30/Jan/18
![taking A as origin, and ∠AED=α =tan^(−1) 2 eq. of AC y=x eq. of DE y=a−2x F lies on both lines so x_F =y_F =(a/3) (r_1 /(cot 22.5°+cot ((α/2)))) = (a/2) let cot 22.5° =(1/m_1 ) ((2m_1 )/(1−m_1 ^2 ))=1 ⇒ m_1 ^2 +2m_1 =1 m_1 =((−2+2(√2))/2) = (√2)−1 (1/m_1 ) = (√2)+1 let tan (α/2)=m tan α==((2m)/(1−m^2 )) = 2 ⇒ 2m^2 +2m−2=0 or m=((−2+2(√5))/4) = (((√5)−1)/2) cot (α/2)= (1/m) = (((√5)+1)/2) As r_1 (cot 22.5°+cot (𝛂/2))=(a/2) , r_1 =((a/2)/((√2)+1+(((√5)+1)/2))) =(a/(2(√2)+(√5)+3)) .... tan (45°−(α/2))=((1−((((√5)−1)/2)))/(1+(((√5)−1)/2))) =((3−(√5))/(1+(√5))) = cot (45°−(α/2))=((1+(√5))/(3−(√5))) =((3+5+2(√5))/4) = 2+((√5)/2) r_2 (cot 22.5°+cot (45°−(α/2))= a ⇒ r_2 =(a/((√2)+3+((√5)/2))) ..... cot (45°+(α/2))=tan (45°−(α/2)) =((3−(√5))/((√5)+1)) =((4(√5)−8)/4) =(√5)−2 r_3 [cot 22.5°+cot (45°+(α/2))]= a ⇒ r_3 =(a/((√2)+(√5)−1)) .......](Q28810.png)
Answered by mrW2 last updated on 30/Jan/18
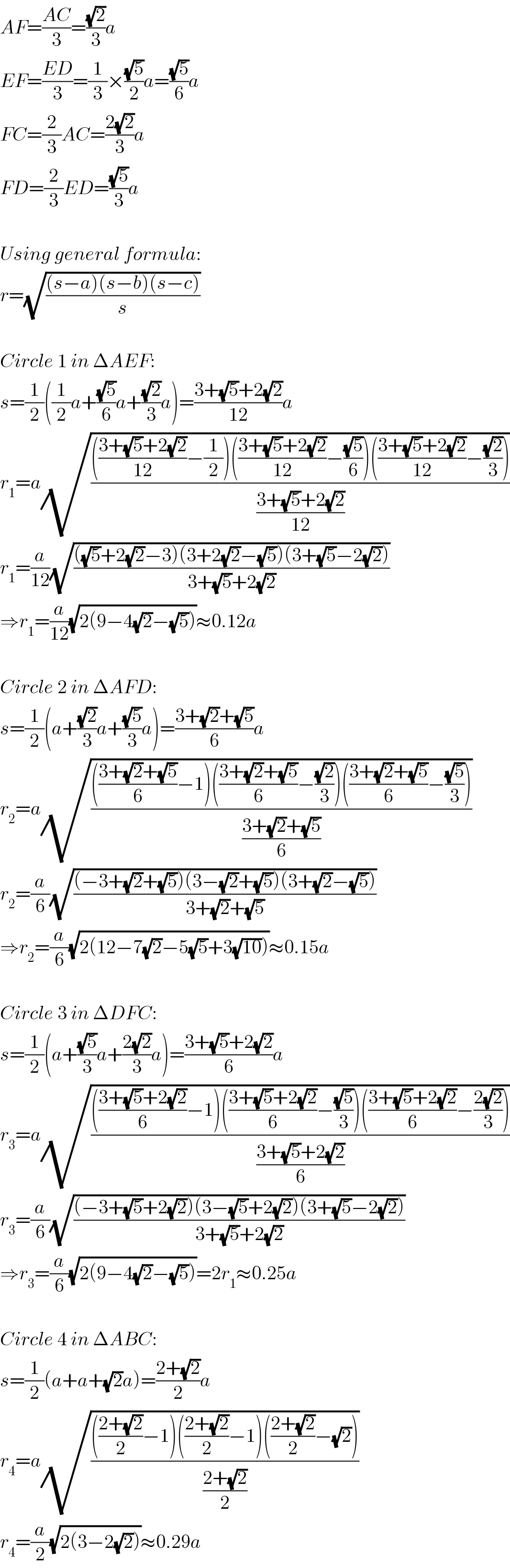
Commented by ajfour last updated on 30/Jan/18

| ||
Question and Answers Forum | ||
Question Number 28808 by ajfour last updated on 30/Jan/18 | ||
 | ||
Answered by ajfour last updated on 30/Jan/18 | ||
![taking A as origin, and ∠AED=α =tan^(−1) 2 eq. of AC y=x eq. of DE y=a−2x F lies on both lines so x_F =y_F =(a/3) (r_1 /(cot 22.5°+cot ((α/2)))) = (a/2) let cot 22.5° =(1/m_1 ) ((2m_1 )/(1−m_1 ^2 ))=1 ⇒ m_1 ^2 +2m_1 =1 m_1 =((−2+2(√2))/2) = (√2)−1 (1/m_1 ) = (√2)+1 let tan (α/2)=m tan α==((2m)/(1−m^2 )) = 2 ⇒ 2m^2 +2m−2=0 or m=((−2+2(√5))/4) = (((√5)−1)/2) cot (α/2)= (1/m) = (((√5)+1)/2) As r_1 (cot 22.5°+cot (𝛂/2))=(a/2) , r_1 =((a/2)/((√2)+1+(((√5)+1)/2))) =(a/(2(√2)+(√5)+3)) .... tan (45°−(α/2))=((1−((((√5)−1)/2)))/(1+(((√5)−1)/2))) =((3−(√5))/(1+(√5))) = cot (45°−(α/2))=((1+(√5))/(3−(√5))) =((3+5+2(√5))/4) = 2+((√5)/2) r_2 (cot 22.5°+cot (45°−(α/2))= a ⇒ r_2 =(a/((√2)+3+((√5)/2))) ..... cot (45°+(α/2))=tan (45°−(α/2)) =((3−(√5))/((√5)+1)) =((4(√5)−8)/4) =(√5)−2 r_3 [cot 22.5°+cot (45°+(α/2))]= a ⇒ r_3 =(a/((√2)+(√5)−1)) .......](Q28810.png) | ||
| ||
Answered by mrW2 last updated on 30/Jan/18 | ||
 | ||
| ||
Commented by ajfour last updated on 30/Jan/18 | ||
 | ||