Question and Answers Forum

All Questions      Topic List

Mechanics Questions

Previous in All Question      Next in All Question      

Previous in Mechanics      Next in Mechanics      

Question Number 29322 by ajfour last updated on 07/Feb/18

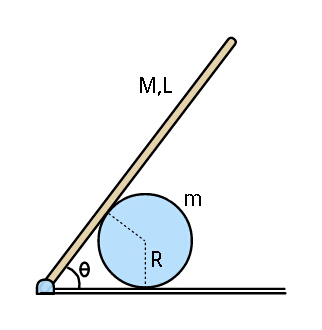
Commented by ajfour last updated on 07/Feb/18

$$Findaccelerationofsphereas$$$$afunctionofangle\theta .$$$$Systemisreleasedat\theta ={\theta }_0(<90°);$$$$\left(Allsurfacesarefrictionless\right).$$

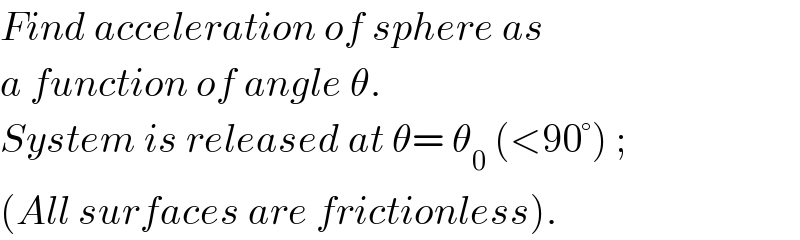
Commented by ajfour last updated on 07/Feb/18

$$Pleasesolveorcheckmysolution$$$$mrWSir.$$

Commented by ajfour last updated on 07/Feb/18

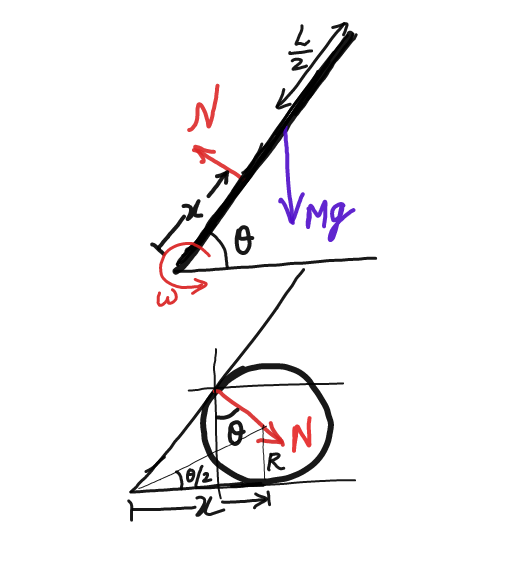
Commented by ajfour last updated on 07/Feb/18

$$x=R\cot \frac{\theta }{2}$$$$v=-\frac{\omega R}{2}\mathrm{cosec}{}^2\frac{\theta }{2}$$$$\Rightarrow {\mathit{v}}^2=\frac{{\mathit{\omega }}^2{\mathit{R}}^2}{4}\mathrm{cosec}{}^4\frac{\theta }{2}....\left(i\right)$$$$a=\frac{dv}{dt}=-\frac{\alpha R}{2}\mathrm{cosec}{}^2\frac{\theta }{2}$$$$+\frac{{\omega }^{2}R}{2}\mathrm{cosec}{}^2\frac{\theta }{2}\cot \frac{\theta }{2}...\left(ii\right)$$$$N\sin \theta =ma....\left(iii\right)$$$$NR\cot \frac{\theta }{2}-\frac{MgL\cos \theta }{2}=\left(\frac{{ML}^2}{3}\right)\alpha$$$$$$$$\Rightarrow \alpha =\frac{3}{{ML}^2}(\frac{maR\cot \frac{\theta }{2}}{\sin \theta }-\frac{MgL\cos \theta }{2})$$$$.....\left(iv\right)$$$$\frac{MgL}{2}(\sin {\theta }_0-\sin \theta )=\frac{1}{2}{mv}^2+\frac{1}{2}\left(\frac{{ML}^2}{3}\right){\omega }^2$$$$using\left(i\right):$$$$\frac{MgL}{2}(\sin {\theta }_0-\sin \theta )=\frac{m{\omega }^2{R}^2}{8}\mathrm{cosec}{}^4\frac{\theta }{2}$$$$+\frac{{ML}^2{\omega }^2}{6}$$$${\omega }^2=\frac{\frac{MgL}{2}(\sin {\theta }_0-\sin \theta )}{\frac{{ML}^2}{6}+\frac{{mR}^2}{8}\mathrm{cosec}{}^4\frac{\theta }{2}}....\left(A\right)$$$$Usingeq.\left(A\right),\left(iii\right),\left(iv\right)ineq.\left(\mathit{i}\mathit{i}\right)$$$$a=\frac{R}{2}\mathrm{cosec}{}^2\frac{\theta }{2}\left(\frac{3}{{ML}^2}\right)(\frac{MgL\cos \theta }{2}-\frac{maR\cot \frac{\theta }{2}}{\sin \theta })$$$$+\frac{R}{2}\mathrm{cosec}{}^2\frac{\theta }{2}\cot \frac{\theta }{2}\left[\frac{\frac{MgL}{2}(\sin {\theta }_0-\sin \theta )}{\frac{{ML}^2}{6}+\frac{{mR}^2}{8}\mathrm{cosec}{}^4\frac{\theta }{2}}\right]$$$$$$$$a[1+\frac{3{mR}^2\mathrm{cosec}{}^2\frac{\theta }{2}\cot \frac{\theta }{2}}{2{ML}^2\sin \theta }]=$$$$\frac{R}{2}\mathrm{cosec}{}^2\frac{\theta }{2}[\frac{3g\cos \theta }{2L}+\frac{\frac{MgL}{2}(\sin {\theta }_0-\sin \theta )\cot \frac{\theta }{2}}{\frac{{ML}^2}{6}+\frac{{mR}^2}{8}\mathrm{cosec}{}^4\frac{\theta }{2}}].$$

Commented by mrW2 last updated on 07/Feb/18

$$solutioniscorrectsir!$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com