
Question and Answers Forum
Question Number 29849 by abdo imad last updated on 12/Feb/18
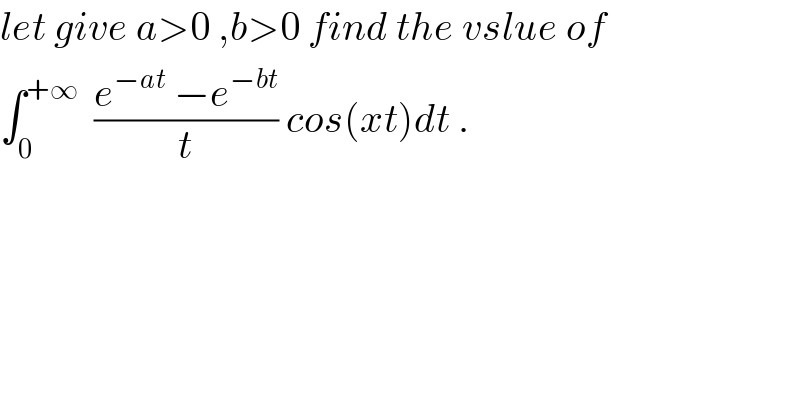
Commented byabdo imad last updated on 19/Feb/18
![let put F(x)= ∫_0 ^∞ ((e^(−at) −e^(−bt) )/t)cos(xt)dt after verifying tbat F is derivable we have F^′ (x)= ∫_0 ^∞ (∂/∂x)( ((e^(−at) −e^(−bt) )/t)cos(xt))dt =−∫_0 ^∞ (e^(−at) − e^(−bt) )sin(xt)dt=Im(∫_0 ^∞ (e^(−bt) −e^(−at) )e^(ixt) dt) =Im( ∫_0 ^∞ e^((−b+ix)t) −e^((−a+ix)t) )dt but ∫_0 ^∞ e^((−b+ix)t) dt= (1/(−b+ix)) [ e^((−b+ix)t) ]_(t=0) ^∞ = ((−1)/(−b +ix)) = (1/(b−ix)) also we have ∫_0 ^∞ e^((−a+ix)t) dt= (1/(a−ix)) ⇒ (dF/dx)(x)= (1/(b−ix)) −(1/(a−ix))= ((b+ix)/(b^2 +x^2 )) − ((a+ix)/(a^2 +x^2 )) =(b/(b^2 +x^2 )) −(a/(a^2 +x^2 )) +ix( (1/(b^2 +x^2 )) − (1/(a^2 +x^2 )))⇒ (dF/dx)(x)=x ((a^2 +x^2 −b^2 −x^2 )/((a^2 +x^2 )(b^2 +x^2 )))=(((a^2 −b^2 )x)/((a^2 +x^2 )(b^2 +x^2 )))⇒ F(x)= ∫ (x/(b^2 +x^2 ))dx −∫ (x/(a^2 +x^2 ))dx +λ =(1/2)ln(b^2 +x^2 ) −(1/2)ln(a^2 +x^2 ) +λ F(x)=(1/2)ln(((x^2 +b^2 )/(x^2 +a^2 ))) +λ λ=lim_(x→0) (F(x)−(1/2)ln( ((x^2 +b^2 )/(x^2 +a^2 ))))=F(o)−ln((b/a)) λ=∫_0 ^∞ ((e^(−at) −e^(−bt) )/t)dt −ln((b/a)) let put I(ξ)=∫_ξ ^(+∞) ((e^(−at) −e^(−bt) )/t)dt=∫_ξ ^∞ (e^(−at) /t)dt −∫_ξ ^∞ (e^(−bt) /t)dt ch.at=u give ∫_ξ ^∞ (e^(−at) /t)dt= ∫_(aξ) ^(+∞) (e^(−u) /(u/a)) (du/a)= ∫_(aξ) ^(+∞) (e^(−u) /u)du⇒ I(ξ)= ∫_(aξ) ^(+∞) (e^(−u) /u)du −∫_(bξ) ^(+∞) (e^(−u) /u)du=∫_(aξ) ^(bξ) (e^(−u) /u) du but ∃ c_ ∈]aξ,bξ[ / I(ξ)= e^(−ξ) ∫_(aξ) ^(bξ) (du/u)=e^(−ξ) ln((b/a)) ⇒ ∫_0 ^∞ ((e^(−at) −e^(−bt) )/t)dt=lim_(ξ→0) I(ξ)=ln((b/a)) ⇒ λ=0 and F(x)=(1/2)ln(((x^2 +b^2 )/(x^2 +a^2 ))) .](Q30242.png)
| ||
Question and Answers Forum | ||
Question Number 29849 by abdo imad last updated on 12/Feb/18 | ||
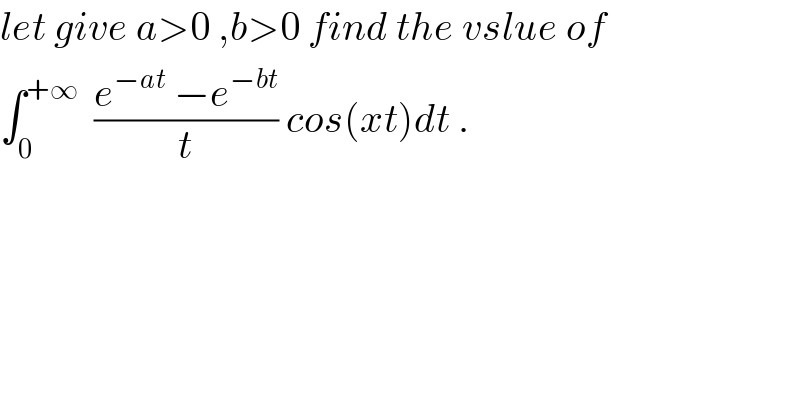 | ||
Commented byabdo imad last updated on 19/Feb/18 | ||
![let put F(x)= ∫_0 ^∞ ((e^(−at) −e^(−bt) )/t)cos(xt)dt after verifying tbat F is derivable we have F^′ (x)= ∫_0 ^∞ (∂/∂x)( ((e^(−at) −e^(−bt) )/t)cos(xt))dt =−∫_0 ^∞ (e^(−at) − e^(−bt) )sin(xt)dt=Im(∫_0 ^∞ (e^(−bt) −e^(−at) )e^(ixt) dt) =Im( ∫_0 ^∞ e^((−b+ix)t) −e^((−a+ix)t) )dt but ∫_0 ^∞ e^((−b+ix)t) dt= (1/(−b+ix)) [ e^((−b+ix)t) ]_(t=0) ^∞ = ((−1)/(−b +ix)) = (1/(b−ix)) also we have ∫_0 ^∞ e^((−a+ix)t) dt= (1/(a−ix)) ⇒ (dF/dx)(x)= (1/(b−ix)) −(1/(a−ix))= ((b+ix)/(b^2 +x^2 )) − ((a+ix)/(a^2 +x^2 )) =(b/(b^2 +x^2 )) −(a/(a^2 +x^2 )) +ix( (1/(b^2 +x^2 )) − (1/(a^2 +x^2 )))⇒ (dF/dx)(x)=x ((a^2 +x^2 −b^2 −x^2 )/((a^2 +x^2 )(b^2 +x^2 )))=(((a^2 −b^2 )x)/((a^2 +x^2 )(b^2 +x^2 )))⇒ F(x)= ∫ (x/(b^2 +x^2 ))dx −∫ (x/(a^2 +x^2 ))dx +λ =(1/2)ln(b^2 +x^2 ) −(1/2)ln(a^2 +x^2 ) +λ F(x)=(1/2)ln(((x^2 +b^2 )/(x^2 +a^2 ))) +λ λ=lim_(x→0) (F(x)−(1/2)ln( ((x^2 +b^2 )/(x^2 +a^2 ))))=F(o)−ln((b/a)) λ=∫_0 ^∞ ((e^(−at) −e^(−bt) )/t)dt −ln((b/a)) let put I(ξ)=∫_ξ ^(+∞) ((e^(−at) −e^(−bt) )/t)dt=∫_ξ ^∞ (e^(−at) /t)dt −∫_ξ ^∞ (e^(−bt) /t)dt ch.at=u give ∫_ξ ^∞ (e^(−at) /t)dt= ∫_(aξ) ^(+∞) (e^(−u) /(u/a)) (du/a)= ∫_(aξ) ^(+∞) (e^(−u) /u)du⇒ I(ξ)= ∫_(aξ) ^(+∞) (e^(−u) /u)du −∫_(bξ) ^(+∞) (e^(−u) /u)du=∫_(aξ) ^(bξ) (e^(−u) /u) du but ∃ c_ ∈]aξ,bξ[ / I(ξ)= e^(−ξ) ∫_(aξ) ^(bξ) (du/u)=e^(−ξ) ln((b/a)) ⇒ ∫_0 ^∞ ((e^(−at) −e^(−bt) )/t)dt=lim_(ξ→0) I(ξ)=ln((b/a)) ⇒ λ=0 and F(x)=(1/2)ln(((x^2 +b^2 )/(x^2 +a^2 ))) .](Q30242.png) | ||