
Question Number 31073 by abdo imad last updated on 02/Mar/18
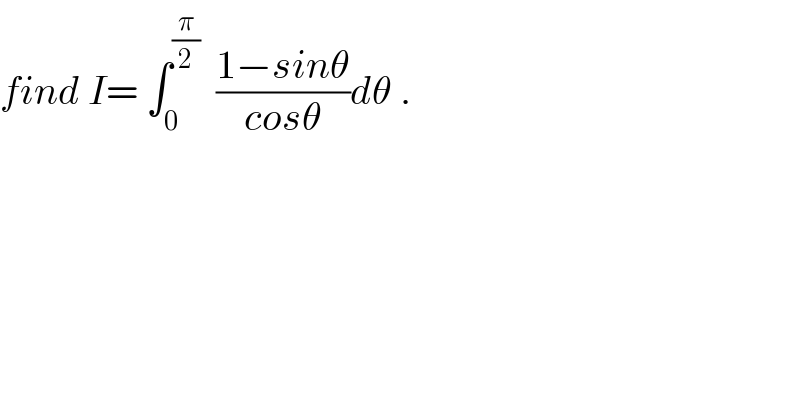
$${find}\:{I}=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\frac{\mathrm{1}−{sin}\theta}{{cos}\theta}{d}\theta\:. \\ $$
Commented by prof Abdo imad last updated on 03/Mar/18
![the ch.tan((θ/2))=x give I=∫_0 ^1 ((1 −((2x)/(1+x^2 )))/((1−x^2 )/(1+x^2 ))) ((2dx)/(1+x^2 )) = 2∫_0 ^1 (((x−1)^2 )/((1−x^2 )(1+x^2 )))dx =2 ∫_0 ^1 ((1−x)/((1+x)(1+x^2 )))dx let decompose the frsction F(x)= ((1−x)/((1+x)(1+x^2 ))) = (a/(1+x)) +((bx +c)/(1+x^2 )) a=lim_(x→−1) (x+1)F(x)= (2/2)=1 lim_(x→+∞) xF(x)= 0=a+b ⇒b=−a=−1 ⇒ F(x)= (1/(1+x)) +((−x+c)/(1+x^2 )) F(0)=1= 1+c⇒c=0 soF(x)= (1/(1+x)) −(x/(1+x^2 ))⇒ I= 2∫_0 ^1 (dx/(1+x))dx +∫_0 ^1 ((2x)/(1+x^2 ))dxp = 2[ln∣1+x∣]_0 ^(1 ) −[ln(1+x^2 )]_0 ^1 = 2ln(2) −ln(2)⇒ I=ln(2) .](Q31140.png)
$${the}\:{ch}.{tan}\left(\frac{\theta}{\mathrm{2}}\right)={x}\:{give} \\ $$$${I}=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{\mathrm{1}\:−\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }}{\frac{\mathrm{1}−{x}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} }}\:\frac{\mathrm{2}{dx}}{\mathrm{1}+{x}^{\mathrm{2}} }\:=\:\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\left({x}−\mathrm{1}\right)^{\mathrm{2}} }{\left(\mathrm{1}−{x}^{\mathrm{2}} \right)\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{dx} \\ $$$$=\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{1}−{x}}{\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}{dx}\:{let}\:{decompose}\:{the}\:{frsction} \\ $$$${F}\left({x}\right)=\:\frac{\mathrm{1}−{x}}{\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}\:=\:\frac{{a}}{\mathrm{1}+{x}}\:+\frac{{bx}\:+{c}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$${a}={lim}_{{x}\rightarrow−\mathrm{1}} \left({x}+\mathrm{1}\right){F}\left({x}\right)=\:\frac{\mathrm{2}}{\mathrm{2}}=\mathrm{1} \\ $$$${lim}_{{x}\rightarrow+\infty} {xF}\left({x}\right)=\:\mathrm{0}={a}+{b}\:\Rightarrow{b}=−{a}=−\mathrm{1}\:\Rightarrow \\ $$$${F}\left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{1}+{x}}\:+\frac{−{x}+{c}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$${F}\left(\mathrm{0}\right)=\mathrm{1}=\:\mathrm{1}+{c}\Rightarrow{c}=\mathrm{0}\:{soF}\left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{1}+{x}}\:−\frac{{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\Rightarrow \\ $$$${I}=\:\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{dx}}{\mathrm{1}+{x}}{dx}\:\:+\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }{dxp} \\ $$$$=\:\mathrm{2}\left[{ln}\mid\mathrm{1}+{x}\mid\right]_{\mathrm{0}} ^{\mathrm{1}\:\:} \:\:−\left[{ln}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\right]_{\mathrm{0}} ^{\mathrm{1}} \:=\:\mathrm{2}{ln}\left(\mathrm{2}\right)\:−{ln}\left(\mathrm{2}\right)\Rightarrow \\ $$$${I}={ln}\left(\mathrm{2}\right)\:. \\ $$
Answered by Joel578 last updated on 02/Mar/18
![I = lim_(t→(π/2)) (∫_0 ^t sec x − tan x dx) = lim_(t→(π/2)) [ln (sec x + tan x)cos x]_0 ^t = lim_(t→(π/2)) ln ((sec t + tan t)cos t) − ln (1 + 0) = lim_(t→(π/2)) ln ((sec t + tan t)cos t) = lim_(t→(π/2)) ln (1 + tan t cos t) = ln (1 + lim_(t→(π/2)) tan t cos t) = ln (1 + lim_(t→(π/2)) ((cos t)/(cot t))) = ln (1 + lim_(t→(π/2)) ((−sin t)/(−cosec^2 t))) = ln 2](Q31134.png)
$${I}\:=\:\underset{{t}\rightarrow\frac{\pi}{\mathrm{2}}} {\mathrm{lim}}\:\left(\int_{\mathrm{0}} ^{{t}} \mathrm{sec}\:{x}\:−\:\mathrm{tan}\:{x}\:{dx}\right) \\ $$$$\:\:\:=\:\underset{{t}\rightarrow\frac{\pi}{\mathrm{2}}} {\mathrm{lim}}\:\left[\mathrm{ln}\:\left(\mathrm{sec}\:{x}\:+\:\mathrm{tan}\:{x}\right)\mathrm{cos}\:{x}\right]_{\mathrm{0}} ^{{t}} \\ $$$$\:\:\:=\:\underset{{t}\rightarrow\frac{\pi}{\mathrm{2}}} {\mathrm{lim}}\:\mathrm{ln}\:\left(\left(\mathrm{sec}\:{t}\:+\:\mathrm{tan}\:{t}\right)\mathrm{cos}\:{t}\right)\:−\:\mathrm{ln}\:\left(\mathrm{1}\:+\:\mathrm{0}\right) \\ $$$$\:\:\:=\:\underset{{t}\rightarrow\frac{\pi}{\mathrm{2}}} {\mathrm{lim}}\:\mathrm{ln}\:\left(\left(\mathrm{sec}\:{t}\:+\:\mathrm{tan}\:{t}\right)\mathrm{cos}\:{t}\right) \\ $$$$\:\:\:=\:\underset{{t}\rightarrow\frac{\pi}{\mathrm{2}}} {\mathrm{lim}}\:\mathrm{ln}\:\left(\mathrm{1}\:+\:\mathrm{tan}\:{t}\:\mathrm{cos}\:{t}\right)\:=\:\mathrm{ln}\:\left(\mathrm{1}\:+\:\underset{{t}\rightarrow\frac{\pi}{\mathrm{2}}} {\mathrm{lim}}\:\mathrm{tan}\:{t}\:\mathrm{cos}\:{t}\right) \\ $$$$\:\:\:=\:\mathrm{ln}\:\left(\mathrm{1}\:+\:\underset{{t}\rightarrow\frac{\pi}{\mathrm{2}}} {\mathrm{lim}}\:\frac{\mathrm{cos}\:{t}}{\mathrm{cot}\:{t}}\right) \\ $$$$\:\:\:=\:\mathrm{ln}\:\left(\mathrm{1}\:+\:\underset{{t}\rightarrow\frac{\pi}{\mathrm{2}}} {\mathrm{lim}}\:\frac{−\mathrm{sin}\:{t}}{−\mathrm{cosec}^{\mathrm{2}} \:{t}}\right)\:=\:\mathrm{ln}\:\mathrm{2} \\ $$
