
Question and Answers Forum
Question Number 32040 by abdo imad last updated on 18/Mar/18
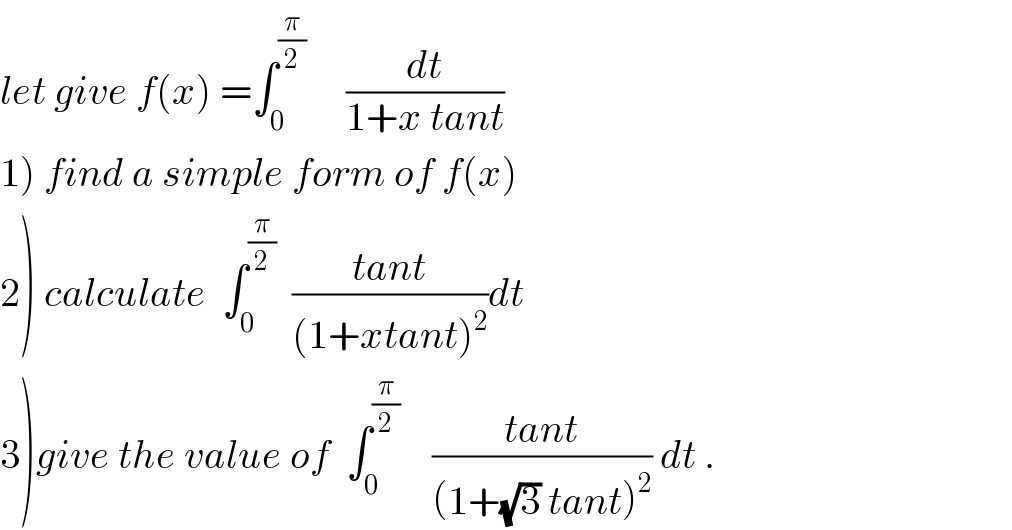
Commented by abdo imad last updated on 21/Mar/18
![ch.tant =u give f(x)=∫_0 ^∞ (1/(1+xu^2 )) (du/(1+u^2 )) =∫_0 ^∞ (du/((1+u^2 )(1+xu^2 ))) let decompose F(u)= (1/((1+u^2 )(1+xu^2 ))) = ((au +b)/(1+u^2 )) + ((cu +d)/(1+xu^2 )) F(−u)=F(u)⇒a=c=0⇒F(u)= (b/(1+u^2 )) +(d/(1+xu^2 )) F(0)=1= b+d F(1)= (1/(2(1+x))) = (b/2) + (d/(1+x))= (b/2) +((2d)/(2(1+x))) ⇒ (1/(1+x)) = b +((2d)/(1+x)) ⇒1 =(1+x)b +2d ⇒ 1 =(1+x)b +2(1−b)⇒1= 2 +(x−1)b ⇒b=(1/(1−x)) d=1−b =1−(1/(1−x)) = ((−x)/(1−x)) ⇒ F(u) = (1/((1−x)(1+u^2 ))) −(x/((1−x)(1+xu^2 ))) f(x)= (1/(1−x))∫_0 ^∞ (du/(1+u^2 )) −(x/(1−x))∫_0 ^∞ (du/(1+xu^2 )) but we have ∫_0 ^∞ (du/(1+u^2 ))= [arctanu]_0 ^∞ =(π/2) and ch. (√x) u =t (we suppose x>0) ∫_0 ^∞ (du/(1+xu^2 )) = ∫_0 ^∞ (1/(1+t^2 )) (dt/(√x)) = (π/(2(√x))) ⇒ f(x)= (π/(2(1−x))) − ((πx)/(2(√x)(1−x))) =(π/(2(1−x)))(1− (√x)) =(π/2) ((1−(√x))/((1+(√x))(1−(√x)))) = (π/(2(1+(√x)))) . 2)we have f(x)=∫_0 ^(π/2) (dt/(1+xtant)) ⇒ f^′ (x) =−∫_0 ^(π/2) ((tant)/((1+xtant)^2 ))dt ⇒ ∫_0 ^(π/2) ((tant)/((1+xtant)^2 )) dt =−f^′ (x) but f^′ (x) =−(π/2) (((1+(√(x )))^′ )/((1+(√x))^2 )) =−(π/2) (1/(2(√x)(1+(√x))^2 )) ⇒ ∫_0 ^(π/2) ((tant)/((1+xtant)^2 )) dt = (π/(4 (√x)(1+(√x))^2 )) 3) let take x=(√3) weget ∫_0 ^(π/2) ((tant)/((1+(√3)tant)^2 )) dt = (π/(4^4 (√3)(1+^4 (√3))^2 )) .](Q32198.png)
Commented by abdo imad last updated on 21/Mar/18

| ||
Question and Answers Forum | ||
Question Number 32040 by abdo imad last updated on 18/Mar/18 | ||
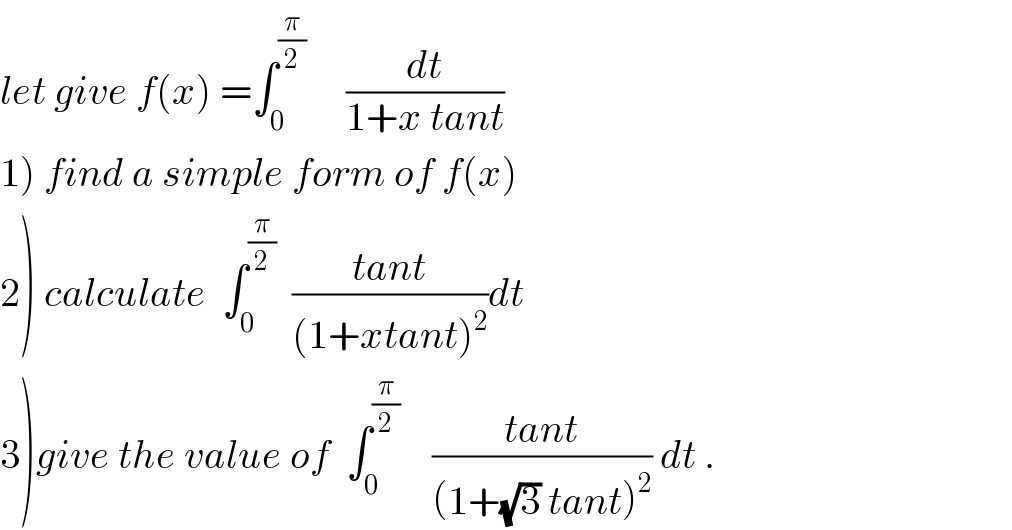 | ||
Commented by abdo imad last updated on 21/Mar/18 | ||
![ch.tant =u give f(x)=∫_0 ^∞ (1/(1+xu^2 )) (du/(1+u^2 )) =∫_0 ^∞ (du/((1+u^2 )(1+xu^2 ))) let decompose F(u)= (1/((1+u^2 )(1+xu^2 ))) = ((au +b)/(1+u^2 )) + ((cu +d)/(1+xu^2 )) F(−u)=F(u)⇒a=c=0⇒F(u)= (b/(1+u^2 )) +(d/(1+xu^2 )) F(0)=1= b+d F(1)= (1/(2(1+x))) = (b/2) + (d/(1+x))= (b/2) +((2d)/(2(1+x))) ⇒ (1/(1+x)) = b +((2d)/(1+x)) ⇒1 =(1+x)b +2d ⇒ 1 =(1+x)b +2(1−b)⇒1= 2 +(x−1)b ⇒b=(1/(1−x)) d=1−b =1−(1/(1−x)) = ((−x)/(1−x)) ⇒ F(u) = (1/((1−x)(1+u^2 ))) −(x/((1−x)(1+xu^2 ))) f(x)= (1/(1−x))∫_0 ^∞ (du/(1+u^2 )) −(x/(1−x))∫_0 ^∞ (du/(1+xu^2 )) but we have ∫_0 ^∞ (du/(1+u^2 ))= [arctanu]_0 ^∞ =(π/2) and ch. (√x) u =t (we suppose x>0) ∫_0 ^∞ (du/(1+xu^2 )) = ∫_0 ^∞ (1/(1+t^2 )) (dt/(√x)) = (π/(2(√x))) ⇒ f(x)= (π/(2(1−x))) − ((πx)/(2(√x)(1−x))) =(π/(2(1−x)))(1− (√x)) =(π/2) ((1−(√x))/((1+(√x))(1−(√x)))) = (π/(2(1+(√x)))) . 2)we have f(x)=∫_0 ^(π/2) (dt/(1+xtant)) ⇒ f^′ (x) =−∫_0 ^(π/2) ((tant)/((1+xtant)^2 ))dt ⇒ ∫_0 ^(π/2) ((tant)/((1+xtant)^2 )) dt =−f^′ (x) but f^′ (x) =−(π/2) (((1+(√(x )))^′ )/((1+(√x))^2 )) =−(π/2) (1/(2(√x)(1+(√x))^2 )) ⇒ ∫_0 ^(π/2) ((tant)/((1+xtant)^2 )) dt = (π/(4 (√x)(1+(√x))^2 )) 3) let take x=(√3) weget ∫_0 ^(π/2) ((tant)/((1+(√3)tant)^2 )) dt = (π/(4^4 (√3)(1+^4 (√3))^2 )) .](Q32198.png) | ||
Commented by abdo imad last updated on 21/Mar/18 | ||
 | ||