Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 32341 by abdo imad last updated on 23/Mar/18

let give λ from R and λ^2 ≠1 and I_n (λ) = ∫_0 ^π ((cos(nt))/(1−2λcost +λ^2 ))dt .calculate I_n (λ).

$${let}\:{give}\:\lambda\:{from}\:{R}\:{and}\:\lambda^{\mathrm{2}} \neq\mathrm{1}\:{and} \\ $$$${I}_{{n}} \left(\lambda\right)\:=\:\int_{\mathrm{0}} ^{\pi} \:\:\:\frac{{cos}\left({nt}\right)}{\mathrm{1}−\mathrm{2}\lambda{cost}\:+\lambda^{\mathrm{2}} }{dt}\:\:.{calculate}\:{I}_{{n}} \left(\lambda\right). \\ $$

Commented by abdo imad last updated on 01/Apr/18

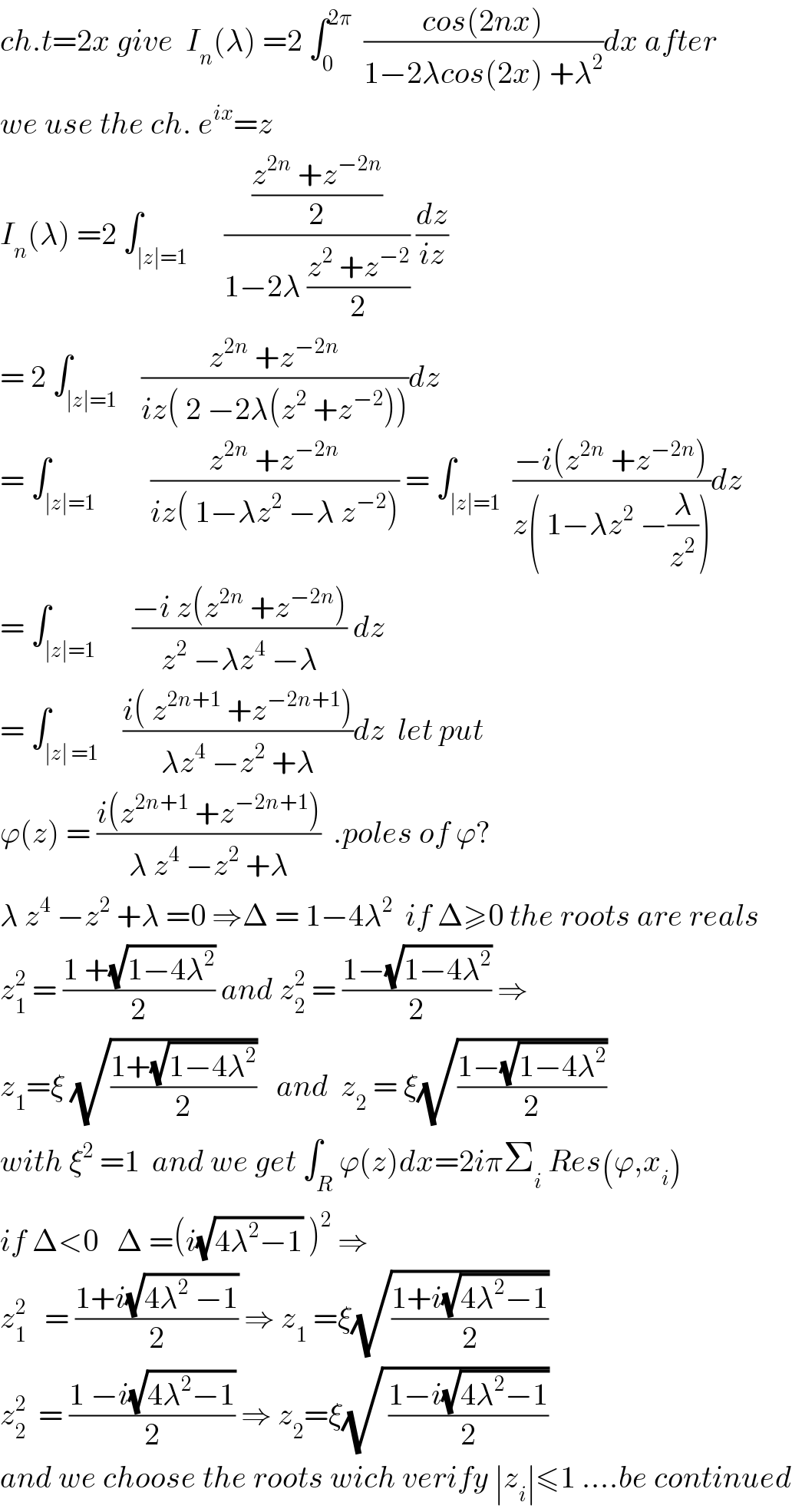
ch.t=2x give I_n (λ) =2 ∫_0 ^(2π) ((cos(2nx))/(1−2λcos(2x) +λ^2 ))dx after we use the ch. e^(ix) =z I_n (λ) =2 ∫_(∣z∣=1) (((z^(2n) +z^(−2n) )/2)/(1−2λ ((z^2 +z^(−2) )/2))) (dz/(iz)) = 2 ∫_(∣z∣=1) ((z^(2n) +z^(−2n) )/(iz( 2 −2λ(z^2 +z^(−2) ))))dz = ∫_(∣z∣=1) ((z^(2n) +z^(−2n) )/(iz( 1−λz^2 −λ z^(−2) ))) = ∫_(∣z∣=1) ((−i(z^(2n) +z^(−2n) ))/(z( 1−λz^2 −(λ/z^2 ))))dz = ∫_(∣z∣=1) ((−i z(z^(2n) +z^(−2n) ))/(z^2 −λz^4 −λ)) dz = ∫_(∣z∣ =1) ((i( z^(2n+1) +z^(−2n+1) ))/(λz^4 −z^2 +λ))dz let put ϕ(z) = ((i(z^(2n+1) +z^(−2n+1) ))/(λ z^4 −z^2 +λ)) .poles of ϕ? λ z^4 −z^2 +λ =0 ⇒Δ = 1−4λ^2 if Δ≥0 the roots are reals z_1 ^2 = ((1 +(√(1−4λ^2 )))/2) and z_2 ^2 = ((1−(√(1−4λ^2 )))/2) ⇒ z_1 =ξ (√((1+(√(1−4λ^2 )))/2)) and z_2 = ξ(√((1−(√(1−4λ^2 )))/2)) with ξ^2 =1 and we get ∫_R ϕ(z)dx=2iπΣ_i Res(ϕ,x_i ) if Δ<0 Δ =(i(√(4λ^2 −1)) )^2 ⇒ z_1 ^2 = ((1+i(√(4λ^2 −1)))/2) ⇒ z_1 =ξ(√((1+i(√(4λ^2 −1)))/2)) z_2 ^2 = ((1 −i(√(4λ^2 −1)))/2) ⇒ z_2 =ξ(√( ((1−i(√(4λ^2 −1)))/2))) and we choose the roots wich verify ∣z_i ∣≤1 ....be continued

$${ch}.{t}=\mathrm{2}{x}\:{give}\:\:{I}_{{n}} \left(\lambda\right)\:=\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\:\frac{{cos}\left(\mathrm{2}{nx}\right)}{\mathrm{1}−\mathrm{2}\lambda{cos}\left(\mathrm{2}{x}\right)\:+\lambda^{\mathrm{2}} }{dx}\:{after} \\ $$$${we}\:{use}\:{the}\:{ch}.\:{e}^{{ix}} ={z} \\ $$$${I}_{{n}} \left(\lambda\right)\:=\mathrm{2}\:\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\:\frac{\frac{{z}^{\mathrm{2}{n}} \:+{z}^{−\mathrm{2}{n}} }{\mathrm{2}}}{\mathrm{1}−\mathrm{2}\lambda\:\frac{{z}^{\mathrm{2}} \:+{z}^{−\mathrm{2}} }{\mathrm{2}}}\:\frac{{dz}}{{iz}} \\ $$$$=\:\mathrm{2}\:\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\frac{{z}^{\mathrm{2}{n}} \:+{z}^{−\mathrm{2}{n}} }{{iz}\left(\:\mathrm{2}\:−\mathrm{2}\lambda\left({z}^{\mathrm{2}} \:+{z}^{−\mathrm{2}} \right)\right)}{dz} \\ $$$$=\:\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\:\:\:\:\frac{{z}^{\mathrm{2}{n}} \:+{z}^{−\mathrm{2}{n}} }{{iz}\left(\:\mathrm{1}−\lambda{z}^{\mathrm{2}} \:−\lambda\:{z}^{−\mathrm{2}} \right)}\:=\:\int_{\mid{z}\mid=\mathrm{1}} \:\frac{−{i}\left({z}^{\mathrm{2}{n}} \:+{z}^{−\mathrm{2}{n}} \right)}{{z}\left(\:\mathrm{1}−\lambda{z}^{\mathrm{2}} \:−\frac{\lambda}{{z}^{\mathrm{2}} }\right)}{dz} \\ $$$$=\:\int_{\mid{z}\mid=\mathrm{1}} \:\:\:\:\:\frac{−{i}\:{z}\left({z}^{\mathrm{2}{n}} \:+{z}^{−\mathrm{2}{n}} \right)}{{z}^{\mathrm{2}} \:−\lambda{z}^{\mathrm{4}} \:−\lambda}\:{dz} \\ $$$$=\:\int_{\mid{z}\mid\:=\mathrm{1}} \:\:\:\frac{{i}\left(\:{z}^{\mathrm{2}{n}+\mathrm{1}} \:+{z}^{−\mathrm{2}{n}+\mathrm{1}} \right)}{\lambda{z}^{\mathrm{4}} \:−{z}^{\mathrm{2}} \:+\lambda}{dz}\:\:{let}\:{put} \\ $$$$\varphi\left({z}\right)\:=\:\frac{{i}\left({z}^{\mathrm{2}{n}+\mathrm{1}} \:+{z}^{−\mathrm{2}{n}+\mathrm{1}} \right)}{\lambda\:{z}^{\mathrm{4}} \:−{z}^{\mathrm{2}} \:+\lambda}\:\:.{poles}\:{of}\:\varphi? \\ $$$$\lambda\:{z}^{\mathrm{4}} \:−{z}^{\mathrm{2}} \:+\lambda\:=\mathrm{0}\:\Rightarrow\Delta\:=\:\mathrm{1}−\mathrm{4}\lambda^{\mathrm{2}} \:\:{if}\:\Delta\geqslant\mathrm{0}\:{the}\:{roots}\:{are}\:{reals} \\ $$$${z}_{\mathrm{1}} ^{\mathrm{2}} \:=\:\frac{\mathrm{1}\:+\sqrt{\mathrm{1}−\mathrm{4}\lambda^{\mathrm{2}} }}{\mathrm{2}}\:{and}\:{z}_{\mathrm{2}} ^{\mathrm{2}} \:=\:\frac{\mathrm{1}−\sqrt{\mathrm{1}−\mathrm{4}\lambda^{\mathrm{2}} }}{\mathrm{2}}\:\Rightarrow \\ $$$${z}_{\mathrm{1}} =\xi\:\sqrt{\frac{\mathrm{1}+\sqrt{\mathrm{1}−\mathrm{4}\lambda^{\mathrm{2}} }}{\mathrm{2}}}\:\:\:{and}\:\:{z}_{\mathrm{2}} \:=\:\xi\sqrt{\frac{\mathrm{1}−\sqrt{\mathrm{1}−\mathrm{4}\lambda^{\mathrm{2}} }}{\mathrm{2}}} \\ $$$${with}\:\xi^{\mathrm{2}} \:=\mathrm{1}\:\:{and}\:{we}\:{get}\:\int_{{R}} \varphi\left({z}\right){dx}=\mathrm{2}{i}\pi\sum_{{i}} \:{Res}\left(\varphi,{x}_{{i}} \right) \\ $$$${if}\:\Delta<\mathrm{0}\:\:\:\Delta\:=\left({i}\sqrt{\mathrm{4}\lambda^{\mathrm{2}} −\mathrm{1}}\:\right)^{\mathrm{2}} \:\Rightarrow\: \\ $$$${z}_{\mathrm{1}} ^{\mathrm{2}} \:\:\:=\:\frac{\mathrm{1}+{i}\sqrt{\mathrm{4}\lambda^{\mathrm{2}} \:−\mathrm{1}}}{\mathrm{2}}\:\Rightarrow\:{z}_{\mathrm{1}} \:=\xi\sqrt{\frac{\mathrm{1}+{i}\sqrt{\mathrm{4}\lambda^{\mathrm{2}} −\mathrm{1}}}{\mathrm{2}}} \\ $$$${z}_{\mathrm{2}} ^{\mathrm{2}} \:\:=\:\frac{\mathrm{1}\:−{i}\sqrt{\mathrm{4}\lambda^{\mathrm{2}} −\mathrm{1}}}{\mathrm{2}}\:\Rightarrow\:{z}_{\mathrm{2}} =\xi\sqrt{\:\frac{\mathrm{1}−{i}\sqrt{\mathrm{4}\lambda^{\mathrm{2}} −\mathrm{1}}}{\mathrm{2}}} \\ $$$${and}\:{we}\:{choose}\:{the}\:{roots}\:{wich}\:{verify}\:\mid{z}_{{i}} \mid\leqslant\mathrm{1}\:....{be}\:{continued} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com