
Question and Answers Forum
Question Number 36931 by maxmathsup by imad last updated on 07/Jun/18

Commented by math khazana by abdo last updated on 09/Jun/18
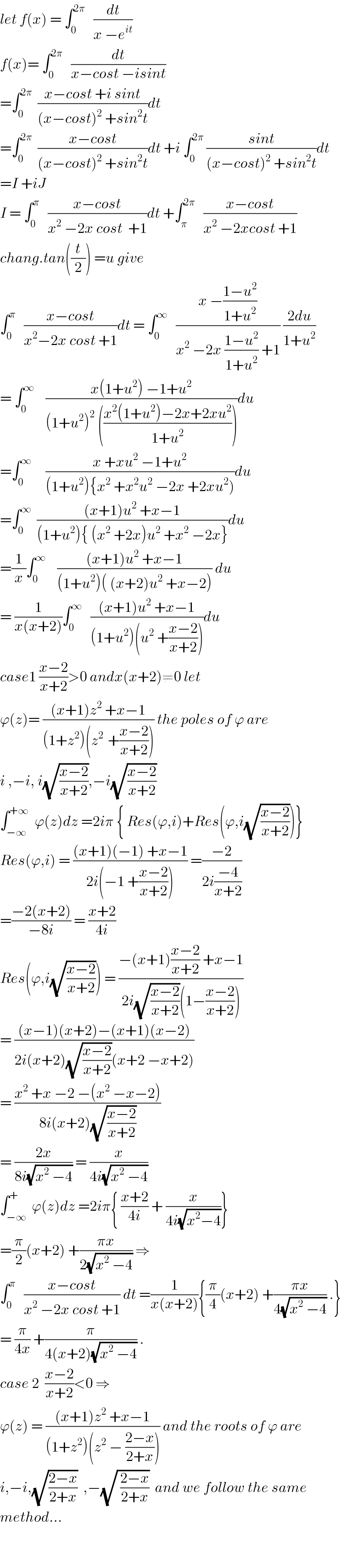
Commented by math khazana by abdo last updated on 09/Jun/18
| ||
Question and Answers Forum | ||
Question Number 36931 by maxmathsup by imad last updated on 07/Jun/18 | ||
 | ||
Commented by math khazana by abdo last updated on 09/Jun/18 | ||
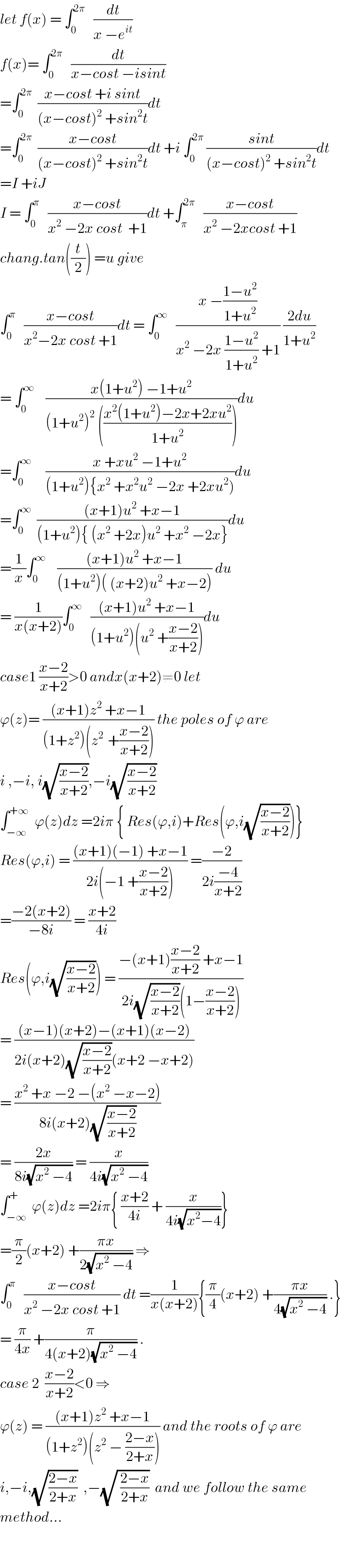 | ||
Commented by math khazana by abdo last updated on 09/Jun/18 | ||
 | ||