
Question and Answers Forum
Question Number 33705 by math khazana by abdo last updated on 22/Apr/18
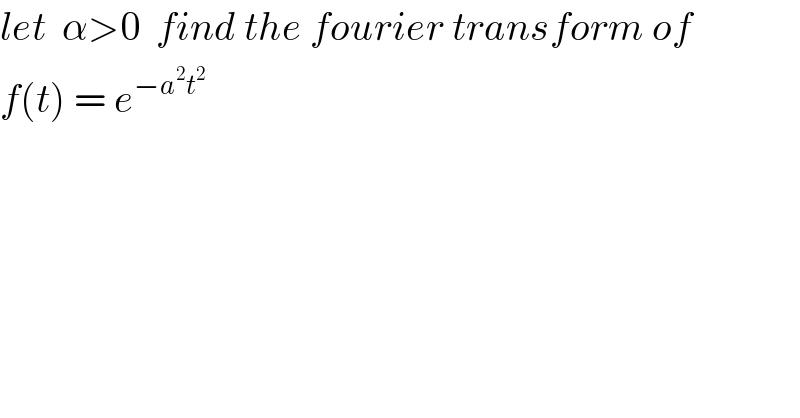
Commented bymath khazana by abdo last updated on 22/Apr/18
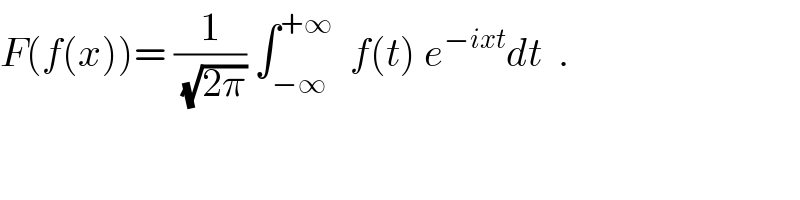
Answered by sma3l2996 last updated on 22/Apr/18
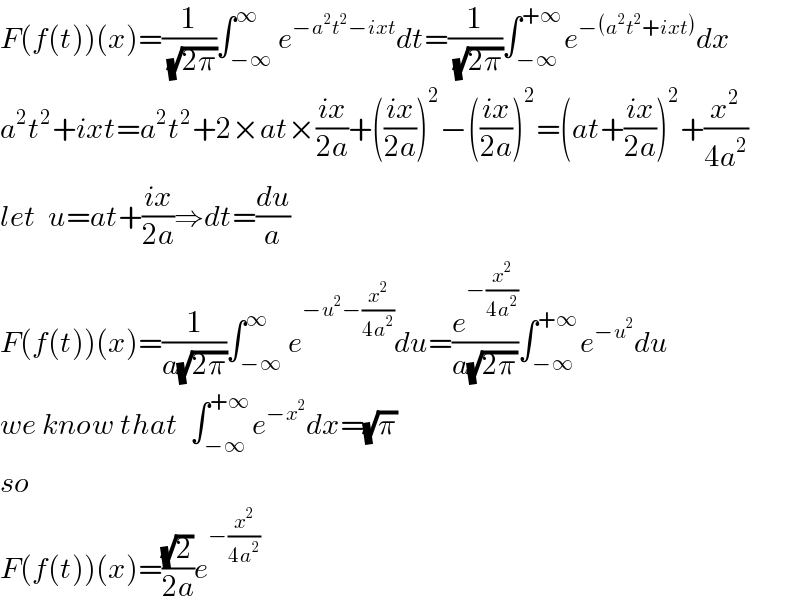
| ||
Question and Answers Forum | ||
Question Number 33705 by math khazana by abdo last updated on 22/Apr/18 | ||
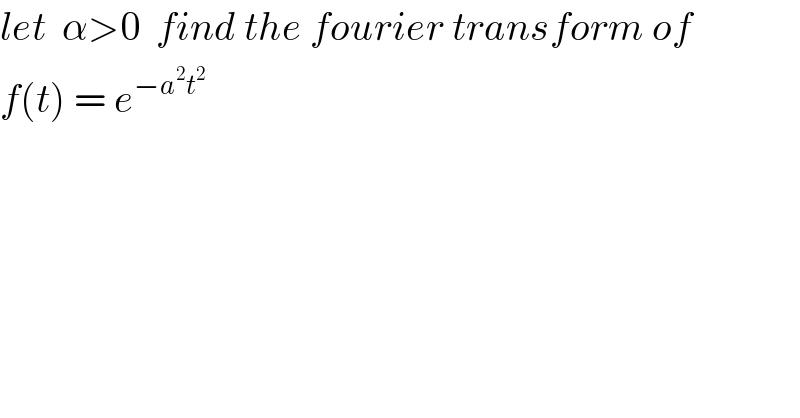 | ||
Commented bymath khazana by abdo last updated on 22/Apr/18 | ||
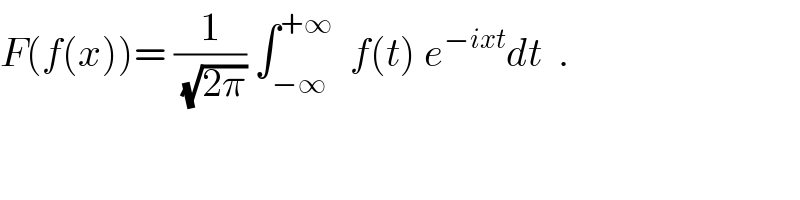 | ||
Answered by sma3l2996 last updated on 22/Apr/18 | ||
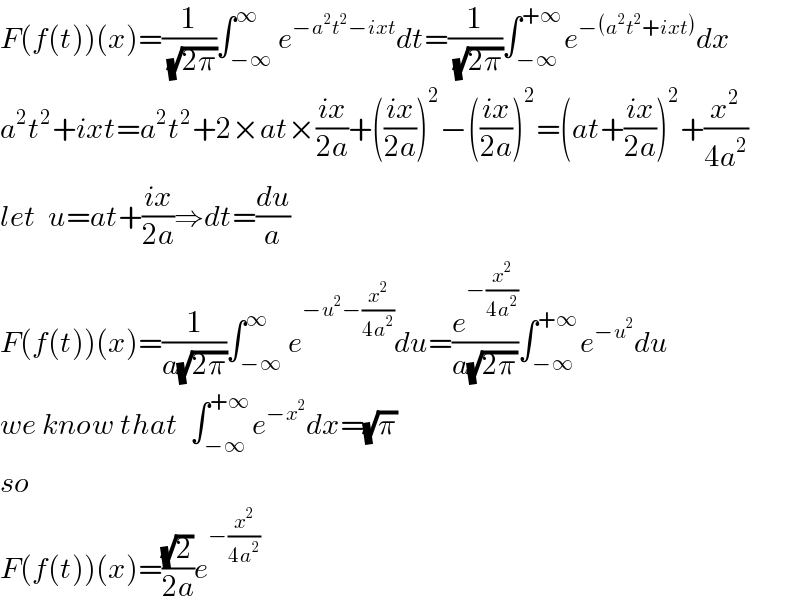 | ||
| ||