Math Question and Answers


Question and Answers Forum

All Questions Topic List
Matrices and Determinants Questions
Previous in All Question Next in All Question
Previous in Matrices and Determinants Next in Matrices and Determinants
Question Number 34355 by srikarkailash9@gmail.com last updated on 05/May/18

Commented by math khazana by abdo last updated on 05/May/18

$l e t p r o v e b y r e c u r r e n c e t h a t$ $A^{n} = (\begin{matrix} p^{n} q \frac{p^{n} - 1}{p - 1} \\ 0 1 \end{matrix})$ $w i t h p \neq 1 f o r n = 1 A^{1} = (\begin{matrix} p q \\ 0 1 \end{matrix})$ $r e l a t i o n t r u e l e t s u p p o s e A^{n} = (\begin{matrix} p^{n} q \frac{p^{n} - 1}{p - 1} \\ 0 1 \end{matrix})$ $A^{n + 1} = A^{n} . A = (\begin{matrix} p^{n} q \frac{p^{n} - 1}{p - 1} \\ 0 1 \end{matrix}) . (\begin{matrix} p q \\ 0 1 \end{matrix})$ $= (\begin{matrix} p^{n + 1} p^{n} q + q \frac{p^{n} - 1}{p - 1} \\ 0 1 \end{matrix})$ $= (\begin{matrix} p^{n + 1} q (\frac{p^{n + 1} - p^{n} + p^{n} - 1}{p - 1}) \\ 0 1 \end{matrix})$ $= (\begin{matrix} p^{n + 1} q \frac{p^{n + 1} - 1}{p - 1} \\ 0 1 \end{matrix})$ $t h e r e l a t i o n i s t r u e a t t e r m n + 1 l e t t a k e n = 8 \Rightarrow$ $A^{8} = (\begin{matrix} p^{8} q \frac{p^{8} - 1}{p - 1} \\ 0 1 \end{matrix})$
	Answered by srikarkailash9@gmail.com last updated on 05/May/18

	$s o l v e i t s t e p w i s e$
	Answered by candre last updated on 05/May/18
	$A^1 = ((p,q),(0,1) ) A^n = ((a_n ,b_n ),(c_n ,d_n ) ) A^(n+1) = ((a_(n+1) ,b_(n+1) ),(c_(n+1) ,d_(n+1) ) ) =A^n A = ((a_n ,b_n ),(c_n ,d_n ) ) ((p,q),(0,1) ) = (((a_n p),(a_n q+b_n )),((c_n p),(c_n q+d_n )) ) from where we get { ((a_(n+1) =a_n p),(a_1 =p)),((b_(n+1) =a_n q+b_n ),(b_1 =q)),((c_(n+1) =c_n p),(c_1 =0)),((d_(n+1) =c_n q+d_n ),(d_1 =0)) :} studing each separated for a a_2 =a_1 p a_3 =a_2 p=a_1 p^2 ⋮ a_n =a_1 p^(n−1) ⇒a_(n+1) =a_n p=a_1 p^n a_1 =p⇒a_n =pp^(n−1) =p^n for c similiar argument apply, but: c_1 =0⇒c_n =c_1 p^(n−1) =0 for b b_2 =a_1 q+b_1 b_3 =a_2 q+b_2 =(a_1 +a_2 )q+b_1 ⋮ b_n =(a_1 +...+a_(n−1) )q+b_1 ⇒b_(n+1) =a_n q+b_n =(a_1 +...+a_(n−1) +a_n )q+b_1 a_n =p^n ⇒Σ_(i=1) ^n a_i =p+p^2 +...+p^n =p((p^n −1)/(p−1))=((p^(n+1) −p)/(p−1))(p≠1) b_1 =q⇒b_n =qΣ_(i=1) ^(n−1) a_i +q=q(((p^n −p)/(p−1))+1)=q((p^n −p+p−1)/(p−1))=q((p^n −1)/(p−1)) similiar for d c_n =0⇒Σ_(i=1) ^n c_i =0 d_1 =1⇒d_n =qΣ_(i=1) ^(n−1) c_n +d_1 =1 so A^n = ((p^n ,(q((p^n −1)/(p−1)))),(0,1) ) where p≠1 above is just the case of n=8$
	$A^{1} = (\begin{matrix} p & q \\ 0 & 1 \end{matrix})$ $A^{n} = (\begin{matrix} a_{n} & b_{n} \\ c_{n} & d_{n} \end{matrix})$ $A^{n + 1} = (\begin{matrix} a_{n + 1} & b_{n + 1} \\ c_{n + 1} & d_{n + 1} \end{matrix})$ $= A^{n} A$ $= (\begin{matrix} a_{n} & b_{n} \\ c_{n} & d_{n} \end{matrix}) (\begin{matrix} p & q \\ 0 & 1 \end{matrix})$ $= (\begin{matrix} a_{n} p & a_{n} q + b_{n} \\ c_{n} p & c_{n} q + d_{n} \end{matrix})$ $from where we get$ ${\begin{cases} a_{n + 1} = a_{n} p & a_{1} = p \\ b_{n + 1} = a_{n} q + b_{n} & b_{1} = q \\ c_{n + 1} = c_{n} p & c_{1} = 0 \\ d_{n + 1} = c_{n} q + d_{n} & d_{1} = 0 \end{cases}$ $studing each separated$ $for a$ $a_{2} = a_{1} p$ $a_{3} = a_{2} p = a_{1} p^{2}$ $⋮$ $a_{n} = a_{1} p^{n - 1} \Rightarrow a_{n + 1} = a_{n} p = a_{1} p^{n}$ $a_{1} = p \Rightarrow a_{n} = {p p}^{n - 1} = p^{n}$ $for c similiar argument apply, b u t :$ $c_{1} = 0 \Rightarrow c_{n} = c_{1} p^{n - 1} = 0$ $for b$ $b_{2} = a_{1} q + b_{1}$ $b_{3} = a_{2} q + b_{2} = (a_{1} + a_{2}) q + b_{1}$ $⋮$ $b_{n} = (a_{1} + . . . + a_{n - 1}) q + b_{1} \Rightarrow b_{n + 1} = a_{n} q + b_{n} = (a_{1} + . . . + a_{n - 1} + a_{n}) q + b_{1}$ $a_{n} = p^{n} \Rightarrow \underset{i = 1}{\sum^{n}} a_{i} = p + p^{2} + . . . + p^{n} = p \frac{p^{n} - 1}{p - 1} = \frac{p^{n + 1} - p}{p - 1} (p \neq 1)$ $b_{1} = q \Rightarrow b_{n} = q \underset{i = 1}{\sum^{n - 1}} a_{i} + q = q (\frac{p^{n} - p}{p - 1} + 1) = q \frac{p^{n} - p + p - 1}{p - 1} = q \frac{p^{n} - 1}{p - 1}$ $similiar for d$ $c_{n} = 0 \Rightarrow \underset{i = 1}{\sum^{n}} c_{i} = 0$ $d_{1} = 1 \Rightarrow d_{n} = q \underset{i = 1}{\sum^{n - 1}} c_{n} + d_{1} = 1$ $s o$ $A^{n} = (\begin{matrix} p^{n} & q \frac{p^{n} - 1}{p - 1} \\ 0 & 1 \end{matrix}) where p \neq 1$ $above is just the case of n = 8$
	Commented by candre last updated on 05/May/18

	$if p = 1 we get$ $A^{n} = (\begin{matrix} 1 & q n \\ 0 & 1 \end{matrix})$ $;)$
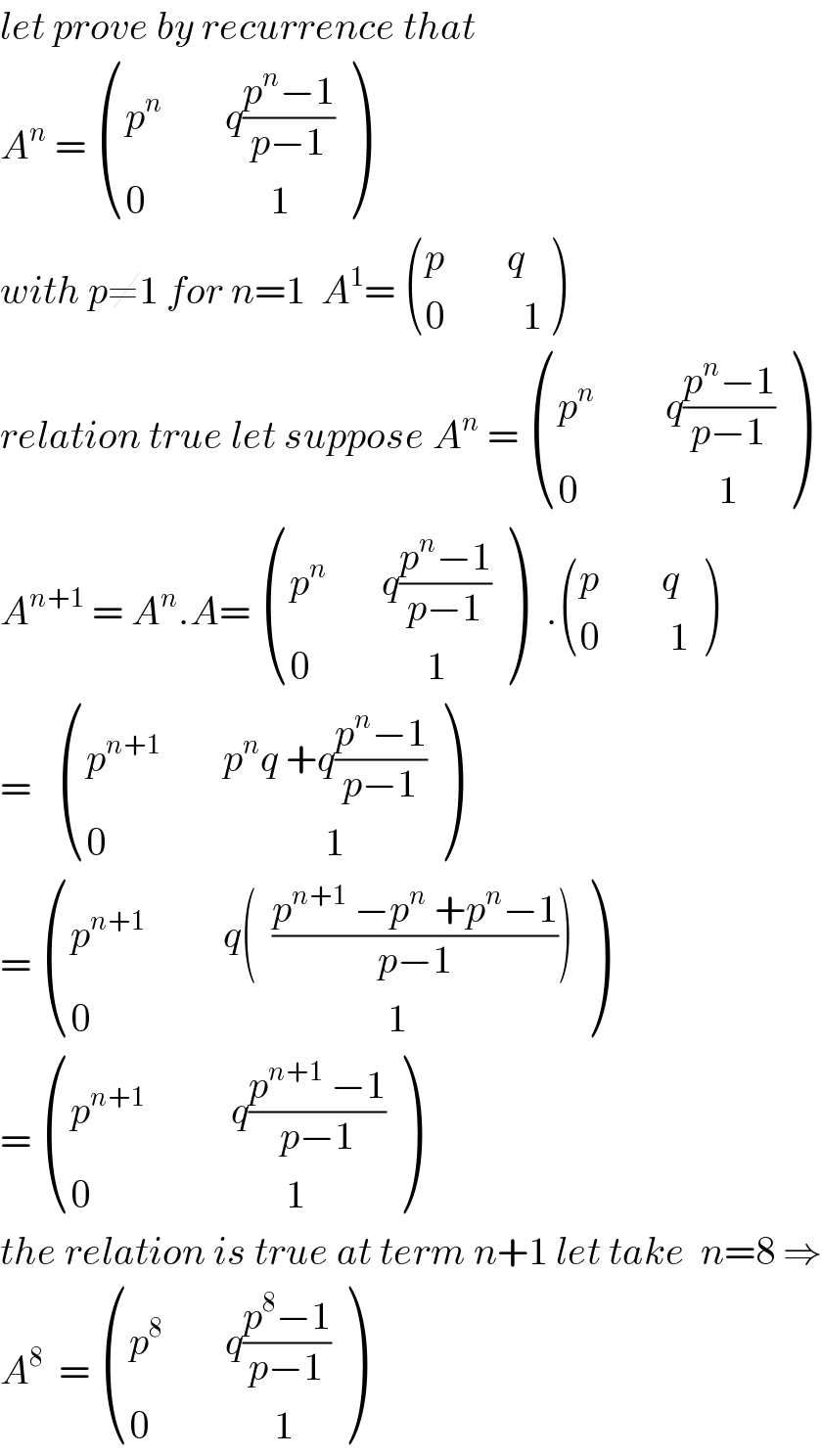
	Commented by abdo mathsup 649 cc last updated on 05/May/18

	$w h e r e a r e y o u f r o m s i r c a n d r e ?$
	Commented by candre last updated on 05/May/18

	$b r a z i l, b u t w h y t h e q u e s t i o n ?$
	Commented by math khazana by abdo last updated on 05/May/18

	$i t s o n l y a s i m l p l e i n f o r m a t i o n s i r i k n o w t h a t$ $b r a z i l i s a b e a u t i f u l c o u n t r y w i t h g o o d a n d$ $g e n e r o u s p e o p l e i a m l o o k i n g t h a t y o u a r e m o s t$ $i n t e r e s t e d t o m a t h e m a t i c s . . . i h o p e t o v i s i t b r a z i l$ $s o m e d a y . . . .$
	Commented by Rio Mike last updated on 06/May/18

	$h e y i l i v e i n B r a z i l t o S a o P a o l o$ $c a n i k n o w y o u s i r ?$
	Commented by candre last updated on 11/May/18

	$i does live in state of sao paulo, but not the city .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum