Previous in Relation and Functions Next in Relation and Functions
Question Number 34433 by abdo mathsup 649 cc last updated on 06/May/18
$${find}\:{lim}_{{n}\rightarrow+\infty} \frac{\mathrm{1}}{{n}}\sum_{{k}=\mathrm{1}} ^{{n}−\mathrm{1}} \:\sqrt{\frac{{n}+{k}}{{n}−{k}}} \\ $$
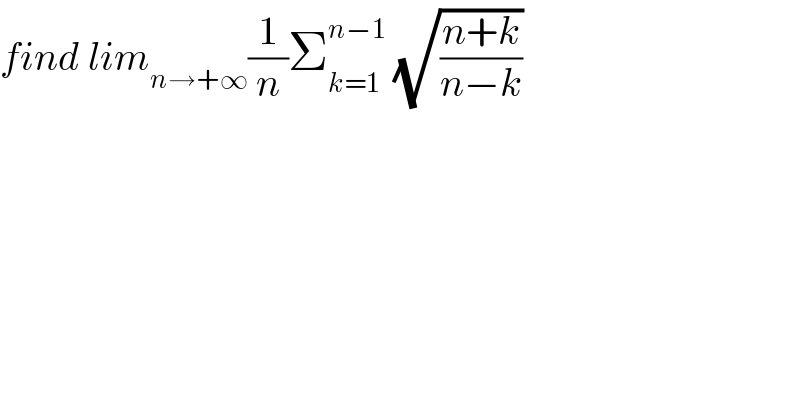
Commented by math khazana by abdo last updated on 06/May/18
![let put S_n = (1/n) Σ_(k=1) ^(n−1) (√((n+k)/(n−k))) S_n = (1/n) Σ_(k=1) ^(n−1) (√((1+(k/n))/(1−(k/n)))) ⇒ lim_(n→+∞) S_n = ∫_0 ^1 (√((1+x)/(1−x))) dx changement x= cost give ∫_0 ^1 (√(((1+x)/(1−x)) )) dx = −∫_(π/2) ^0 (√(((cos^2 ((t/2)))/(sin^2 ((t/2)))) )) sint dt = ∫_0 ^(π/2) cotan((t/2)) sint dt = ∫_0 ^(π/2) ((cos((t/2)))/(sin((t/2)))) 2sin((t/2))cos((t/2)) dt = ∫_0 ^(π/2) 2 cos^2 ((t/2))dt = ∫_0 ^(π/2) (1+cost)dt = (π/2) +[sint]_0 ^(π/2) = 1 +(π/2) ★ lim_(n→+∞) S_n = 1+(π/2) ★](Q34470.png)
$${let}\:{put}\:{S}_{{n}} \:=\:\frac{\mathrm{1}}{{n}}\:\sum_{{k}=\mathrm{1}} ^{{n}−\mathrm{1}} \:\sqrt{\frac{{n}+{k}}{{n}−{k}}} \\ $$$${S}_{{n}} =\:\frac{\mathrm{1}}{{n}}\:\sum_{{k}=\mathrm{1}} ^{{n}−\mathrm{1}} \:\:\sqrt{\frac{\mathrm{1}+\frac{{k}}{{n}}}{\mathrm{1}−\frac{{k}}{{n}}}}\:\:\:\Rightarrow \\ $$$${lim}_{{n}\rightarrow+\infty} \:\:{S}_{{n}} =\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\sqrt{\frac{\mathrm{1}+{x}}{\mathrm{1}−{x}}}\:{dx}\:\:\:{changement} \\ $$$${x}=\:{cost}\:{give}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\sqrt{\frac{\mathrm{1}+{x}}{\mathrm{1}−{x}}\:}\:{dx}\:\:=\:−\int_{\frac{\pi}{\mathrm{2}}} ^{\mathrm{0}} \sqrt{\frac{{cos}^{\mathrm{2}} \left(\frac{{t}}{\mathrm{2}}\right)}{{sin}^{\mathrm{2}} \left(\frac{{t}}{\mathrm{2}}\right)}\:}\:{sint}\:{dt} \\ $$$$=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\:{cotan}\left(\frac{{t}}{\mathrm{2}}\right)\:{sint}\:{dt} \\ $$$$=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{cos}\left(\frac{{t}}{\mathrm{2}}\right)}{{sin}\left(\frac{{t}}{\mathrm{2}}\right)}\:\mathrm{2}{sin}\left(\frac{{t}}{\mathrm{2}}\right){cos}\left(\frac{{t}}{\mathrm{2}}\right)\:{dt} \\ $$$$=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\mathrm{2}\:{cos}^{\mathrm{2}} \left(\frac{{t}}{\mathrm{2}}\right){dt}\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\left(\mathrm{1}+{cost}\right){dt} \\ $$$$=\:\frac{\pi}{\mathrm{2}}\:+\left[{sint}\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:=\:\mathrm{1}\:+\frac{\pi}{\mathrm{2}} \\ $$$$\bigstar\:\:{lim}_{{n}\rightarrow+\infty} \:{S}_{{n}} \:=\:\mathrm{1}+\frac{\pi}{\mathrm{2}}\:\:\bigstar \\ $$
Answered by arnabmaiti550@gmail.com last updated on 06/May/18
![lim_(n→∞) (1/n)Σ_(k=1) ^(n−1) (√((n+k)/(n−k))) =lim_(h→0) hΣ_(k=1) ^(n−1) (√((1/h+k)/(1/h−k))) =lim_(h→0) hΣ_(k=1) ^(n−1) (√(((1+kh)/(1−kh)) )) as h→0 h≠0 =lim_(h→0) h[ Σ_(k=0) ^(n−1) (√((1+kh)/(1−kh)))−(√((1+0.h)/(1−0.h))) ] =lim_(h→0) h Σ_(k=0) ^(n−1) (√((1+kh)/(1−kh)))−lim_(h→0 ) h =∫_(0 ) ^( 1) (√((1+x)/(1−x)))dx−0 =∫_0 ^( 1) ((1+x)/(√(1−x^2 )))dx =∫_(0 ) ^( 1) (dx/(√(1−x^2 )))+∫_0 ^( 1) ((x dx)/(√(1−x^2 ))) =[sin^(−1) (x)]_0 ^1 +∫_0 ^( (π/2)) ((sinθ cosθ dθ)/(cosθ)) [put x=sinθ] =(π/2)+∫_(0 ) ^( (π/2)) sinθ dθ =(π/2)+[−cosθ]_0 ^(π/2) =(π/2)+1](Q34451.png)
$$\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{1}}{\mathrm{n}}\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{n}−\mathrm{1}} {\sum}}\sqrt{\frac{\mathrm{n}+\mathrm{k}}{\mathrm{n}−\mathrm{k}}} \\ $$$$=\underset{\mathrm{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\mathrm{h}\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{n}−\mathrm{1}} {\sum}}\sqrt{\frac{\mathrm{1}/\mathrm{h}+\mathrm{k}}{\mathrm{1}/\mathrm{h}−\mathrm{k}}} \\ $$$$=\underset{\mathrm{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\mathrm{h}\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{n}−\mathrm{1}} {\sum}}\sqrt{\frac{\mathrm{1}+\mathrm{kh}}{\mathrm{1}−\mathrm{kh}}\:}\:\:\:\:\mathrm{as}\:\mathrm{h}\rightarrow\mathrm{0}\:\:\mathrm{h}\neq\mathrm{0} \\ $$$$=\underset{\mathrm{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\mathrm{h}\left[\:\underset{\mathrm{k}=\mathrm{0}} {\overset{\mathrm{n}−\mathrm{1}} {\sum}}\sqrt{\frac{\mathrm{1}+\mathrm{kh}}{\mathrm{1}−\mathrm{kh}}}−\sqrt{\frac{\mathrm{1}+\mathrm{0}.\mathrm{h}}{\mathrm{1}−\mathrm{0}.\mathrm{h}}}\:\right] \\ $$$$=\underset{\mathrm{h}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\mathrm{h}\:\underset{\mathrm{k}=\mathrm{0}} {\overset{\mathrm{n}−\mathrm{1}} {\sum}}\sqrt{\frac{\mathrm{1}+\mathrm{kh}}{\mathrm{1}−\mathrm{kh}}}−\underset{\mathrm{h}\rightarrow\mathrm{0}\:} {\mathrm{lim}}\:\mathrm{h} \\ $$$$=\int_{\mathrm{0}\:} ^{\:\mathrm{1}} \sqrt{\frac{\mathrm{1}+\mathrm{x}}{\mathrm{1}−\mathrm{x}}}\mathrm{dx}−\mathrm{0} \\ $$$$=\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\mathrm{1}+\mathrm{x}}{\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}\mathrm{dx} \\ $$$$=\int_{\mathrm{0}\:} ^{\:\mathrm{1}} \frac{\mathrm{dx}}{\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }}+\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\mathrm{x}\:\mathrm{dx}}{\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }} \\ $$$$=\left[\mathrm{sin}^{−\mathrm{1}} \left(\mathrm{x}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} \:+\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \frac{\mathrm{sin}\theta\:\mathrm{cos}\theta\:\mathrm{d}\theta}{\mathrm{cos}\theta}\:\:\:\left[\mathrm{put}\:\mathrm{x}=\mathrm{sin}\theta\right] \\ $$$$=\frac{\pi}{\mathrm{2}}+\int_{\mathrm{0}\:} ^{\:\frac{\pi}{\mathrm{2}}} \mathrm{sin}\theta\:\mathrm{d}\theta \\ $$$$=\frac{\pi}{\mathrm{2}}+\left[−\mathrm{cos}\theta\right]_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \\ $$$$=\frac{\pi}{\mathrm{2}}+\mathrm{1} \\ $$