lim_(x→0) ((1/x) − ((ln^(1000) (1 + x))/x^(1001) ))


Question and Answers Forum

All Questions Topic List
Limits Questions
Previous in All Question Next in All Question
Previous in Limits Next in Limits
Question Number 34755 by Joel579 last updated on 10/May/18

$\lim_{x \to 0} (\frac{1}{x} - \frac{\ln^{1000} (1 + x)}{x^{1001}})$
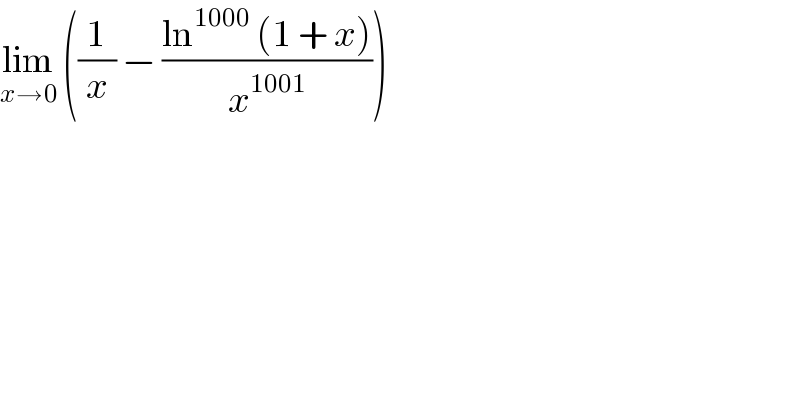
Commented by Joel579 last updated on 10/May/18

$L = \lim_{x \to 0} \frac{1}{x} (1 - \frac{\ln^{1000} (1 + x)}{x^{1000}})$ $= \lim_{x \to 0} [\frac{1 - {(\frac{\ln (1 + x)}{x})}^{1000}}{x}]$ $= \lim_{x \to 0} - 1000 {(\frac{\ln (1 + x)}{x})}^{999} . [\frac{\frac{x}{1 + x} - \ln (1 + x)}{x^{2}}] L^{'} H o p i t a l$ $= \lim_{x \to 0} [- 1000 {(1 - \frac{x}{2} + \frac{x}{3} - . . .)}^{999}] . [\frac{x - x \ln (1 + x) - \ln (1 + x)}{x^{2} (1 + x)}]$ $= - 1000 . \lim_{x \to 0} [\frac{1}{1 + x}] [\frac{x - \ln (1 + x)}{x^{2}} - \frac{\ln (1 + x)}{x}]$ $= - 1000 . 1 . \lim_{x \to 0} [\frac{x - \ln (1 + x)}{x^{2}} - (1 - \frac{x}{2} + \frac{x}{3} - . . .)]$ $= - 1000 . 1 . [\frac{1}{2} - 1]$ $L = 500$ $\ln (1 + x) = - \underset{k = 1}{\sum^{\infty}} \frac{{(- 1)}^{k} . x^{k}}{k}$ $= x - \frac{x^{2}}{2} + \frac{x^{3}}{3} - . . .$ $\frac{\ln (1 + x)}{x} = \frac{1}{x} (x - \frac{x^{2}}{2} + \frac{x^{3}}{3} - . . .)$ $= 1 - \frac{x}{2} + \frac{x}{3} - . . .$
Commented by Joel579 last updated on 10/May/18

$M a y b e s o m e o n e h a s a n o t h e r m e t h o d ?$
Commented by abdo mathsup 649 cc last updated on 11/May/18

$l e t f i n d {l i m}_{x \to o} (\frac{1}{x} - \frac{{l n}^{a} (1 + x)}{x^{a + 1}}) w i t h a f r o m N$ $= {l i m}_{x \to 0} \frac{x^{a + 1} - x {l n}^{a} (1 + x)}{x^{a + 2}} b u t$ ${l n (1 + x))}^{^{'}} = \frac{1}{1 + x} = 1 - x + o (x^{2}) \Rightarrow$ $l n (1 + x) = x - \frac{x^{2}}{2} + o (x^{3}) \Rightarrow=$ ${(l n (1 + x))}^{a} = x^{a} {(1 - \frac{x}{2} + o (x))}^{a} \sim x^{a} (1 - \frac{a x}{2})$ $\Rightarrow \frac{x^{a + 1} - x {l n}^{a} (1 + x)}{x^{a + 2}} \sim \frac{x^{a + 1} - x^{a + 1} + \frac{{a x}^{a + 2}}{2}}{x^{a + 2}}$ $\sim \frac{a}{2} \Rightarrow {l i m}_{x \to 0} (\frac{1}{x} - \frac{{l n}^{a} (1 + x)}{x^{a + 1}}) = \frac{a}{2}$ $l e t t a k e a = 1000 \Rightarrow$ ${l i m}_{x \to 0} (\frac{1}{x} - \frac{{l n}^{1000} (1 + x)}{x^{1001}}) = 500 .$
Commented by Joel579 last updated on 11/May/18

$T h a n k y o u, b u t I {d i d n}^{'} t u n d e r s t a n d$ $w h a t o (x^{2}) i s$
Commented by abdo mathsup 649 cc last updated on 11/May/18

$o (x^{2}) = x^{2} ξ (x) w i t h {l i m}_{x \to 0} ξ (x) = 0$
	Answered by tanmay.chaudhury50@gmail.com last updated on 12/May/18
	$=((lim)/(x→0)) (1/x)[1−{((ln(1+x))/x)}^(1000) ] =((lim)/(x→0))(1/x)[1−{((x−(x^2 /2)+(x^3 /3)−(x^4 /4)+...)/x)}^(1000) ] =((lim)/(x→0))(1/(x ))[1−{1−(x/2)+(x^2 /3)−(x^3 /4)+...}^(1000) ] =((lim)/(x→0))(1/x)[1−{1−ψ(x)}] here ψ(x) are the terms containing x^(1000) and higher power of x... =((lim)/(x→0))(1/x)[ψ(x)] now {1−ψ(x)}={1−(x/2)+(x^2 /3)−(x^3 /4)+...}^(1000) ={1−(x/2)}^(1000) ignoring other terms ={1−1000_C_1 .(x/2)+other terms containg x^k to the power x^(2 ) and ablve...so ψ(x)=500x+other terms clntaining x^k k≥2 =((lim)/(x→0))((500x+other terms clintaing x^k )/x) =500$
	$= \frac{l i m}{x \to 0} \frac{1}{x} [1 - {\frac{l n (1 + x)}{x}}^{1000}]$ $= \frac{l i m}{x \to 0} \frac{1}{x} [1 - {\frac{x - \frac{x^{2}}{2} + \frac{x^{3}}{3} - \frac{x^{4}}{4} + . . .}{x}}^{1000}]$ $= \frac{l i m}{x \to 0} \frac{1}{x} [1 - {1 - \frac{x}{2} + \frac{x^{2}}{3} - \frac{x^{3}}{4} + . . .}^{1000}]$ $= \frac{l i m}{x \to 0} \frac{1}{x} [1 - {1 - ψ (x)}]$ $h e r e ψ (x) a r e t h e t e r m s c o n t a i n i n g x^{1000} a n d$ $h i g h e r p o w e r o f x . . .$ $= \frac{l i m}{x \to 0} \frac{1}{x} [ψ (x)]$ $n o w {1 - ψ (x)} = {1 - \frac{x}{2} + \frac{x^{2}}{3} - \frac{x^{3}}{4} + . . .}^{1000}$ $= {1 - \frac{x}{2}}^{1000} i g n o r i n g o t h e r t e r m s$ $= {1 - 1000_{C_{1}} . \frac{x}{2} + o t h e r t e r m s c o n t a i n g x^{k} t o t h e$ $p o w e r x^{2} a n d a b l v e . . . s o ψ (x) = 500 x + o t h e r$ $t e r m s c l n t a i n i n g x^{k} k ⩾ 2$ $= \frac{l i m}{x \to 0} \frac{500 x + o t h e r t e r m s c l i n t a i n g x^{k}}{x}$ $= 500$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum