Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 34862 by a.i msup by abdo last updated on 12/May/18

find the value of f(x) = ∫_0 ^π ((cosx)/(1+2sin(2x)))dx

$${find}\:{the}\:{value}\:{of} \\ $$$${f}\left({x}\right)\:=\:\int_{\mathrm{0}} ^{\pi} \:\:\:\frac{{cosx}}{\mathrm{1}+\mathrm{2}{sin}\left(\mathrm{2}{x}\right)}{dx} \\ $$

Commented by prof Abdo imad last updated on 14/May/18

thank you sir for this remark..i have working a lot in complexe analysis but i find often something that we can t understand and explain it s more and more difficult...

$${thank}\:{you}\:{sir}\:{for}\:{this}\:{remark}..{i}\:{have}\:{working} \\ $$$${a}\:{lot}\:{in}\:{complexe}\:{analysis}\:{but}\:{i}\:{find}\:{often} \\ $$$${something}\:{that}\:{we}\:{can}\:{t}\:\:{understand}\:{and} \\ $$$${explain}\:\:{it}\:{s}\:{more}\:{and}\:{more}\:{difficult}... \\ $$

Commented by prof Abdo imad last updated on 12/May/18

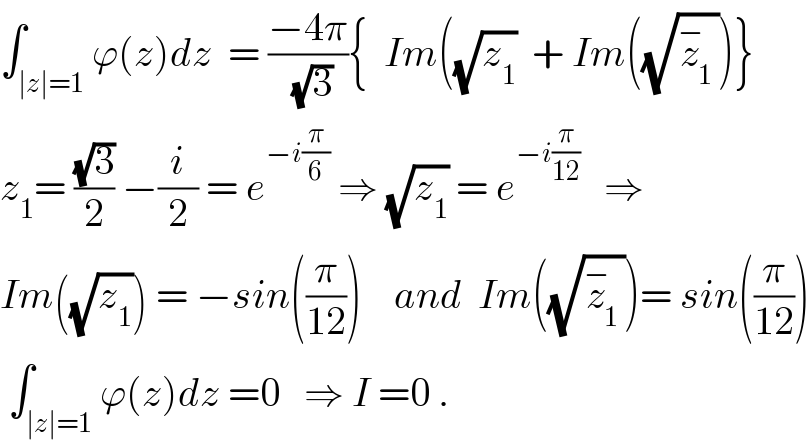
∫_(∣z∣=1) ϕ(z)dz = ((−4π)/(√3)){ Im((√z_1 ) + Im((√z_1 ^− ))} z_1 = ((√3)/2) −(i/2) = e^(−i(π/6)) ⇒ (√z_1 ) = e^(−i(π/(12))) ⇒ Im((√z_1 )) = −sin((π/(12))) and Im((√z_1 ^− ))= sin((π/(12))) ∫_(∣z∣=1) ϕ(z)dz =0 ⇒ I =0 .

$$\int_{\mid{z}\mid=\mathrm{1}} \varphi\left({z}\right){dz}\:\:=\:\frac{−\mathrm{4}\pi}{\sqrt{\mathrm{3}}}\left\{\:\:{Im}\left(\sqrt{{z}_{\mathrm{1}} }\:\:+\:{Im}\left(\sqrt{\overset{−} {{z}}_{\mathrm{1}} }\right)\right\}\right. \\ $$$${z}_{\mathrm{1}} =\:\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:−\frac{{i}}{\mathrm{2}}\:=\:{e}^{−{i}\frac{\pi}{\mathrm{6}}} \:\Rightarrow\:\sqrt{{z}_{\mathrm{1}} }\:=\:{e}^{−{i}\frac{\pi}{\mathrm{12}}} \:\:\:\Rightarrow \\ $$$${Im}\left(\sqrt{{z}_{\mathrm{1}} }\right)\:=\:−{sin}\left(\frac{\pi}{\mathrm{12}}\right)\:\:\:\:{and}\:\:{Im}\left(\sqrt{\overset{−} {{z}}_{\mathrm{1}} }\right)=\:{sin}\left(\frac{\pi}{\mathrm{12}}\right) \\ $$$$\:\int_{\mid{z}\mid=\mathrm{1}} \varphi\left({z}\right){dz}\:=\mathrm{0}\:\:\:\Rightarrow\:{I}\:=\mathrm{0}\:. \\ $$

Commented by MJS last updated on 13/May/18

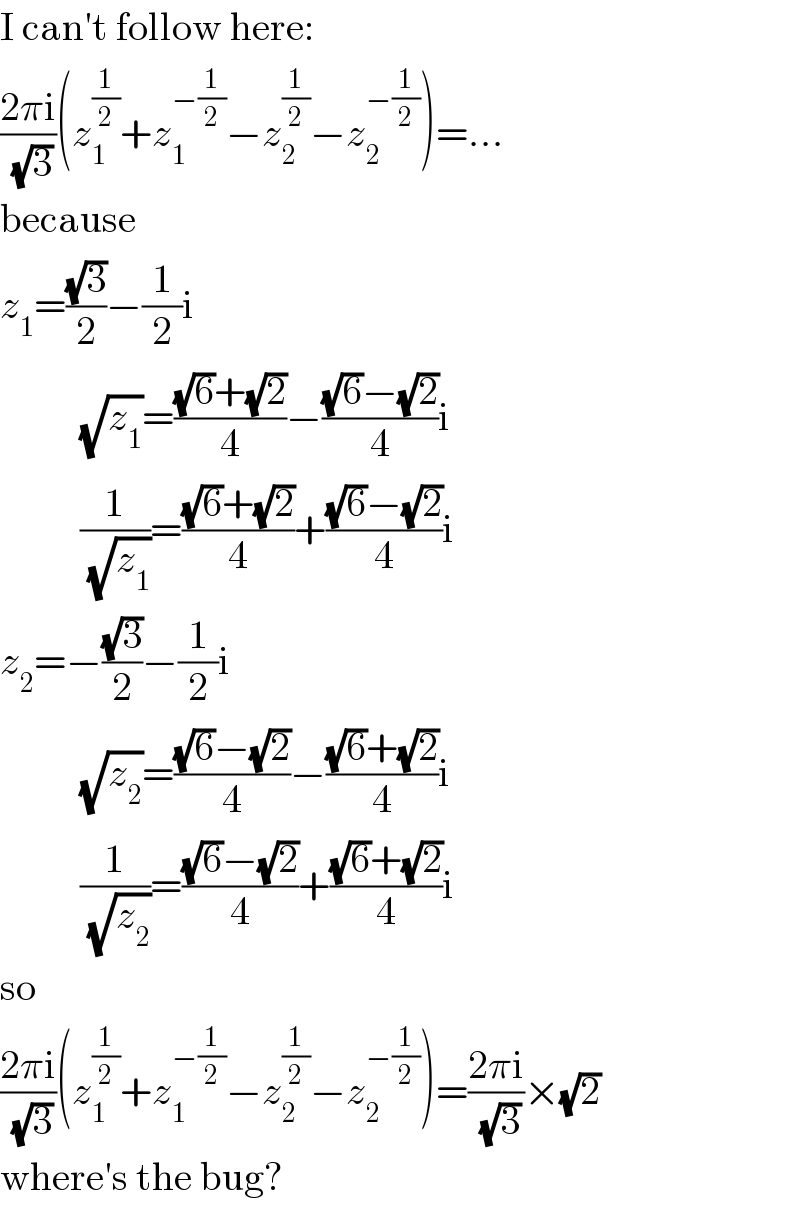
I can′t follow here: ((2πi)/(√3))(z_1 ^(1/2) +z_1 ^(−(1/2)) −z_2 ^(1/2) −z_2 ^(−(1/2)) )=... because z_1 =((√3)/2)−(1/2)i (√z_1 )=(((√6)+(√2))/4)−(((√6)−(√2))/4)i (1/(√z_1 ))=(((√6)+(√2))/4)+(((√6)−(√2))/4)i z_2 =−((√3)/2)−(1/2)i (√z_2 )=(((√6)−(√2))/4)−(((√6)+(√2))/4)i (1/(√z_2 ))=(((√6)−(√2))/4)+(((√6)+(√2))/4)i so ((2πi)/(√3))(z_1 ^(1/2) +z_1 ^(−(1/2)) −z_2 ^(1/2) −z_2 ^(−(1/2)) )=((2πi)/(√3))×(√2) where′s the bug?

$$\mathrm{I}\:\mathrm{can}'\mathrm{t}\:\mathrm{follow}\:\mathrm{here}: \\ $$$$\frac{\mathrm{2}\pi\mathrm{i}}{\sqrt{\mathrm{3}}}\left({z}_{\mathrm{1}} ^{\frac{\mathrm{1}}{\mathrm{2}}} +{z}_{\mathrm{1}} ^{−\frac{\mathrm{1}}{\mathrm{2}}} −{z}_{\mathrm{2}} ^{\frac{\mathrm{1}}{\mathrm{2}}} −{z}_{\mathrm{2}} ^{−\frac{\mathrm{1}}{\mathrm{2}}} \right)=... \\ $$$$\mathrm{because} \\ $$$${z}_{\mathrm{1}} =\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{i} \\ $$$$\:\:\:\:\:\:\:\:\:\:\sqrt{{z}_{\mathrm{1}} }=\frac{\sqrt{\mathrm{6}}+\sqrt{\mathrm{2}}}{\mathrm{4}}−\frac{\sqrt{\mathrm{6}}−\sqrt{\mathrm{2}}}{\mathrm{4}}\mathrm{i} \\ $$$$\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{1}}{\sqrt{{z}_{\mathrm{1}} }}=\frac{\sqrt{\mathrm{6}}+\sqrt{\mathrm{2}}}{\mathrm{4}}+\frac{\sqrt{\mathrm{6}}−\sqrt{\mathrm{2}}}{\mathrm{4}}\mathrm{i} \\ $$$${z}_{\mathrm{2}} =−\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}\mathrm{i} \\ $$$$\:\:\:\:\:\:\:\:\:\:\sqrt{{z}_{\mathrm{2}} }=\frac{\sqrt{\mathrm{6}}−\sqrt{\mathrm{2}}}{\mathrm{4}}−\frac{\sqrt{\mathrm{6}}+\sqrt{\mathrm{2}}}{\mathrm{4}}\mathrm{i} \\ $$$$\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{1}}{\sqrt{{z}_{\mathrm{2}} }}=\frac{\sqrt{\mathrm{6}}−\sqrt{\mathrm{2}}}{\mathrm{4}}+\frac{\sqrt{\mathrm{6}}+\sqrt{\mathrm{2}}}{\mathrm{4}}\mathrm{i} \\ $$$$\mathrm{so} \\ $$$$\frac{\mathrm{2}\pi\mathrm{i}}{\sqrt{\mathrm{3}}}\left({z}_{\mathrm{1}} ^{\frac{\mathrm{1}}{\mathrm{2}}} +{z}_{\mathrm{1}} ^{−\frac{\mathrm{1}}{\mathrm{2}}} −{z}_{\mathrm{2}} ^{\frac{\mathrm{1}}{\mathrm{2}}} −{z}_{\mathrm{2}} ^{−\frac{\mathrm{1}}{\mathrm{2}}} \right)=\frac{\mathrm{2}\pi\mathrm{i}}{\sqrt{\mathrm{3}}}×\sqrt{\mathrm{2}} \\ $$$$\mathrm{where}'\mathrm{s}\:\mathrm{the}\:\mathrm{bug}? \\ $$

Commented by abdo mathsup 649 cc last updated on 13/May/18

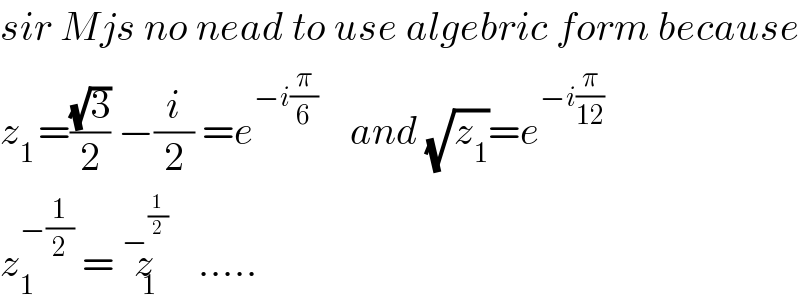
sir Mjs no nead to use algebric form because z_(1 ) =((√3)/2) −(i/2) =e^(−i(π/6)) and (√z_1 )=e^(−i(π/(12))) z_1 ^(−(1/2)) = z_1 ^−^(1/2) .....

$${sir}\:{Mjs}\:{no}\:{nead}\:{to}\:{use}\:{algebric}\:{form}\:{because} \\ $$$${z}_{\mathrm{1}\:} =\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:−\frac{{i}}{\mathrm{2}}\:={e}^{−{i}\frac{\pi}{\mathrm{6}}} \:\:\:\:{and}\:\sqrt{{z}_{\mathrm{1}} }={e}^{−{i}\frac{\pi}{\mathrm{12}}} \\ $$$${z}_{\mathrm{1}} ^{−\frac{\mathrm{1}}{\mathrm{2}}} \:=\:\overset{−^{\frac{\mathrm{1}}{\mathrm{2}}} } {{z}}_{\mathrm{1}} \:\:..... \\ $$

Commented by MJS last updated on 14/May/18

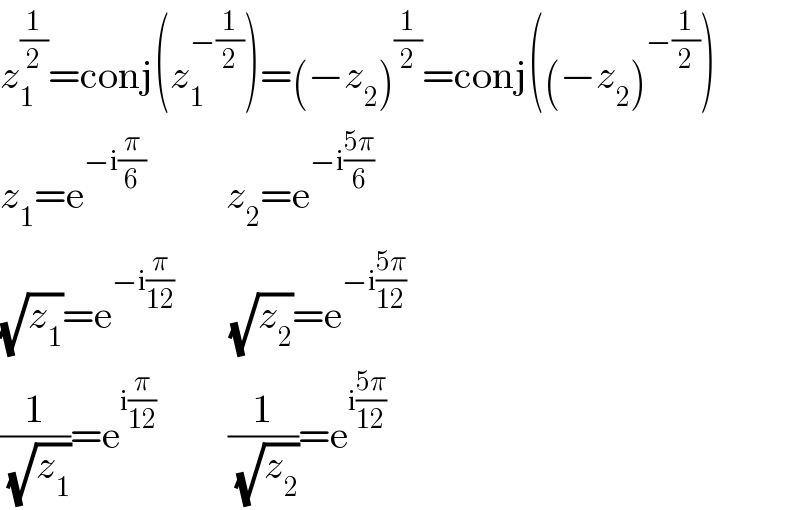
z_1 ^(1/2) =conj(z_1 ^(−(1/2)) )=(−z_2 )^(1/2) =conj((−z_2 )^(−(1/2)) ) z_1 =e^(−i(π/6)) z_2 =e^(−i((5π)/6)) (√z_1 )=e^(−i(π/(12))) (√z_2 )=e^(−i((5π)/(12))) (1/(√z_1 ))=e^(i(π/(12))) (1/(√z_2 ))=e^(i((5π)/(12)))

$${z}_{\mathrm{1}} ^{\frac{\mathrm{1}}{\mathrm{2}}} =\mathrm{conj}\left({z}_{\mathrm{1}} ^{−\frac{\mathrm{1}}{\mathrm{2}}} \right)=\left(−{z}_{\mathrm{2}} \right)^{\frac{\mathrm{1}}{\mathrm{2}}} =\mathrm{conj}\left(\left(−{z}_{\mathrm{2}} \right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \right) \\ $$$${z}_{\mathrm{1}} =\mathrm{e}^{−\mathrm{i}\frac{\pi}{\mathrm{6}}} \:\:\:\:\:\:\:\:\:\:{z}_{\mathrm{2}} =\mathrm{e}^{−\mathrm{i}\frac{\mathrm{5}\pi}{\mathrm{6}}} \\ $$$$\sqrt{{z}_{\mathrm{1}} }=\mathrm{e}^{−\mathrm{i}\frac{\pi}{\mathrm{12}}} \:\:\:\:\:\:\:\sqrt{{z}_{\mathrm{2}} }=\mathrm{e}^{−\mathrm{i}\frac{\mathrm{5}\pi}{\mathrm{12}}} \\ $$$$\frac{\mathrm{1}}{\sqrt{{z}_{\mathrm{1}} }}=\mathrm{e}^{\mathrm{i}\frac{\pi}{\mathrm{12}}} \:\:\:\:\:\:\:\:\:\frac{\mathrm{1}}{\sqrt{{z}_{\mathrm{2}} }}=\mathrm{e}^{\mathrm{i}\frac{\mathrm{5}\pi}{\mathrm{12}}} \\ $$

Commented by MJS last updated on 14/May/18

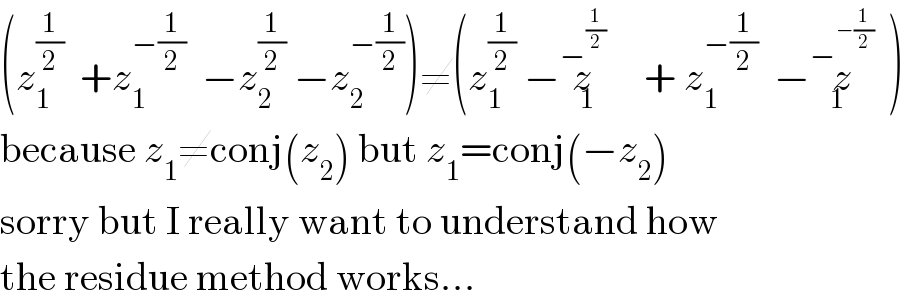
(z_1 ^(1/2) +z_1 ^(−(1/2)) −z_2 ^(1/2) −z_2 ^(−(1/2)) )≠(z_1 ^(1/2) −z_1 ^−^(1/2) + z_1 ^(−(1/2)) −z_1 ^−^(−(1/2)) ) because z_1 ≠conj(z_2 ) but z_1 =conj(−z_2 ) sorry but I really want to understand how the residue method works...

$$\left({z}_{\mathrm{1}} ^{\frac{\mathrm{1}}{\mathrm{2}}} \:\:+{z}_{\mathrm{1}} ^{−\frac{\mathrm{1}}{\mathrm{2}}} \:\:−{z}_{\mathrm{2}} ^{\frac{\mathrm{1}}{\mathrm{2}}} \:−{z}_{\mathrm{2}} ^{−\frac{\mathrm{1}}{\mathrm{2}}} \right)\neq\left({z}_{\mathrm{1}} ^{\frac{\mathrm{1}}{\mathrm{2}}} \:−\overset{−^{\frac{\mathrm{1}}{\mathrm{2}}} } {{z}}_{\mathrm{1}} \:\:\:+\:{z}_{\mathrm{1}} ^{−\frac{\mathrm{1}}{\mathrm{2}}} \:\:−\overset{−^{−\frac{\mathrm{1}}{\mathrm{2}}} } {{z}}_{\mathrm{1}} \right) \\ $$$$\mathrm{because}\:{z}_{\mathrm{1}} \neq\mathrm{conj}\left({z}_{\mathrm{2}} \right)\:\mathrm{but}\:{z}_{\mathrm{1}} =\mathrm{conj}\left(−{z}_{\mathrm{2}} \right) \\ $$$$\mathrm{sorry}\:\mathrm{but}\:\mathrm{I}\:\mathrm{really}\:\mathrm{want}\:\mathrm{to}\:\mathrm{understand}\:\mathrm{how} \\ $$$$\mathrm{the}\:\mathrm{residue}\:\mathrm{method}\:\mathrm{works}... \\ $$

Commented by abdo mathsup 649 cc last updated on 14/May/18

sir Mjs i will delet my method used in this integral and try another because the consept of residus is more difficult in some cases....

$${sir}\:{Mjs}\:{i}\:{will}\:{delet}\:{my}\:{method}\:{used}\:{in}\:{this}\:{integral} \\ $$$${and}\:{try}\:{another}\:{because}\:{the}\:{consept}\:{of}\:{residus} \\ $$$${is}\:{more}\:{difficult}\:{in}\:{some}\:{cases}.... \\ $$

Commented by MJS last updated on 14/May/18

Sir, I can′t say if your method is right or wrong, I′m just trying to follow and hopefully understand what you′re doing. I believe you′re very much better in these things than I am, since I had been studying maths about 25 years ago and I have forgotten many things... I′ll be truly thankful for any explanation you give in this or another case

$$\mathrm{Sir},\:\mathrm{I}\:\mathrm{can}'\mathrm{t}\:\mathrm{say}\:\mathrm{if}\:\mathrm{your}\:\mathrm{method}\:\mathrm{is}\:\mathrm{right}\:\mathrm{or} \\ $$$$\mathrm{wrong},\:\mathrm{I}'\mathrm{m}\:\mathrm{just}\:\mathrm{trying}\:\mathrm{to}\:\mathrm{follow}\:\mathrm{and} \\ $$$$\mathrm{hopefully}\:\mathrm{understand}\:\mathrm{what}\:\mathrm{you}'\mathrm{re}\:\mathrm{doing}. \\ $$$$\mathrm{I}\:\mathrm{believe}\:\mathrm{you}'\mathrm{re}\:\mathrm{very}\:\mathrm{much}\:\mathrm{better}\:\mathrm{in}\:\mathrm{these} \\ $$$$\mathrm{things}\:\mathrm{than}\:\mathrm{I}\:\mathrm{am},\:\mathrm{since}\:\mathrm{I}\:\mathrm{had}\:\mathrm{been}\:\mathrm{studying} \\ $$$$\mathrm{maths}\:\mathrm{about}\:\mathrm{25}\:\mathrm{years}\:\mathrm{ago}\:\mathrm{and}\:\mathrm{I}\:\mathrm{have}\:\mathrm{forgotten} \\ $$$$\mathrm{many}\:\mathrm{things}...\:\mathrm{I}'\mathrm{ll}\:\mathrm{be}\:\mathrm{truly}\:\mathrm{thankful}\:\mathrm{for}\:\mathrm{any} \\ $$$$\mathrm{explanation}\:\mathrm{you}\:\mathrm{give}\:\mathrm{in}\:\mathrm{this}\:\mathrm{or}\:\mathrm{another}\:\mathrm{case} \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 12/May/18

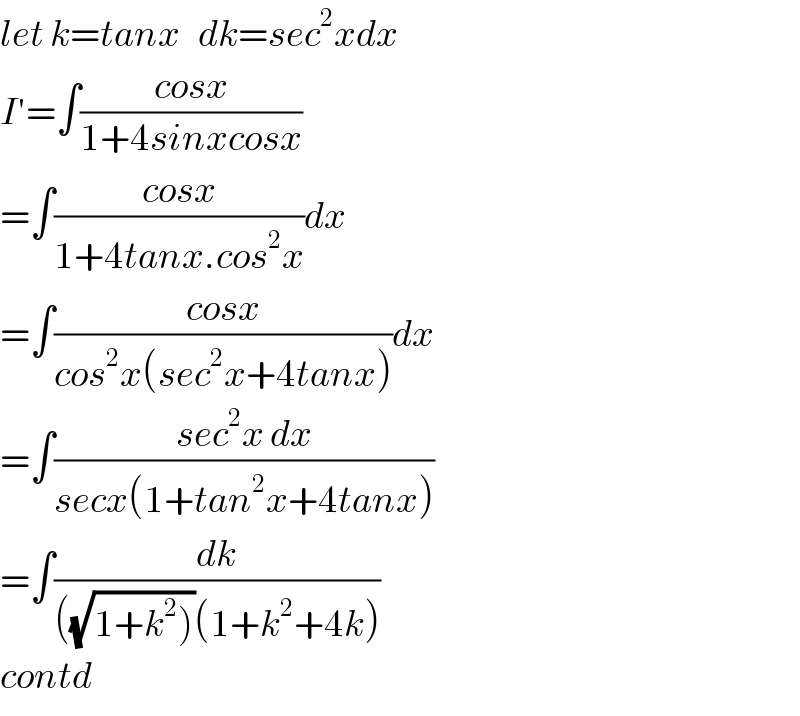
let k=tanx dk=sec^2 xdx I′=∫((cosx)/(1+4sinxcosx)) =∫((cosx)/(1+4tanx.cos^2 x))dx =∫((cosx)/(cos^2 x(sec^2 x+4tanx)))dx =∫((sec^2 x dx)/(secx(1+tan^2 x+4tanx))) =∫(dk/(((√(1+k^2 )))(1+k^2 +4k))) contd

$${let}\:{k}={tanx}\:\:\:{dk}={sec}^{\mathrm{2}} {xdx} \\ $$$${I}'=\int\frac{{cosx}}{\mathrm{1}+\mathrm{4}{sinxcosx}} \\ $$$$=\int\frac{{cosx}}{\mathrm{1}+\mathrm{4}{tanx}.{cos}^{\mathrm{2}} {x}}{dx} \\ $$$$=\int\frac{{cosx}}{{cos}^{\mathrm{2}} {x}\left({sec}^{\mathrm{2}} {x}+\mathrm{4}{tanx}\right)}{dx} \\ $$$$=\int\frac{{sec}^{\mathrm{2}} {x}\:{dx}}{{secx}\left(\mathrm{1}+{tan}^{\mathrm{2}} {x}+\mathrm{4}{tanx}\right)} \\ $$$$=\int\frac{{dk}}{\left(\sqrt{\left.\mathrm{1}+{k}^{\mathrm{2}} \right)}\left(\mathrm{1}+{k}^{\mathrm{2}} +\mathrm{4}{k}\right)\right.} \\ $$$${contd} \\ $$

Answered by math1967 last updated on 12/May/18

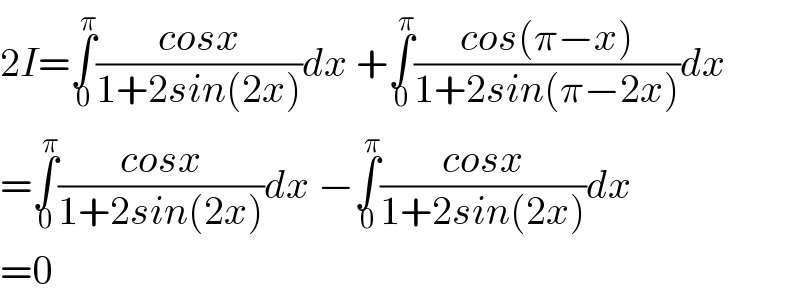
2I=∫_0 ^π ((cosx)/(1+2sin(2x)))dx +∫_0 ^π ((cos(π−x))/(1+2sin(π−2x)))dx =∫_0 ^π ((cosx)/(1+2sin(2x)))dx −∫_0 ^π ((cosx)/(1+2sin(2x)))dx =0

$$\mathrm{2}{I}=\underset{\mathrm{0}} {\overset{\pi} {\int}}\frac{{cosx}}{\mathrm{1}+\mathrm{2}{sin}\left(\mathrm{2}{x}\right)}{dx}\:+\underset{\mathrm{0}} {\overset{\pi} {\int}}\frac{{cos}\left(\pi−{x}\right)}{\mathrm{1}+\mathrm{2}{sin}\left(\pi−\mathrm{2}{x}\right)}{dx} \\ $$$$=\underset{\mathrm{0}} {\overset{\pi} {\int}}\frac{{cosx}}{\mathrm{1}+\mathrm{2}{sin}\left(\mathrm{2}{x}\right)}{dx}\:−\underset{\mathrm{0}} {\overset{\pi} {\int}}\frac{{cosx}}{\mathrm{1}+\mathrm{2}{sin}\left(\mathrm{2}{x}\right)}{dx} \\ $$$$=\mathrm{0} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com