
Question and Answers Forum
Question Number 34901 by rishabh last updated on 12/May/18
![∫_(−π/2) ^(+π/2) (√(cos^(2n−1) x−cos^(2n+1) x))dx =[−((2cos^((2n+1)/2) x)/(2n+1))]_(−π/2) ^(+π/2) =0? What is the mistake in above? ∫_(−π/2) ^(+π/2) (√(cos^(2n−1) x−cos^(2n+1) x))dx =2∫_0 ^(π/2) (√(cos^(2n−1) x−cos^(2n+1) x))dx =(4/(2n+1)) (this is correct answer)](Q34901.png)
Commented by rishabh last updated on 13/May/18
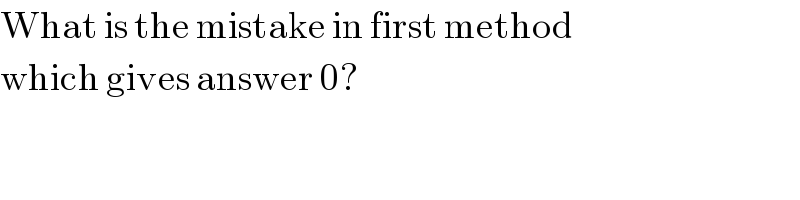
Commented by math khazana by abdo last updated on 13/May/18
![I = 2 ∫_0 ^(π/2) (√(cos^(2n−1) x −cos^(2n+1) x)) dx chang. cos^(2n+1) x =t ⇒cosx =t^(1/(2n+1)) ⇒ x =arcos(t^(1/(2n+1)) ) cos^(2n−1) x= cos^(2n+1 −2) =(t/(cos^2 x)) = (t/t^(2/(2n+1)) ) ⇒ I = 2 ∫_1 ^0 (√( (t/t^(2/(2n+1)) )−t)) (1/(2n+1)) t^((1/(2n+1))−1) ((−dt)/(√(1−t^(2/(2n+1)) ))) = (2/(2n+1)) ∫_0 ^1 (√t) (√((1−t^(2/(2n+1)) )/t^(2/(2n+1)) )) .t^((1/(2n+1))−1) (dt/(√(1−t^(2/(2n+1)) ))) = (2/(2n+1)) ∫_0 ^1 (√t) t^(1/(2n+1)) t^( (1/(2n+1)) −1) dt = (2/(2n+1)) ∫_0 ^1 (√t) t^((2/(2n+1))−1) dt ((√t)=x) = (2/(2n+1)) ∫_0 ^1 x . (x^2 )^((2/(2n+1))−1 ) dx = (2/(2n+1)) ∫_0 ^1 x^(4/(2n+1)) dx = (2/(2n+1))[ (1/((4/(2n+1))+1)) x^((4/(2n+1))+1) ]_0 ^1 = (2/(2n+1)) (1/((2n+5)/(2n+1))) =(2/(2n+5))](Q34943.png)
Commented by math khazana by abdo last updated on 13/May/18
![error in the final lines I = (2/(2n+1)) ∫_0 ^1 x (x^2 )^((2/(2n+1))−1) (2xdx) I = (4/(2n+1)) ∫_0 ^1 x^2 x^((4/(2n+1)) −2) dx = (4/(2n+1)) ∫_0 ^1 x^(4/(2n+1)) dx = (4/(2n+1))[ (1/((4/(2n+1)) +1)) x^((4/(2n+1)) +1) ]_0 ^1 = (4/(2n+1)) .(1/((2n+5)/(2n+1))) = (4/(2n+5)) I = (4/(2n+5)) .](Q34946.png)
