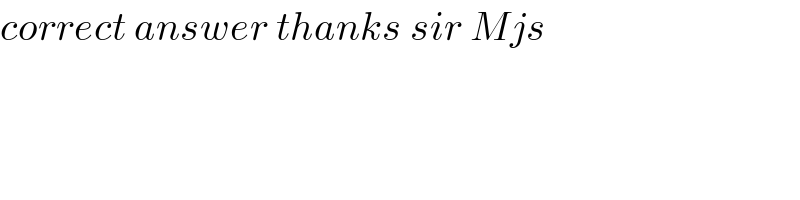Question Number 35044 by math khazana by abdo last updated on 14/May/18
$$\left.\mathrm{1}\right){find}\:\int\:\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:{dt} \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\:\int_{\mathrm{1}} ^{\sqrt{\mathrm{3}}} \:\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:\:{dt} \\ $$
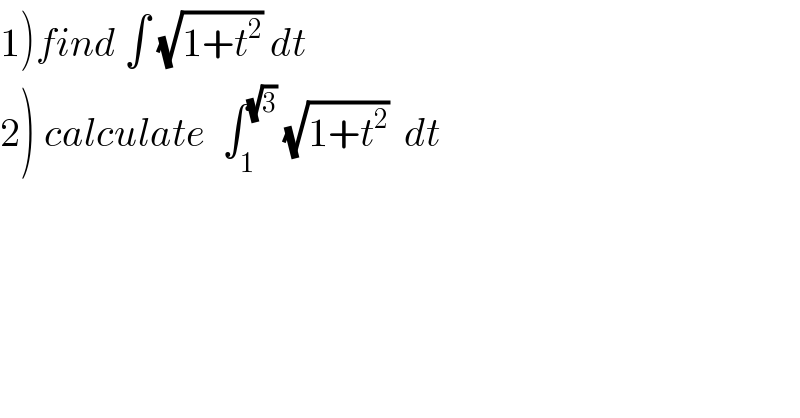
Commented by math khazana by abdo last updated on 15/May/18
![let put I = ∫(√(1+t^2 )) dt changement t =shx give I = ∫ chx chxdx =∫ ch^2 xdx =∫ ((1+ch(2x))/2)dx = (x/2) +(1/4)sh(2x) = (x/2) + (1/2)shx chx = ((argsh(t))/2) +(1/2)t(√(1+t^2 )) =(1/2)ln(t +(√(1+t^2 )) ) +(1/2)t(√(1+t^2 )) +c 2) ∫_1 ^(√3) (√(1+t^2 )) dt =[(1/2)ln(t+(√(1+t^2 )) ) +(1/2)t(√(1+t^2 )) ]_1 ^(√3) =(1/2){ ln( 2+(√3)) +((√3)/2) .2 −ln(1+(√2)) −(√2) } =(1/2){ ln(2+(√3)) −ln(1+(√2)) +(√3) −(√2) }](Q35064.png)
$${let}\:{put}\:{I}\:=\:\int\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:{dt}\:\:{changement}\:{t}\:={shx}\:{give} \\ $$$${I}\:=\:\int\:{chx}\:{chxdx}\:=\int\:{ch}^{\mathrm{2}} {xdx}\: \\ $$$$=\int\:\frac{\mathrm{1}+{ch}\left(\mathrm{2}{x}\right)}{\mathrm{2}}{dx}\:=\:\frac{{x}}{\mathrm{2}}\:\:+\frac{\mathrm{1}}{\mathrm{4}}{sh}\left(\mathrm{2}{x}\right) \\ $$$$=\:\frac{{x}}{\mathrm{2}}\:\:+\:\frac{\mathrm{1}}{\mathrm{2}}{shx}\:{chx}\:=\:\frac{{argsh}\left({t}\right)}{\mathrm{2}}\:+\frac{\mathrm{1}}{\mathrm{2}}{t}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}\:+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:\right)\:+\frac{\mathrm{1}}{\mathrm{2}}{t}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:\:+{c} \\ $$$$\left.\mathrm{2}\right)\:\int_{\mathrm{1}} ^{\sqrt{\mathrm{3}}} \:\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:{dt}\:=\left[\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({t}+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:\right)\:+\frac{\mathrm{1}}{\mathrm{2}}{t}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\:\right]_{\mathrm{1}} ^{\sqrt{\mathrm{3}}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left\{\:{ln}\left(\:\mathrm{2}+\sqrt{\mathrm{3}}\right)\:+\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\:.\mathrm{2}\:−{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)\:−\sqrt{\mathrm{2}}\:\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left\{\:{ln}\left(\mathrm{2}+\sqrt{\mathrm{3}}\right)\:−{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)\:+\sqrt{\mathrm{3}}\:−\sqrt{\mathrm{2}}\:\right\} \\ $$
Answered by MJS last updated on 14/May/18
![∫(√(1+t^2 ))dt= [t=tan(u) → dt=sec^2 (u)du] =∫sec^2 (u)(√(1+tan^2 (u)))du= =∫sec^3 (u)du= [∫sec^n (u)du=((sec^(n−2) (u)tan(u))/(n−1))+((n−2)/(n−1))∫sec^(n−2) (u)du] =((sec(u)tan(u))/2)+(1/2)∫sec(u)du= =((sec(u)tan(u))/2)+(1/2)∫sec(u)((tan(u)+sec(u))/(tan(u)+sec(u)))du= =((sec(u)tan(u))/2)+(1/2)∫((tan(u)sec(u)+sec^2 (u))/(tan(u)+sec(u)))du= [v=tan(u)+sec(u) → du=(dv/(tan(u)sec(u)+sec^2 (u)))] =((sec(u)tan(u))/2)+(1/2)∫(1/v)dv= =((sec(u)tan(u))/2)+((ln(v))/2)+C= =((sec(u)tan(u))/2)+((ln∣tan(u)+sec(u)∣)/2)+C= =((sec(arctan(t))tan(arcran(t)))/2)+((ln∣tan(arctan(t))+sec(arctan(t))∣)/2)+C= =((t(√(1+t^2 )))/2)+((ln∣t+(√(1+t^2 ))∣)/2)+C ∫_1 ^(√3) (√(1+t^2 ))dt=[((t(√(1+t^2 )))/2)+((ln∣t+(√(1+t^2 ))∣)/2)]_1 ^(√3) = =(√3)+((ln(2+(√3)))/2)−(((√2)/2)+((ln(1+(√2)))/2))= =(√3)−((√2)/2)+(1/2)ln(((2+(√3))/(1+(√2))))=(√3)−((√2)/2)+(1/2)ln((√6)−(√3)+2(√2)−2)≈ ≈1.242736](Q35063.png)
$$\int\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }{dt}= \\ $$$$\:\:\:\:\:\:\:\:\:\:\left[{t}=\mathrm{tan}\left({u}\right)\:\rightarrow\:{dt}=\mathrm{sec}^{\mathrm{2}} \left({u}\right){du}\right] \\ $$$$=\int\mathrm{sec}^{\mathrm{2}} \left({u}\right)\sqrt{\mathrm{1}+\mathrm{tan}^{\mathrm{2}} \left({u}\right)}{du}= \\ $$$$=\int\mathrm{sec}^{\mathrm{3}} \left({u}\right){du}= \\ $$$$\:\:\:\:\:\:\:\:\:\:\left[\int\mathrm{sec}^{{n}} \left({u}\right){du}=\frac{\mathrm{sec}^{{n}−\mathrm{2}} \left({u}\right)\mathrm{tan}\left({u}\right)}{{n}−\mathrm{1}}+\frac{{n}−\mathrm{2}}{{n}−\mathrm{1}}\int\mathrm{sec}^{{n}−\mathrm{2}} \left({u}\right){du}\right] \\ $$$$=\frac{\mathrm{sec}\left({u}\right)\mathrm{tan}\left({u}\right)}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\int\mathrm{sec}\left({u}\right){du}= \\ $$$$=\frac{\mathrm{sec}\left({u}\right)\mathrm{tan}\left({u}\right)}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\int\mathrm{sec}\left({u}\right)\frac{\mathrm{tan}\left({u}\right)+\mathrm{sec}\left({u}\right)}{\mathrm{tan}\left({u}\right)+\mathrm{sec}\left({u}\right)}{du}= \\ $$$$=\frac{\mathrm{sec}\left({u}\right)\mathrm{tan}\left({u}\right)}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{tan}\left({u}\right)\mathrm{sec}\left({u}\right)+\mathrm{sec}^{\mathrm{2}} \left({u}\right)}{\mathrm{tan}\left({u}\right)+\mathrm{sec}\left({u}\right)}{du}= \\ $$$$\:\:\:\:\:\:\:\:\:\:\left[{v}=\mathrm{tan}\left({u}\right)+\mathrm{sec}\left({u}\right)\:\rightarrow\:{du}=\frac{{dv}}{\mathrm{tan}\left({u}\right)\mathrm{sec}\left({u}\right)+\mathrm{sec}^{\mathrm{2}} \left({u}\right)}\right] \\ $$$$=\frac{\mathrm{sec}\left({u}\right)\mathrm{tan}\left({u}\right)}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{1}}{{v}}{dv}= \\ $$$$=\frac{\mathrm{sec}\left({u}\right)\mathrm{tan}\left({u}\right)}{\mathrm{2}}+\frac{\mathrm{ln}\left({v}\right)}{\mathrm{2}}+{C}= \\ $$$$=\frac{\mathrm{sec}\left({u}\right)\mathrm{tan}\left({u}\right)}{\mathrm{2}}+\frac{\mathrm{ln}\mid\mathrm{tan}\left({u}\right)+\mathrm{sec}\left({u}\right)\mid}{\mathrm{2}}+{C}= \\ $$$$=\frac{\mathrm{sec}\left(\mathrm{arctan}\left({t}\right)\right)\mathrm{tan}\left(\mathrm{arcran}\left({t}\right)\right)}{\mathrm{2}}+\frac{\mathrm{ln}\mid\mathrm{tan}\left(\mathrm{arctan}\left({t}\right)\right)+\mathrm{sec}\left(\mathrm{arctan}\left({t}\right)\right)\mid}{\mathrm{2}}+{C}= \\ $$$$=\frac{{t}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }}{\mathrm{2}}+\frac{\mathrm{ln}\mid{t}+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\mid}{\mathrm{2}}+{C} \\ $$$$ \\ $$$$\underset{\mathrm{1}} {\overset{\sqrt{\mathrm{3}}} {\int}}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }{dt}=\left[\frac{{t}\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }}{\mathrm{2}}+\frac{\mathrm{ln}\mid{t}+\sqrt{\mathrm{1}+{t}^{\mathrm{2}} }\mid}{\mathrm{2}}\right]_{\mathrm{1}} ^{\sqrt{\mathrm{3}}} = \\ $$$$=\sqrt{\mathrm{3}}+\frac{\mathrm{ln}\left(\mathrm{2}+\sqrt{\mathrm{3}}\right)}{\mathrm{2}}−\left(\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+\frac{\mathrm{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)}{\mathrm{2}}\right)= \\ $$$$=\sqrt{\mathrm{3}}−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\left(\frac{\mathrm{2}+\sqrt{\mathrm{3}}}{\mathrm{1}+\sqrt{\mathrm{2}}}\right)=\sqrt{\mathrm{3}}−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}+\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\left(\sqrt{\mathrm{6}}−\sqrt{\mathrm{3}}+\mathrm{2}\sqrt{\mathrm{2}}−\mathrm{2}\right)\approx \\ $$$$\approx\mathrm{1}.\mathrm{242736} \\ $$
Commented by math khazana by abdo last updated on 15/May/18
$${correct}\:{answer}\:{thanks}\:{sir}\:{Mjs} \\ $$