
Question and Answers Forum
Question Number 35297 by 123 45 polytechnicien last updated on 17/May/18
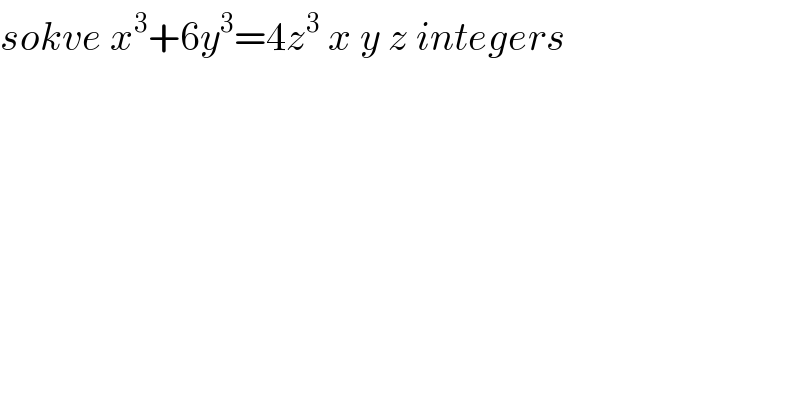
Commented by Rasheed.Sindhi last updated on 18/May/18
![4z^3 →even⇒x^3 +6y^3 →even x^3 +6y^3 →even ∧ 6y^3 →even ⇒x^3 →even⇒x→even Let x=2m x^3 +6y^3 =4z^3 ⇒8m^3 +6y^3 =4z^3 ⇒4m^3 +3y^3 =2z^3 4m^3 →even ∧ 2z^3 →even⇒3y^3 →even ⇒y^3 →even⇒y→even x^3 +6y^3 =4z^3 ⇒(even)^3 +6(even)^3 =4z^3 8∣(even)^3 ⇒8∣ [(even)^3 +6(even)^3 ] ⇒8∣4z^3 ⇒z^3 →even⇒z→even So x,y,z→even Let x=2m,y=2n & z=2l x^3 +6y^3 =4z^3 ⇒(2m)^3 +6(2n)^3 =4(2l)^3 8m^3 +6(8n^3 )=4(8l^3 ) m^3 +6n^3 =4l^3 This is same as x^3 +6y^3 =4z^3 (hahaha we reeched at the begning) Anyway we should search x,y,z in in even numbers and this is a clue!](Q35388.png)
| ||
Question and Answers Forum | ||
Question Number 35297 by 123 45 polytechnicien last updated on 17/May/18 | ||
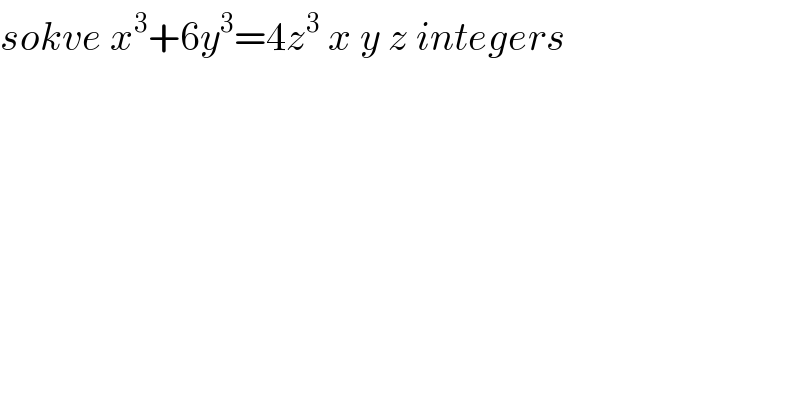 | ||
Commented by Rasheed.Sindhi last updated on 18/May/18 | ||
![4z^3 →even⇒x^3 +6y^3 →even x^3 +6y^3 →even ∧ 6y^3 →even ⇒x^3 →even⇒x→even Let x=2m x^3 +6y^3 =4z^3 ⇒8m^3 +6y^3 =4z^3 ⇒4m^3 +3y^3 =2z^3 4m^3 →even ∧ 2z^3 →even⇒3y^3 →even ⇒y^3 →even⇒y→even x^3 +6y^3 =4z^3 ⇒(even)^3 +6(even)^3 =4z^3 8∣(even)^3 ⇒8∣ [(even)^3 +6(even)^3 ] ⇒8∣4z^3 ⇒z^3 →even⇒z→even So x,y,z→even Let x=2m,y=2n & z=2l x^3 +6y^3 =4z^3 ⇒(2m)^3 +6(2n)^3 =4(2l)^3 8m^3 +6(8n^3 )=4(8l^3 ) m^3 +6n^3 =4l^3 This is same as x^3 +6y^3 =4z^3 (hahaha we reeched at the begning) Anyway we should search x,y,z in in even numbers and this is a clue!](Q35388.png) | ||