Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 35304 by Rio Mike last updated on 17/May/18

Q1. a) solve for x 9^x +5(3^x )=6 b)write down the first 4 terms in the binomial expansion of (1−3x)^7 c)the sum S_n of the first n^(th) terms is given by S_(n ) = 3(1−((2/3))^n ) find d) the common ratio e) the sum to infinity of the progression

$$\left.\:{Q}\mathrm{1}.\:\:\:{a}\right)\:{solve}\:{for}\:{x}\:\:\mathrm{9}^{{x}} +\mathrm{5}\left(\mathrm{3}^{{x}} \right)=\mathrm{6} \\ $$$$\left.{b}\right){write}\:{down}\:{the}\:{first}\:\:\mathrm{4}\:{terms} \\ $$$${in}\:{the}\:{binomial}\:{expansion}\:{of}\:\left(\mathrm{1}−\mathrm{3}{x}\right)^{\mathrm{7}} \\ $$$$\left.{c}\right){the}\:{sum}\:{S}_{{n}} \:{of}\:{the}\:{first}\:{n}^{{th}} {terms} \\ $$$${is}\:{given}\:{by}\:{S}_{{n}\:} =\:\mathrm{3}\left(\mathrm{1}−\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{{n}} \right)\:{find} \\ $$$$\left.{d}\right)\:{the}\:{common}\:{ratio} \\ $$$$\left.{e}\right)\:{the}\:{sum}\:{to}\:{infinity}\:{of}\:{the}\:{progression} \\ $$

Commented by prakash jain last updated on 17/May/18

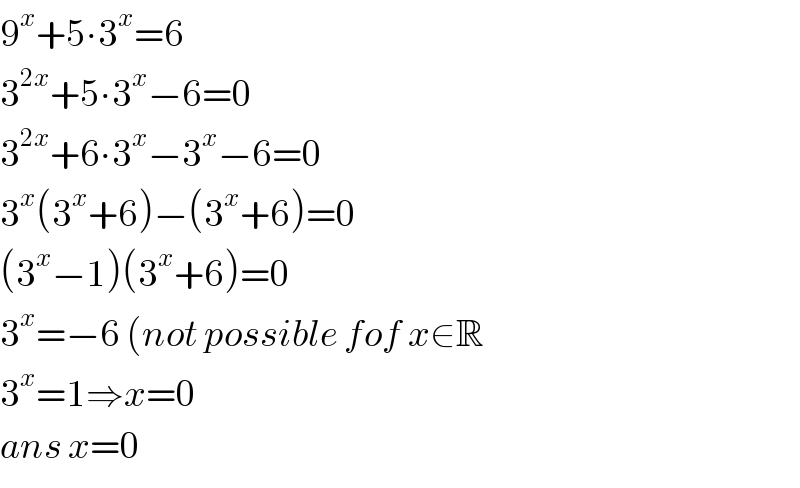
9^x +5∙3^x =6 3^(2x) +5∙3^x −6=0 3^(2x) +6∙3^x −3^x −6=0 3^x (3^x +6)−(3^x +6)=0 (3^x −1)(3^x +6)=0 3^x =−6 (not possible fof x∈R 3^x =1⇒x=0 ans x=0

$$\mathrm{9}^{{x}} +\mathrm{5}\centerdot\mathrm{3}^{{x}} =\mathrm{6} \\ $$$$\mathrm{3}^{\mathrm{2}{x}} +\mathrm{5}\centerdot\mathrm{3}^{{x}} −\mathrm{6}=\mathrm{0} \\ $$$$\mathrm{3}^{\mathrm{2}{x}} +\mathrm{6}\centerdot\mathrm{3}^{{x}} −\mathrm{3}^{{x}} −\mathrm{6}=\mathrm{0} \\ $$$$\mathrm{3}^{{x}} \left(\mathrm{3}^{{x}} +\mathrm{6}\right)−\left(\mathrm{3}^{{x}} +\mathrm{6}\right)=\mathrm{0} \\ $$$$\left(\mathrm{3}^{{x}} −\mathrm{1}\right)\left(\mathrm{3}^{{x}} +\mathrm{6}\right)=\mathrm{0} \\ $$$$\mathrm{3}^{{x}} =−\mathrm{6}\:\left({not}\:{possible}\:{fof}\:{x}\in\mathbb{R}\right. \\ $$$$\mathrm{3}^{{x}} =\mathrm{1}\Rightarrow{x}=\mathrm{0} \\ $$$${ans}\:{x}=\mathrm{0} \\ $$

Commented by Rasheed.Sindhi last updated on 18/May/18

Welcome back SIR! I haven′t seen your post for long time.Are you too busy nowadays?

$$\mathrm{Welcome}\:\mathrm{back}\:\mathrm{SIR}!\:\mathrm{I}\:\mathrm{haven}'\mathrm{t}\:\mathrm{seen}\:\mathrm{your} \\ $$$$\mathrm{post}\:\mathrm{for}\:\mathrm{long}\:\mathrm{time}.\mathrm{Are}\:\mathrm{you}\:\mathrm{too}\:\mathrm{busy}\: \\ $$$$\mathrm{nowadays}? \\ $$

Commented by rahul 19 last updated on 18/May/18

No problem sir ��

Commented by prakash jain last updated on 18/May/18

Was very busy in office. Could only read the post once in a week.

$$\mathrm{Was}\:\mathrm{very}\:\mathrm{busy}\:\mathrm{in}\:\mathrm{office}.\:\mathrm{Could} \\ $$$$\mathrm{only}\:\mathrm{read}\:\mathrm{the}\:\mathrm{post}\:\mathrm{once}\:\mathrm{in}\:\mathrm{a}\:\mathrm{week}. \\ $$

Answered by MJS last updated on 18/May/18

(3^x )^2 +5(3^x )−6=0 (3^x )=−(5/2)±(√(((25)/4)+6))=−(5/2)±(7/2) (3^x )=−6 ∨ (3^x )=1 x_2 =0 x_1 =((ln 6)/(ln 3))+(((2n+1)π)/(ln 3))i; n∈N_0 (a−b)^7 = ((7),(0) )×a^7 − ((7),(1) )×a^6 b+ ((7),(2) )×a^5 b^2 − ((7),(3) )×a^4 b^3 + ((7),(4) )×a^3 b^4 − ((7),(5) )×a^2 b^5 + ((7),(6) )×ab^6 − ((7),(7) )×ib^7 (1−3x)^7 =1−21x+189x^2 −945x^3 +2835x^4 −5103x^5 +5103x^6 −2187x^7 the ratio is (2/3) p_n =((2/3))^n p_n =q^n ⇒ S_n =((q^(n+1) −1)/(q−1)) S_∞ =(1/(1−q))=3

$$\left(\mathrm{3}^{{x}} \right)^{\mathrm{2}} +\mathrm{5}\left(\mathrm{3}^{{x}} \right)−\mathrm{6}=\mathrm{0} \\ $$$$\left(\mathrm{3}^{{x}} \right)=−\frac{\mathrm{5}}{\mathrm{2}}\pm\sqrt{\frac{\mathrm{25}}{\mathrm{4}}+\mathrm{6}}=−\frac{\mathrm{5}}{\mathrm{2}}\pm\frac{\mathrm{7}}{\mathrm{2}} \\ $$$$\left(\mathrm{3}^{{x}} \right)=−\mathrm{6}\:\vee\:\left(\mathrm{3}^{{x}} \right)=\mathrm{1} \\ $$$${x}_{\mathrm{2}} =\mathrm{0} \\ $$$${x}_{\mathrm{1}} =\frac{\mathrm{ln}\:\mathrm{6}}{\mathrm{ln}\:\mathrm{3}}+\frac{\left(\mathrm{2}{n}+\mathrm{1}\right)\pi}{\mathrm{ln}\:\mathrm{3}}\mathrm{i};\:{n}\in\mathbb{N}_{\mathrm{0}} \\ $$$$ \\ $$$$\left({a}−{b}\right)^{\mathrm{7}} =\begin{pmatrix}{\mathrm{7}}\\{\mathrm{0}}\end{pmatrix}×{a}^{\mathrm{7}} −\begin{pmatrix}{\mathrm{7}}\\{\mathrm{1}}\end{pmatrix}×{a}^{\mathrm{6}} {b}+\begin{pmatrix}{\mathrm{7}}\\{\mathrm{2}}\end{pmatrix}×{a}^{\mathrm{5}} {b}^{\mathrm{2}} −\begin{pmatrix}{\mathrm{7}}\\{\mathrm{3}}\end{pmatrix}×{a}^{\mathrm{4}} {b}^{\mathrm{3}} +\begin{pmatrix}{\mathrm{7}}\\{\mathrm{4}}\end{pmatrix}×{a}^{\mathrm{3}} {b}^{\mathrm{4}} −\begin{pmatrix}{\mathrm{7}}\\{\mathrm{5}}\end{pmatrix}×{a}^{\mathrm{2}} {b}^{\mathrm{5}} +\begin{pmatrix}{\mathrm{7}}\\{\mathrm{6}}\end{pmatrix}×{ab}^{\mathrm{6}} −\begin{pmatrix}{\mathrm{7}}\\{\mathrm{7}}\end{pmatrix}×{ib}^{\mathrm{7}} \\ $$$$\left(\mathrm{1}−\mathrm{3}{x}\right)^{\mathrm{7}} =\mathrm{1}−\mathrm{21}{x}+\mathrm{189}{x}^{\mathrm{2}} −\mathrm{945}{x}^{\mathrm{3}} +\mathrm{2835}{x}^{\mathrm{4}} −\mathrm{5103}{x}^{\mathrm{5}} +\mathrm{5103}{x}^{\mathrm{6}} −\mathrm{2187}{x}^{\mathrm{7}} \\ $$$$ \\ $$$$\mathrm{the}\:\mathrm{ratio}\:\mathrm{is}\:\frac{\mathrm{2}}{\mathrm{3}} \\ $$$${p}_{{n}} =\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{{n}} \\ $$$${p}_{{n}} ={q}^{{n}} \:\Rightarrow\:{S}_{{n}} =\frac{{q}^{{n}+\mathrm{1}} −\mathrm{1}}{{q}−\mathrm{1}} \\ $$$${S}_{\infty} =\frac{\mathrm{1}}{\mathrm{1}−{q}}=\mathrm{3} \\ $$

Answered by Rasheed.Sindhi last updated on 27/May/18

(c) S_n = 3(1−((2/3))^n ) First term=a=3(1−((2/3))^1 )=1 ⋇Common ratio r 1+r=(S_2 /S_1 )=((3(1−((2/3))^2 ))/(3(1−((2/3))^1 ))) [(S_2 /S_1 )=((a+ar)/a)=1+r] =(((1−(2/3))(1+(2/3)))/((1−(2/3))))=(5/3) r=(5/3)−1=(2/3) ⋇ Sum of infinite terms=(a/(1−r))=(1/(1−(2/3))) =(1/(1/3))=3

$$\left(\mathrm{c}\right)\:{S}_{\mathrm{n}} =\:\mathrm{3}\left(\mathrm{1}−\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{{n}} \right) \\ $$$$\:\:\mathrm{First}\:\mathrm{term}={a}=\mathrm{3}\left(\mathrm{1}−\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{1}} \right)=\mathrm{1} \\ $$$$\:\:\:\divideontimes\mathrm{Common}\:\mathrm{ratio}\:\:{r} \\ $$$$\mathrm{1}+{r}=\frac{{S}_{\mathrm{2}} }{{S}_{\mathrm{1}} }=\frac{\mathrm{3}\left(\mathrm{1}−\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{2}} \right)}{\mathrm{3}\left(\mathrm{1}−\left(\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{1}} \right)}\:\:\:\left[\frac{\mathrm{S}_{\mathrm{2}} }{\mathrm{S}_{\mathrm{1}} }=\frac{{a}+{ar}}{{a}}=\mathrm{1}+{r}\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\left(\mathrm{1}−\frac{\mathrm{2}}{\mathrm{3}}\right)\left(\mathrm{1}+\frac{\mathrm{2}}{\mathrm{3}}\right)}{\left(\mathrm{1}−\frac{\mathrm{2}}{\mathrm{3}}\right)}=\frac{\mathrm{5}}{\mathrm{3}} \\ $$$$\:\:\:\:\:\:\mathrm{r}=\frac{\mathrm{5}}{\mathrm{3}}−\mathrm{1}=\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$\:\:\:\:\divideontimes\:\mathrm{Sum}\:\mathrm{of}\:\mathrm{infinite}\:\mathrm{terms}=\frac{{a}}{\mathrm{1}−{r}}=\frac{\mathrm{1}}{\mathrm{1}−\frac{\mathrm{2}}{\mathrm{3}}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{\mathrm{1}/\mathrm{3}}=\mathrm{3} \\ $$

Commented by Rasheed.Sindhi last updated on 27/May/18

Corrected.

$$\mathrm{Corrected}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com