Math Question and Answers


Question and Answers Forum

All Questions Topic List
Electrostatics Questions
Previous in All Question Next in All Question
Previous in Electrostatics Next in Electrostatics
Question Number 35895 by ajfour last updated on 25/May/18

Commented by ajfour last updated on 25/May/18

$F i n d t h e e l e c t r i c f i e l d d u e t o a$ $u n i f o r m l y c h a r g e d e q u i l a t e r a l$ $t r i a n g u l a r p l a t e (o f c h a r g e$ $d e n s i t y σ) o n t h e y a x i s a t a$ $d i s t a n c e y f r o m o r i g i n (t h e$ $c e n t r o i d o f p l a t e) .$
	Answered by tanmay.chaudhury50@gmail.com last updated on 25/May/18

	$t h e e l e m e n t a r y a r e a a s p e r f i g u r e i s d x d z$ $f o r s i g n c o n v e n i e n c e l e t c o s i d e r t h e p o s i t i o n$ $c o o r i d i n a t e i s (x, z)$ $e l e c t r i c f i e l d a t a d i s t a n c e y a s p e r f i g u r e i s$ $d E = \frac{1}{4 Π ϵ_{0}} \times \frac{σ d x d y}{x^{2} + y^{2} + z^{2}}$ $n o w t o f i n d l i m i t o f x a n d z$ $c e n t r o i d d e v i d e h e i g h t o f t h e t r i a n g l e i n 2 : 1$ $s o d e v i d i n g \frac{\sqrt{3} a}{2} i n 2 : 1 r a t i o w e g e t t h e l i m i t$ $o f z u p p e r l i m i t = \frac{2}{3} \times \frac{\sqrt{3} a}{2}, l o w e r = \frac{1}{3} \times \frac{\sqrt{3} a}{2}$ $Z_{u p} = \frac{a}{\sqrt{3}} Z_{l o w} = \frac{a}{2 \sqrt{3}} l i m i t o f z f r o m \frac{a}{\sqrt{3}} t o (- \frac{a}{2 \sqrt{3}}$ $n o w t o f i n d l i m i t o f x \frac{2}{3} = \frac{x}{\frac{a}{2}}$ $x = \frac{a}{3} l i m i t o f x i s f r o m - \frac{a_{}}{3} t o + \frac{a}{3}$ $E = \frac{1}{4 Π ϵ_{0}} \times \int_{- \frac{a}{3}}^{\frac{a}{3}} \int_{- \frac{a}{2 \sqrt{3}}}^{+ \frac{a}{\sqrt{3}}} \frac{σ d x d z}{x^{2} + y^{2} + z^{2}}$ $g i v e m e t i m e . . . .$
	Commented by ajfour last updated on 25/May/18

	$n o t o k a y, y o u a r e i n e r r o r . .$
	Commented by tanmay.chaudhury50@gmail.com last updated on 25/May/18

	$t r u e$
	Commented by tanmay.chaudhury50@gmail.com last updated on 25/May/18

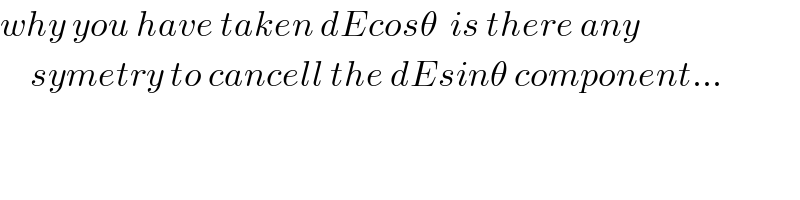
	$w h y y o u h a v e t a k e n d E c o s θ i s t h e r e a n y$ $s y m e t r y t o c a n c e l l t h e d E s i n θ c o m p o n e n t . . .$
	Commented by ajfour last updated on 25/May/18

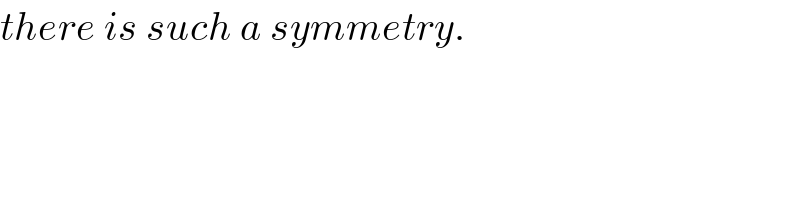
	$t h e r e i s s u c h a s y m m e t r y .$
	Answered by ajfour last updated on 25/May/18

	$d E = \frac{1}{4 π ϵ_{0}} (\frac{σ d x d z}{x^{2} + y^{2} + z^{2}})$ $E ∣_{(0, y, 0)} = \int \int d E \cos θ$ $= \frac{1}{4 π ϵ_{0}} \int_{- \frac{a}{2}}^{\frac{a}{2}} [\int_{- \frac{a}{2 \sqrt{3}}}^{(\frac{2}{3} \times \frac{a}{2} - x) \sqrt{3}} \frac{σ y d z}{{(x^{2} + y^{2} + z^{2})}^{3 / 2}}] d x$ $l e t z = \sqrt{x^{2} + y^{2}} \tan ϕ$ $d z = \sqrt{x^{2} + y^{2}} \sec^{2} ϕ d ϕ$ $E = \frac{σ y}{4 π ϵ_{0}} \int_{- \frac{a}{2}}^{\frac{a}{2}} [\int \frac{\sqrt{x^{2} + y^{2}}}{{(x^{2} + y^{2})}^{3 / 2} \sec^{3} ϕ} \sec^{2} ϕ d ϕ] d x$ $E = \frac{σ y}{4 π ϵ_{0}} \int_{- \frac{a}{2}}^{\frac{a}{2}} [\int \frac{\cos ϕ d ϕ}{(x^{2} + y^{2})}] d x$ $= \frac{σ y}{4 π ϵ_{0}} \int_{- \frac{a}{2}}^{\frac{a}{2}} \frac{1}{(x^{2} + y^{2})} {[\frac{z}{\sqrt{x^{2} + y^{2} + z^{2}}}]}_{- \frac{a}{2 \sqrt{3}}}^{(\frac{a}{3} - x) \sqrt{3}} d x$ $= \frac{σ y}{4 π ϵ_{0}} \int_{- \frac{a}{2}}^{\frac{a}{2}} \frac{1}{(x^{2} + y^{2})} [\frac{(\frac{a}{3} - x) \sqrt{3}}{\sqrt{x^{2} + y^{2} + 3 {(\frac{a}{3} - x)}^{2}}} + \frac{(\frac{a}{2 \sqrt{3}})}{\sqrt{x^{2} + y^{2} + \frac{a^{2}}{12}}}] d x$ $= \frac{σ y}{4 π ϵ_{0}} \int_{- \frac{a}{2}}^{\frac{a}{2}} [\frac{(\frac{a}{3} - x) \sqrt{3}}{(x^{2} + y^{2}) \sqrt{4 x^{2} - 2 a x + \frac{a^{2}}{3} + y^{2}}} + \frac{a}{2 \sqrt{3}} \times \frac{1}{(x^{2} + y^{2}) \sqrt{x^{2} + y^{2} + \frac{a^{2}}{12}}}] d x$ $= \frac{σ y}{4 π ϵ_{0}} \int_{- \frac{a}{2}}^{\frac{a}{2}} [\frac{(\frac{a}{3} - x) \sqrt{3}}{(x^{2} + y^{2}) \sqrt{{(2 x - \frac{a}{2})}^{2} + \frac{a^{2}}{12} + y^{2}}} + \frac{a}{2 \sqrt{3}} \times \frac{1}{(x^{2} + y^{2}) \sqrt{x^{2} + y^{2} + \frac{a^{2}}{12}}}] d x$ $. . . . .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum