
Question and Answers Forum
Question Number 363 by rajabhay last updated on 25/Jan/15
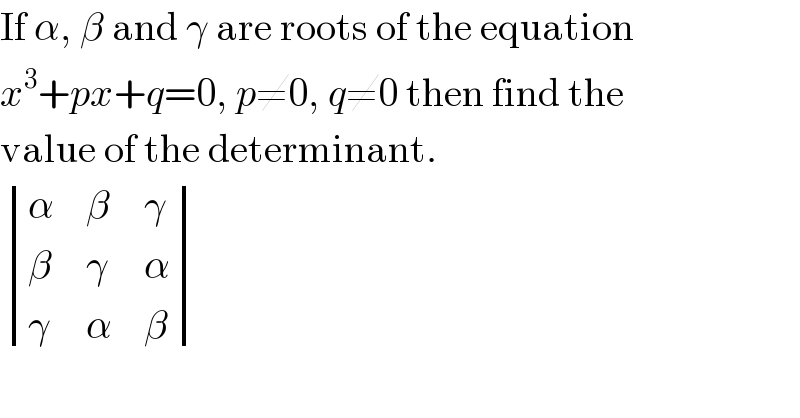
Commented by 123456 last updated on 24/Dec/14
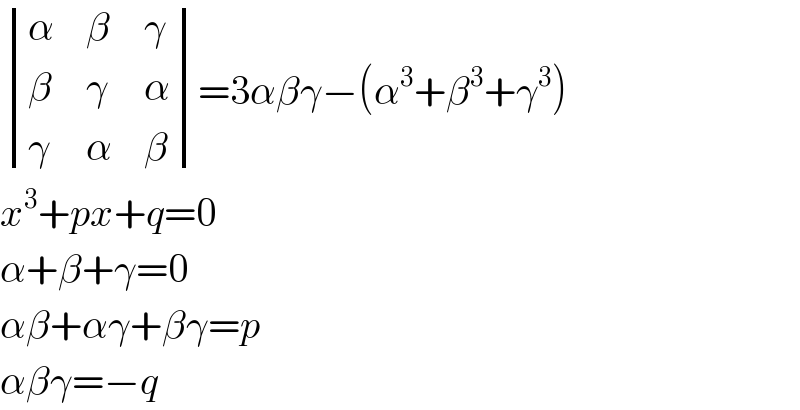
Answered by prakash jain last updated on 24/Dec/14

| ||
Question and Answers Forum | ||
Question Number 363 by rajabhay last updated on 25/Jan/15 | ||
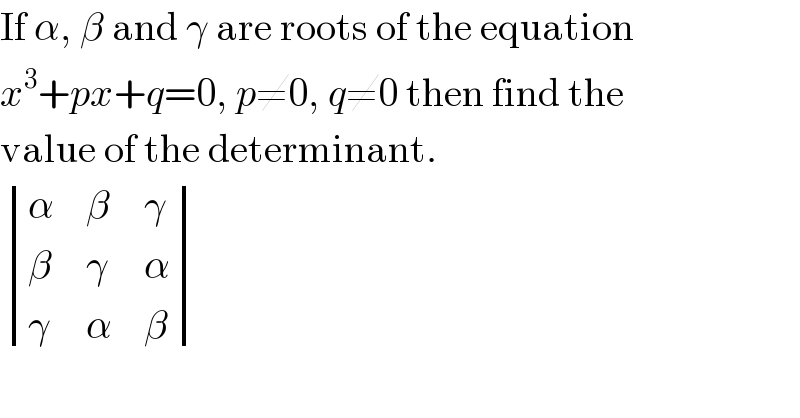 | ||
Commented by 123456 last updated on 24/Dec/14 | ||
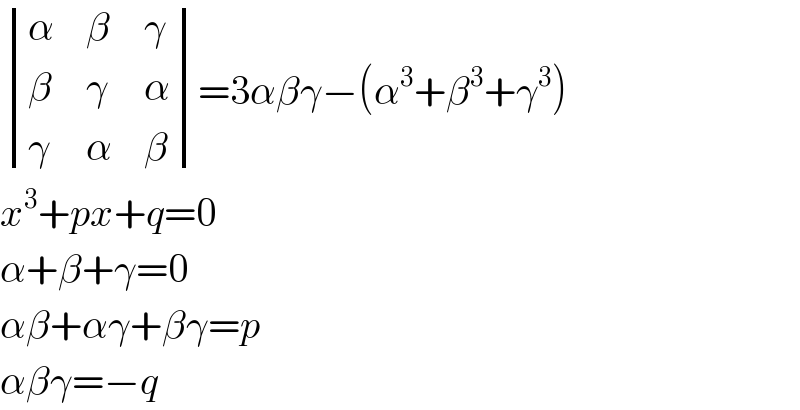 | ||
Answered by prakash jain last updated on 24/Dec/14 | ||
 | ||
| ||