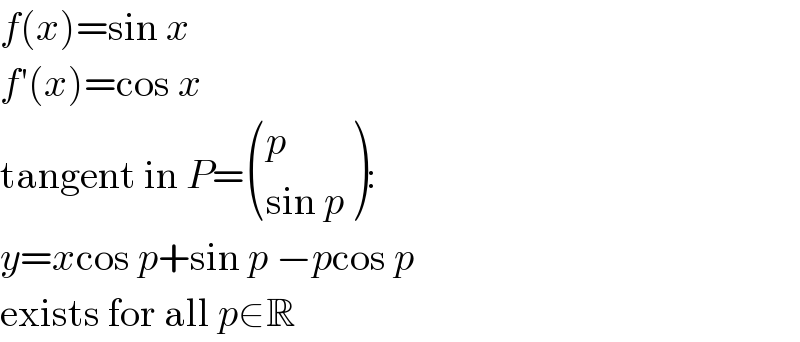Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 36703 by saikiran last updated on 04/Jun/18

$$showthatf\left(x\right)=\sin Xisderivableateverya\epsilon R$$

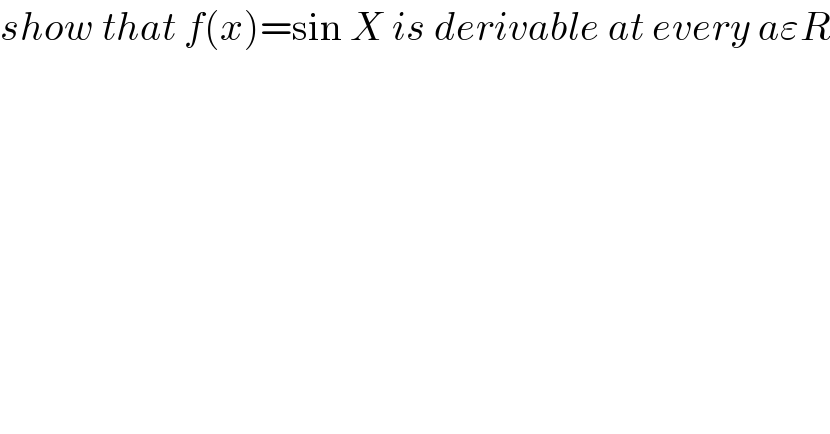
Commented by abdo mathsup 649 cc last updated on 04/Jun/18

$$wehaveforallx\in R$$$${lim}_{h\to 0}\frac{f(x+h)-f\left(x\right)}{h}={lim}_{h\to 0}\frac{sin(x+h)-sinx}{h}$$$$={lim}_{h\to 0}\frac{sinxcosh+cosxsinh-sinx}{h}$$$$={lim}_{h\to 0}\frac{-sinx(1-cosh)}{h}+\frac{sinh}{h}cosxbut$$$$weknowthat{lim}_{h\to 0}\frac{1-cosh}{h^2}=\frac{1}{2}\Rightarrow$$$${lim}_{h\to 0}\frac{1-cosh}{h}=0also{lim}_{h\to 0}\frac{sinh}{h}=1so$$$${lim}_{h\to 0}\frac{f(x+h)-f\left(x\right)}{h}=cosx(\in R)sofis$$$$derivablabeateverypointofR.$$

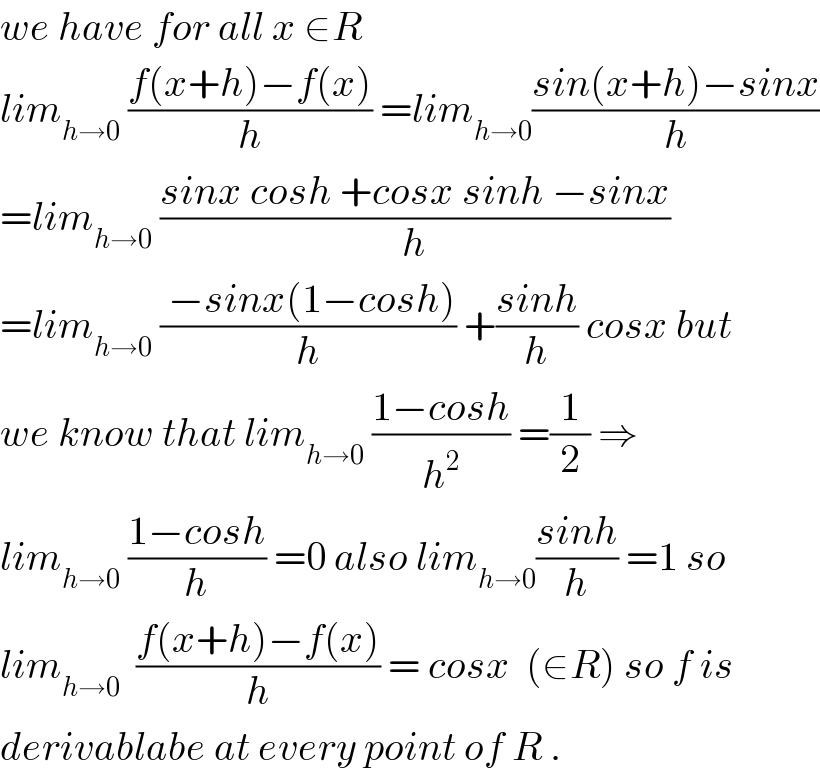
Commented by MJS last updated on 04/Jun/18

$$\mathrm{to}\mathrm{show}$$$$\underset{h\to 0}{\lim }\frac{\sin (x+h)-\sin (x-h)}{2h}=\cos x$$$$\mathrm{we}\mathrm{can}\mathrm{use}\sin ==\frac{{\mathrm{e}}^{\mathrm{i}x}-{\mathrm{e}}^{-\mathrm{i}x}}{2\mathrm{i}};\cos x=\frac{{\mathrm{e}}^{\mathrm{i}x}+{\mathrm{e}}^{-\mathrm{i}x}}{2}$$$$\underset{h\to 0}{\lim }\frac{{\mathrm{e}}^{\mathrm{i}(x+h)}-{\mathrm{e}}^{-\mathrm{i}(x+h)}-{\mathrm{e}}^{\mathrm{i}(x-h)}+{\mathrm{e}}^{-\mathrm{i}(x-h)}}{4h\mathrm{i}}=$$$$=\underset{h\to 0}{\lim }\frac{\frac{d}{dh}({\mathrm{e}}^{\mathrm{i}(x+h)}-{\mathrm{e}}^{-\mathrm{i}(x+h)}-{\mathrm{e}}^{\mathrm{i}(x-h)}+{\mathrm{e}}^{-\mathrm{i}(x-h)})}{\frac{d}{dh}\left(4h\mathrm{i}\right)}=$$$$=\underset{h\to 0}{\lim }\frac{\mathrm{i}}{4\mathrm{i}}({\mathrm{e}}^{\mathrm{i}(x+h)}+{\mathrm{e}}^{-\mathrm{i}(x+h)}+{\mathrm{e}}^{\mathrm{i}(x-h)}+{\mathrm{e}}^{-\mathrm{i}(x-h)})=$$$$=\frac{1}{4}({2\mathrm{e}}^{\mathrm{i}x}+{2\mathrm{e}}^{-\mathrm{i}x})=\frac{{\mathrm{e}}^{\mathrm{i}x}+{\mathrm{e}}^{-\mathrm{i}x}}{2}=\cos x$$

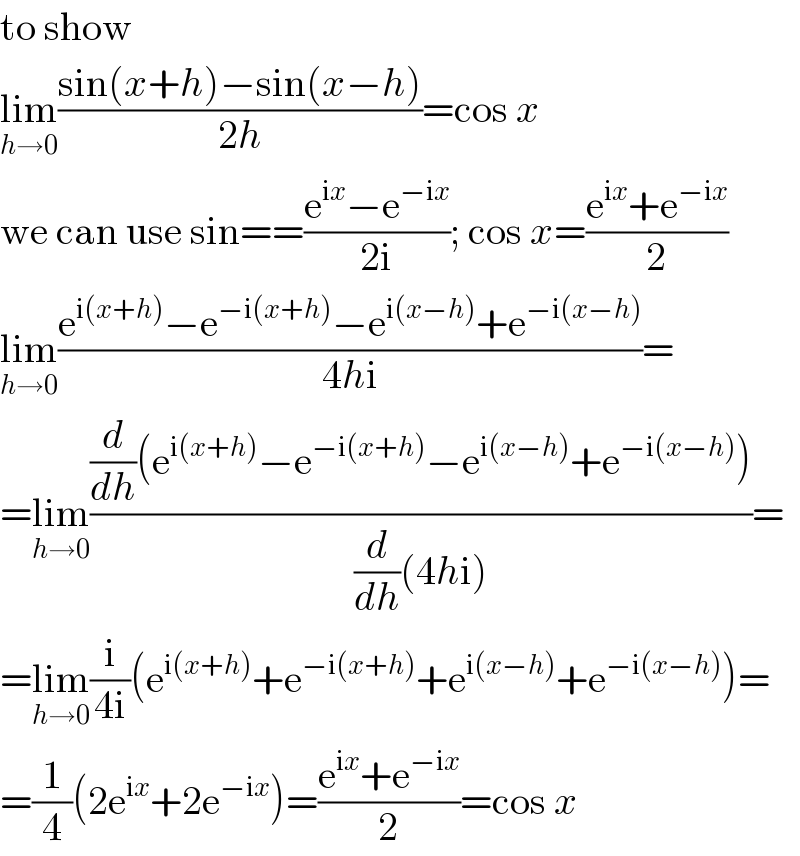
Answered by tanmay.chaudhury50@gmail.com last updated on 04/Jun/18

$$1)meaningofderivativeistheabilitytodraw$$$$tangentatapointonacurve.$$$$2)sinxisacontinuouscurveateverypoint$$$$andtangentcanbedrawnateveryoint.soit$$$$isderivable$$$$$$

Answered by MJS last updated on 04/Jun/18

$$f\left(x\right)=\sin x$$$$f^{\prime }\left(x\right)=\cos x$$$$\mathrm{tangent}\mathrm{in}P=\left(\begin{array}{c}p\\ \sin p\end{array}\right):$$$$y=x\cos p+\sin p-p\cos p$$$$\mathrm{exists}\mathrm{for}\mathrm{all}p\in \mathbb{R}$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com