Question Number 37071 by math khazana by abdo last updated on 08/Jun/18
$${find}\:{the}\:{value}\:{of}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\:\:\frac{{xdx}}{\mathrm{1}+{cosx}} \\ $$
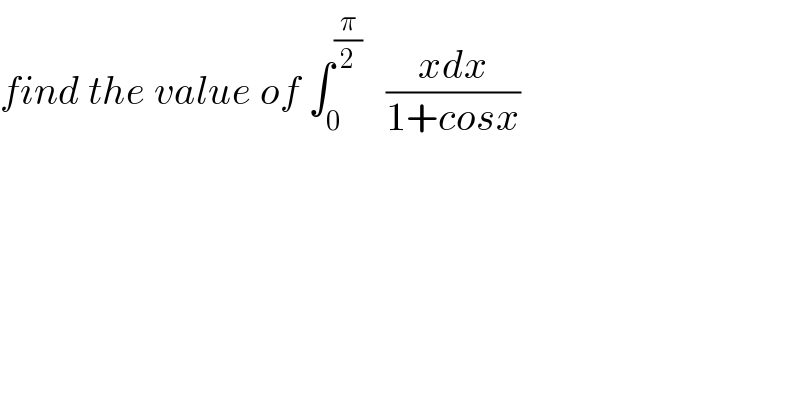
Commented by math khazana by abdo last updated on 10/Jun/18
![changement tan((x/2))=t give I = ∫_0 ^1 ((2arctan(t))/(1+((1−t^2 )/(1+t^2 )))) ((2dt)/(1+t^2 )) =4 ∫_0 ^1 ((arctan(t))/(1+t^2 +1−t^2 ))dt =2 ∫_0 ^1 arctan(t)dt and by parts I =2 { [t arctan(t)]_0 ^1 −∫_0 ^1 (t/(1+t^2 ))dt} =2{ (π/4) −(1/2)[ln(1+t^2 )]_0 ^1 } =(π/2) −ln(2) ★ I =(π/2) −ln(2)★](Q37169.png)
$${changement}\:{tan}\left(\frac{{x}}{\mathrm{2}}\right)={t}\:{give}\: \\ $$$${I}\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{\mathrm{2}{arctan}\left({t}\right)}{\mathrm{1}+\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }}\:\:\frac{\mathrm{2}{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }\:=\mathrm{4}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{arctan}\left({t}\right)}{\mathrm{1}+{t}^{\mathrm{2}} \:+\mathrm{1}−{t}^{\mathrm{2}} }{dt} \\ $$$$=\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:{arctan}\left({t}\right){dt}\:{and}\:{by}\:{parts} \\ $$$${I}\:=\mathrm{2}\:\left\{\:\left[{t}\:{arctan}\left({t}\right)\right]_{\mathrm{0}} ^{\mathrm{1}} \:\:−\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{t}}{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\right\} \\ $$$$=\mathrm{2}\left\{\:\frac{\pi}{\mathrm{4}}\:\:−\frac{\mathrm{1}}{\mathrm{2}}\left[{ln}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\right]_{\mathrm{0}} ^{\mathrm{1}} \right\} \\ $$$$=\frac{\pi}{\mathrm{2}}\:−{ln}\left(\mathrm{2}\right) \\ $$$$\bigstar\:{I}\:=\frac{\pi}{\mathrm{2}}\:−{ln}\left(\mathrm{2}\right)\bigstar \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 09/Jun/18
![∫_0 ^(Π/2) (x/(2cos^2 (x/2)))dx (1/2)∫_0 ^(Π/2) ((xsec^2 (x/2))/)dx let I_1 =∫xsec^2 (x/2)dx =x∫sec^2 (x/2)dx−∫[(dx/dx)∫sec^2 (x/2)dx]dx =x((tan(x/2))/(1/2))−∫((tan(x/2))/(1/2))dx =x×2tan(x/2)−4ln∣sec(x/2)∣ so required ans is (1/2){2xtan(x/2)−4ln∣sec(x/2)∣}_0 ^(Π/2) =(1/2)[{2×(Π/2)×1−4ln((√2) )}−{2×0×0−4ln1}] =(Π/2)−2×(1/2)ln2 =(Π/2)−ln2](Q37107.png)
$$\int_{\mathrm{0}} ^{\frac{\Pi}{\mathrm{2}}} \frac{{x}}{\mathrm{2}{cos}^{\mathrm{2}} \frac{{x}}{\mathrm{2}}}{dx} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\frac{\Pi}{\mathrm{2}}} \frac{{xsec}^{\mathrm{2}} \frac{{x}}{\mathrm{2}}}{}{dx} \\ $$$${let}\:{I}_{\mathrm{1}} =\int{xsec}^{\mathrm{2}} \frac{{x}}{\mathrm{2}}{dx} \\ $$$$={x}\int{sec}^{\mathrm{2}} \frac{{x}}{\mathrm{2}}{dx}−\int\left[\frac{{dx}}{{dx}}\int{sec}^{\mathrm{2}} \frac{{x}}{\mathrm{2}}{dx}\right]{dx} \\ $$$$={x}\frac{{tan}\frac{{x}}{\mathrm{2}}}{\frac{\mathrm{1}}{\mathrm{2}}}−\int\frac{{tan}\frac{{x}}{\mathrm{2}}}{\frac{\mathrm{1}}{\mathrm{2}}}{dx}\: \\ $$$$={x}×\mathrm{2}{tan}\frac{{x}}{\mathrm{2}}−\mathrm{4}{ln}\mid{sec}\frac{{x}}{\mathrm{2}}\mid \\ $$$${so}\:{required}\:{ans}\:{is} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\left\{\mathrm{2}{xtan}\frac{{x}}{\mathrm{2}}−\mathrm{4}{ln}\mid{sec}\frac{{x}}{\mathrm{2}}\mid\right\}_{\mathrm{0}} ^{\frac{\Pi}{\mathrm{2}}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left[\left\{\mathrm{2}×\frac{\Pi}{\mathrm{2}}×\mathrm{1}−\mathrm{4}{ln}\left(\sqrt{\mathrm{2}}\:\right)\right\}−\left\{\mathrm{2}×\mathrm{0}×\mathrm{0}−\mathrm{4}{ln}\mathrm{1}\right\}\right] \\ $$$$=\frac{\Pi}{\mathrm{2}}−\mathrm{2}×\frac{\mathrm{1}}{\mathrm{2}}{ln}\mathrm{2} \\ $$$$=\frac{\Pi}{\mathrm{2}}−{ln}\mathrm{2} \\ $$
Commented by math khazana by abdo last updated on 10/Jun/18
$${correct}\:{answer}\:{thanks}\:{sir}\:{Tanmay}. \\ $$
Answered by MJS last updated on 10/Jun/18
![∫(x/(1+cos x))dx= [((∫u′v=uv−∫uv′)),((u′=(1/(1+cos x)) ⇒ u=((sin x)/(1+cos x)))),(( [((u=∫(dx/(1+cos x))=∫((tan (x/2))/(sin x))dx=)),(( [t=(x/2) → dx=2dt])),((=2∫((tan t)/(sin 2t))dt=∫((tan t)/(sin t cos t))dt=∫sec^2 t dt=)),((=tan t=tan (x/2)=((sin x)/(1+cos x)))) ])),((v=x ⇒ v′=1)) ] =((xsin x)/(1+cos x))−∫((sin x)/(1+cos x))dx= [t=1+cos x → dx=−(dt/(sin x))] =((xsin x)/(1+cos x))+∫(dt/t)=((xsin x)/(1+cos x))+ln t= =((xsin x)/(1+cos x))+ln(1+cos x)+C](Q37197.png)
$$\int\frac{{x}}{\mathrm{1}+\mathrm{cos}\:{x}}{dx}= \\ $$$$\:\:\:\:\:\begin{bmatrix}{\int{u}'{v}={uv}−\int{uv}'}\\{{u}'=\frac{\mathrm{1}}{\mathrm{1}+\mathrm{cos}\:{x}}\:\Rightarrow\:{u}=\frac{\mathrm{sin}\:{x}}{\mathrm{1}+\mathrm{cos}\:{x}}}\\{\:\:\:\:\:\begin{bmatrix}{{u}=\int\frac{{dx}}{\mathrm{1}+\mathrm{cos}\:{x}}=\int\frac{\mathrm{tan}\:\frac{{x}}{\mathrm{2}}}{\mathrm{sin}\:{x}}{dx}=}\\{\:\:\:\:\:\left[{t}=\frac{{x}}{\mathrm{2}}\:\rightarrow\:{dx}=\mathrm{2}{dt}\right]}\\{=\mathrm{2}\int\frac{\mathrm{tan}\:{t}}{\mathrm{sin}\:\mathrm{2}{t}}{dt}=\int\frac{\mathrm{tan}\:{t}}{\mathrm{sin}\:{t}\:\mathrm{cos}\:{t}}{dt}=\int\mathrm{sec}^{\mathrm{2}} \:{t}\:{dt}=}\\{=\mathrm{tan}\:{t}=\mathrm{tan}\:\frac{{x}}{\mathrm{2}}=\frac{\mathrm{sin}\:{x}}{\mathrm{1}+\mathrm{cos}\:{x}}}\end{bmatrix}}\\{{v}={x}\:\Rightarrow\:{v}'=\mathrm{1}}\end{bmatrix} \\ $$$$=\frac{{x}\mathrm{sin}\:{x}}{\mathrm{1}+\mathrm{cos}\:{x}}−\int\frac{\mathrm{sin}\:{x}}{\mathrm{1}+\mathrm{cos}\:{x}}{dx}= \\ $$$$\:\:\:\:\:\left[{t}=\mathrm{1}+\mathrm{cos}\:{x}\:\rightarrow\:{dx}=−\frac{{dt}}{\mathrm{sin}\:{x}}\right] \\ $$$$=\frac{{x}\mathrm{sin}\:{x}}{\mathrm{1}+\mathrm{cos}\:{x}}+\int\frac{{dt}}{{t}}=\frac{{x}\mathrm{sin}\:{x}}{\mathrm{1}+\mathrm{cos}\:{x}}+\mathrm{ln}\:{t}= \\ $$$$=\frac{{x}\mathrm{sin}\:{x}}{\mathrm{1}+\mathrm{cos}\:{x}}+\mathrm{ln}\left(\mathrm{1}+\mathrm{cos}\:{x}\right)+{C} \\ $$$$ \\ $$