Question and Answers Forum

All Questions      Topic List

Relation and Functions Questions

Previous in All Question      Next in All Question      

Previous in Relation and Functions      Next in Relation and Functions      

Question Number 37282 by abdo.msup.com last updated on 11/Jun/18

let f(x)=(x/(1+x^2 +x^4 )) 1) find f^((n)) (x) 2)calculate f^((n)) (0) 3)developp f at integr serie.

$${let}\:{f}\left({x}\right)=\frac{{x}}{\mathrm{1}+{x}^{\mathrm{2}} \:+{x}^{\mathrm{4}} } \\ $$$$\left.\mathrm{1}\right)\:{find}\:{f}^{\left({n}\right)} \left({x}\right) \\ $$$$\left.\mathrm{2}\right){calculate}\:{f}^{\left({n}\right)} \left(\mathrm{0}\right) \\ $$$$\left.\mathrm{3}\right){developp}\:{f}\:{at}\:{integr}\:{serie}. \\ $$

Commented by abdo.msup.com last updated on 17/Jun/18

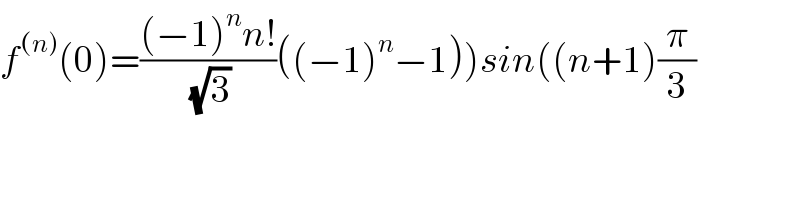
f^((n)) (0)=(((−1)^n n!)/(√3))((−1)^n −1))sin((n+1)(π/3)

$$\left.{f}^{\left({n}\right)} \left(\mathrm{0}\right)=\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\sqrt{\mathrm{3}}}\left(\left(−\mathrm{1}\right)^{{n}} −\mathrm{1}\right)\right){sin}\left(\left({n}+\mathrm{1}\right)\frac{\pi}{\mathrm{3}}\right. \\ $$

Commented by abdo.msup.com last updated on 17/Jun/18

⇒f^((2n)) (0)=0 and f^((2n+1)) (0)= ((2(2n+1)!)/(√3))sin{(2n+2)(π/3)} 3)f(x)=Σ_(n=0) ^∞ ((f^((n)) (0))/(n!)) x^n =Σ_(n=0) ^∞ ((f^((2n+1)) (0))/((2n+1)!)) x^(2n+1) =Σ_(n=0) ^∞ (2/(√3))sin{(2n+2)(π/3)}x^(2n+1) .

$$\Rightarrow{f}^{\left(\mathrm{2}{n}\right)} \left(\mathrm{0}\right)=\mathrm{0}\:{and}\: \\ $$$${f}^{\left(\mathrm{2}{n}+\mathrm{1}\right)} \left(\mathrm{0}\right)=\:\frac{\mathrm{2}\left(\mathrm{2}{n}+\mathrm{1}\right)!}{\sqrt{\mathrm{3}}}{sin}\left\{\left(\mathrm{2}{n}+\mathrm{2}\right)\frac{\pi}{\mathrm{3}}\right\} \\ $$$$\left.\mathrm{3}\right){f}\left({x}\right)=\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{{f}^{\left({n}\right)} \left(\mathrm{0}\right)}{{n}!}\:{x}^{{n}} \\ $$$$=\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{{f}^{\left(\mathrm{2}{n}+\mathrm{1}\right)} \left(\mathrm{0}\right)}{\left(\mathrm{2}{n}+\mathrm{1}\right)!}\:{x}^{\mathrm{2}{n}+\mathrm{1}} \\ $$$$=\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{2}}{\sqrt{\mathrm{3}}}{sin}\left\{\left(\mathrm{2}{n}+\mathrm{2}\right)\frac{\pi}{\mathrm{3}}\right\}{x}^{\mathrm{2}{n}+\mathrm{1}} \:. \\ $$

Commented by prof Abdo imad last updated on 17/Jun/18

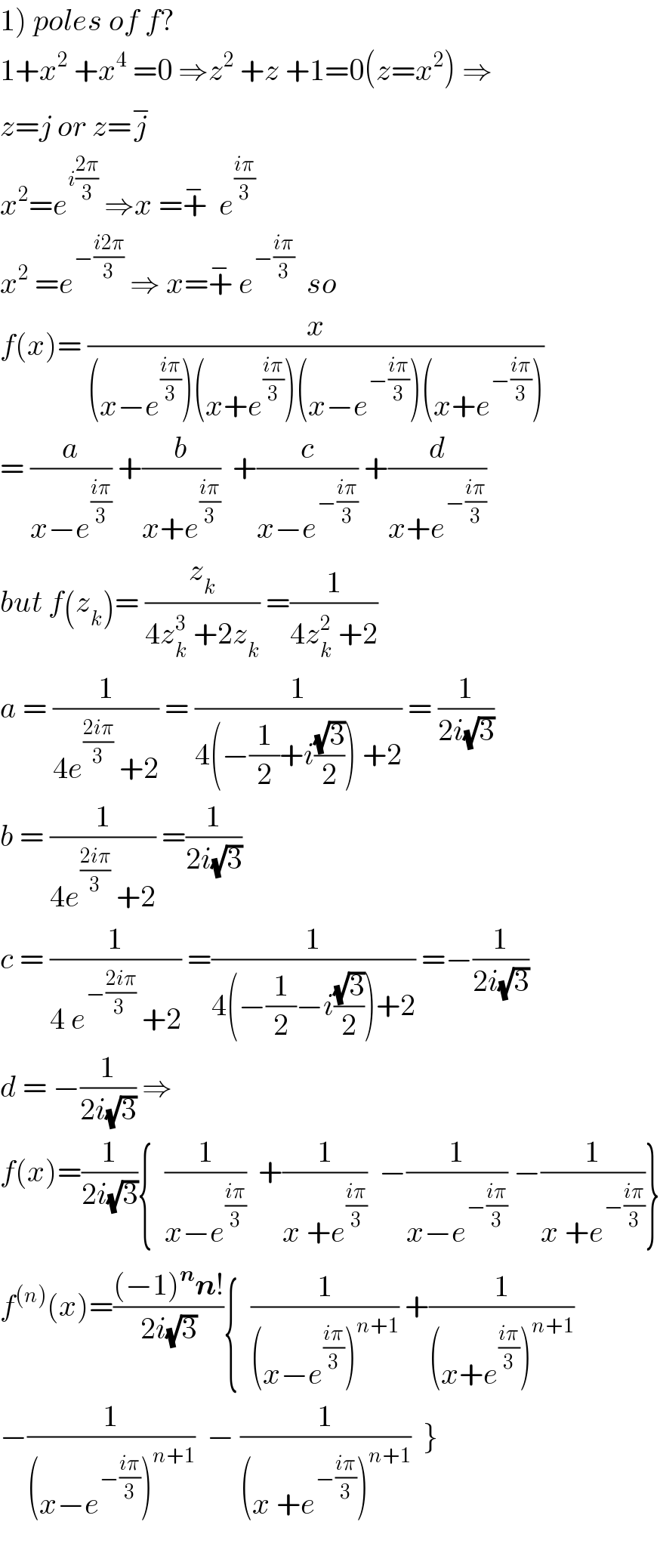
1) poles of f? 1+x^2 +x^4 =0 ⇒z^2 +z +1=0(z=x^2 ) ⇒ z=j or z=j^− x^2 =e^(i((2π)/3)) ⇒x =+^− e^((iπ)/3) x^2 =e^(−((i2π)/3)) ⇒ x=+^− e^(−((iπ)/3)) so f(x)= (x/((x−e^((iπ)/3) )(x+e^((iπ)/3) )(x−e^(−((iπ)/3)) )(x+e^(−((iπ)/3)) ))) = (a/(x−e^((iπ)/3) )) +(b/(x+e^((iπ)/3) )) +(c/(x−e^(−((iπ)/3)) )) +(d/(x+e^(−((iπ)/3)) )) but f(z_k )= (z_k /(4z_k ^3 +2z_k )) =(1/(4z_k ^2 +2)) a = (1/(4e^((2iπ)/3) +2)) = (1/(4(−(1/2)+i((√3)/2)) +2)) = (1/(2i(√3))) b = (1/(4e^((2iπ)/3) +2)) =(1/(2i(√3))) c = (1/(4 e^(−((2iπ)/(3 ))) +2)) =(1/(4(−(1/2)−i((√3)/2))+2)) =−(1/(2i(√3))) d = −(1/(2i(√3))) ⇒ f(x)=(1/(2i(√3))){ (1/(x−e^((iπ)/3) )) +(1/(x +e^((iπ)/3) )) −(1/(x−e^(−((iπ)/3)) )) −(1/(x +e^(−((iπ)/3)) ))} f^((n)) (x)=(((−1)^n n!)/(2i(√3))){ (1/((x−e^((iπ)/3) )^(n+1) )) +(1/((x+e^((iπ)/3) )^(n+1) )) −(1/((x−e^(−((iπ)/3)) )^(n+1) )) − (1/((x +e^(−((iπ)/3)) )^(n+1) )) }

$$\left.\mathrm{1}\right)\:{poles}\:{of}\:{f}? \\ $$$$\mathrm{1}+{x}^{\mathrm{2}} \:+{x}^{\mathrm{4}} \:=\mathrm{0}\:\Rightarrow{z}^{\mathrm{2}} \:+{z}\:+\mathrm{1}=\mathrm{0}\left({z}={x}^{\mathrm{2}} \right)\:\Rightarrow \\ $$$${z}={j}\:{or}\:{z}=\overset{−} {{j}} \\ $$$${x}^{\mathrm{2}} ={e}^{{i}\frac{\mathrm{2}\pi}{\mathrm{3}}} \:\Rightarrow{x}\:=\overset{−} {+}\:\:{e}^{\frac{{i}\pi}{\mathrm{3}}} \\ $$$${x}^{\mathrm{2}} \:={e}^{−\frac{{i}\mathrm{2}\pi}{\mathrm{3}}} \:\Rightarrow\:{x}=\overset{−} {+}\:{e}^{−\frac{{i}\pi}{\mathrm{3}}} \:\:{so} \\ $$$${f}\left({x}\right)=\:\frac{{x}}{\left({x}−{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)\left({x}+{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)\left({x}−{e}^{−\frac{{i}\pi}{\mathrm{3}}} \right)\left({x}+{e}^{−\frac{{i}\pi}{\mathrm{3}}} \right)} \\ $$$$=\:\frac{{a}}{{x}−{e}^{\frac{{i}\pi}{\mathrm{3}}} }\:+\frac{{b}}{{x}+{e}^{\frac{{i}\pi}{\mathrm{3}}} }\:\:+\frac{{c}}{{x}−{e}^{−\frac{{i}\pi}{\mathrm{3}}} }\:+\frac{{d}}{{x}+{e}^{−\frac{{i}\pi}{\mathrm{3}}} } \\ $$$${but}\:{f}\left({z}_{{k}} \right)=\:\frac{{z}_{{k}} }{\mathrm{4}{z}_{{k}} ^{\mathrm{3}} \:+\mathrm{2}{z}_{{k}} }\:=\frac{\mathrm{1}}{\mathrm{4}{z}_{{k}} ^{\mathrm{2}} \:+\mathrm{2}} \\ $$$${a}\:=\:\frac{\mathrm{1}}{\mathrm{4}{e}^{\frac{\mathrm{2}{i}\pi}{\mathrm{3}}} \:+\mathrm{2}}\:=\:\frac{\mathrm{1}}{\mathrm{4}\left(−\frac{\mathrm{1}}{\mathrm{2}}+{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)\:+\mathrm{2}}\:=\:\frac{\mathrm{1}}{\mathrm{2}{i}\sqrt{\mathrm{3}}} \\ $$$${b}\:=\:\frac{\mathrm{1}}{\mathrm{4}{e}^{\frac{\mathrm{2}{i}\pi}{\mathrm{3}}} \:+\mathrm{2}}\:=\frac{\mathrm{1}}{\mathrm{2}{i}\sqrt{\mathrm{3}}} \\ $$$${c}\:=\:\frac{\mathrm{1}}{\mathrm{4}\:{e}^{−\frac{\mathrm{2}{i}\pi}{\mathrm{3}\:}} \:+\mathrm{2}}\:=\frac{\mathrm{1}}{\mathrm{4}\left(−\frac{\mathrm{1}}{\mathrm{2}}−{i}\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}\right)+\mathrm{2}}\:=−\frac{\mathrm{1}}{\mathrm{2}{i}\sqrt{\mathrm{3}}} \\ $$$${d}\:=\:−\frac{\mathrm{1}}{\mathrm{2}{i}\sqrt{\mathrm{3}}}\:\Rightarrow \\ $$$${f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{2}{i}\sqrt{\mathrm{3}}}\left\{\:\:\frac{\mathrm{1}}{{x}−{e}^{\frac{{i}\pi}{\mathrm{3}}} }\:\:+\frac{\mathrm{1}}{{x}\:+{e}^{\frac{{i}\pi}{\mathrm{3}}} }\:\:−\frac{\mathrm{1}}{{x}−{e}^{−\frac{{i}\pi}{\mathrm{3}}} }\:−\frac{\mathrm{1}}{{x}\:+{e}^{−\frac{{i}\pi}{\mathrm{3}}} }\right\} \\ $$$${f}^{\left({n}\right)} \left({x}\right)=\frac{\left(−\mathrm{1}\right)^{\boldsymbol{{n}}} \boldsymbol{{n}}!}{\mathrm{2}{i}\sqrt{\mathrm{3}}}\left\{\:\:\frac{\mathrm{1}}{\left({x}−{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)^{{n}+\mathrm{1}} }\:+\frac{\mathrm{1}}{\left({x}+{e}^{\frac{{i}\pi}{\mathrm{3}}} \right)^{{n}+\mathrm{1}} }\right. \\ $$$$\left.−\frac{\mathrm{1}}{\left({x}−{e}^{−\frac{{i}\pi}{\mathrm{3}}} \right)^{{n}+\mathrm{1}} }\:\:−\:\frac{\mathrm{1}}{\left({x}\:+{e}^{−\frac{{i}\pi}{\mathrm{3}}} \right)^{{n}+\mathrm{1}} }\:\:\right\} \\ $$$$ \\ $$

Commented by prof Abdo imad last updated on 17/Jun/18

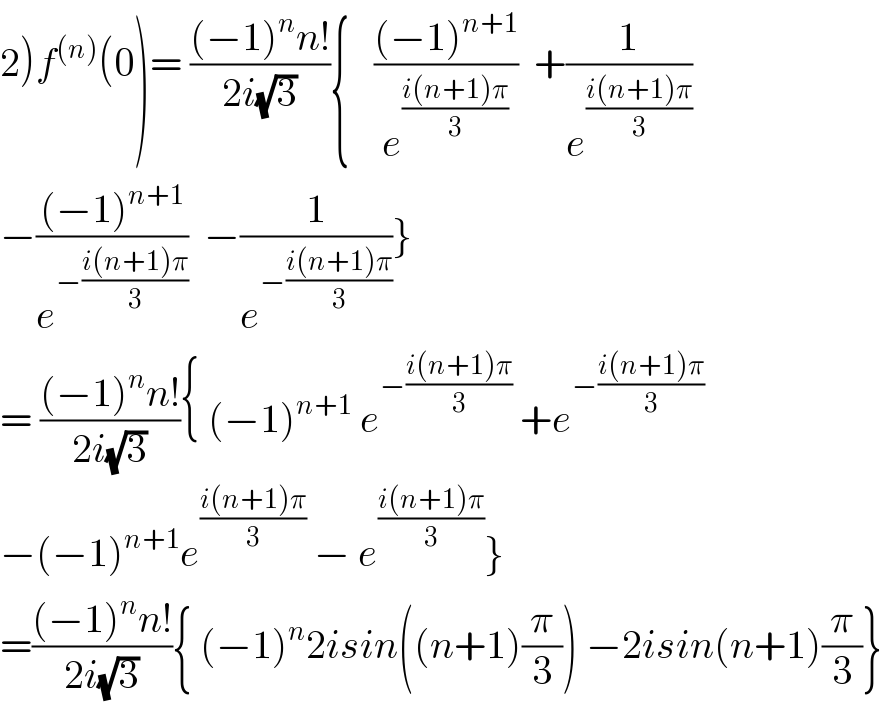
2)f^((n)) (0)= (((−1)^n n!)/(2i(√3))){ (((−1)^(n+1) )/e^((i(n+1)π)/3) ) +(1/e^((i(n+1)π)/3) ) −(((−1)^(n+1) )/e^(−((i(n+1)π)/3)) ) −(1/e^(−((i(n+1)π)/3)) )} = (((−1)^n n!)/(2i(√3))){ (−1)^(n+1) e^(−((i(n+1)π)/3)) +e^(−((i(n+1)π)/3)) −(−1)^(n+1) e^((i(n+1)π)/3) − e^((i(n+1)π)/3) } =(((−1)^n n!)/(2i(√3))){ (−1)^n 2isin((n+1)(π/3)) −2isin(n+1)(π/3)}

$$\left.\mathrm{2}\right){f}^{\left({n}\right)} \left(\mathrm{0}\right)=\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{2}{i}\sqrt{\mathrm{3}}}\left\{\:\:\:\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{{e}^{\frac{{i}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}} }\:\:+\frac{\mathrm{1}}{{e}^{\frac{{i}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}} }\right. \\ $$$$\left.−\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{{e}^{−\frac{{i}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}} }\:\:−\frac{\mathrm{1}}{{e}^{−\frac{{i}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}} }\right\} \\ $$$$=\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{2}{i}\sqrt{\mathrm{3}}}\left\{\:\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} \:{e}^{−\frac{{i}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}} \:+{e}^{−\frac{{i}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}} \right. \\ $$$$\left.−\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} {e}^{\frac{{i}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}} \:−\:{e}^{\frac{{i}\left({n}+\mathrm{1}\right)\pi}{\mathrm{3}}} \right\} \\ $$$$=\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{2}{i}\sqrt{\mathrm{3}}}\left\{\:\left(−\mathrm{1}\right)^{{n}} \mathrm{2}{isin}\left(\left({n}+\mathrm{1}\right)\frac{\pi}{\mathrm{3}}\right)\:−\mathrm{2}{isin}\left({n}+\mathrm{1}\right)\frac{\pi}{\mathrm{3}}\right\} \\ $$

Commented by prof Abdo imad last updated on 17/Jun/18

λ_k = (z_k /(p^′ (z_k ))) with p(x)= 1+x^2 +x^4 ⇒ λ_k = (z_k /(4z_k ^3 +2z_k )) so change f(z_k ) by λ_k

$$\lambda_{{k}} =\:\:\frac{{z}_{{k}} }{{p}^{'} \left({z}_{{k}} \right)}\:{with}\:\:{p}\left({x}\right)=\:\mathrm{1}+{x}^{\mathrm{2}} \:+{x}^{\mathrm{4}} \Rightarrow \\ $$$$\lambda_{{k}} =\:\frac{{z}_{{k}} }{\mathrm{4}{z}_{{k}} ^{\mathrm{3}} \:+\mathrm{2}{z}_{{k}} }\:\:\:{so}\:{change}\:{f}\left({z}_{{k}} \right)\:{by}\:\lambda_{{k}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com