let f(x) = ∫_0 ^1 ln(1+xt^2 )dt with ∣x∣<1 1) find f(x) 2) calculate ∫


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 37343 by math khazana by abdo last updated on 12/Jun/18

$l e t f (x) = \int_{0}^{1} l n (1 + {x t}^{2}) d t w i t h ∣ x ∣< 1$ $1) f i n d f (x)$ $2) c a l c u l a t e \int_{0}^{1} l n (2 + t^{2}) d t .$
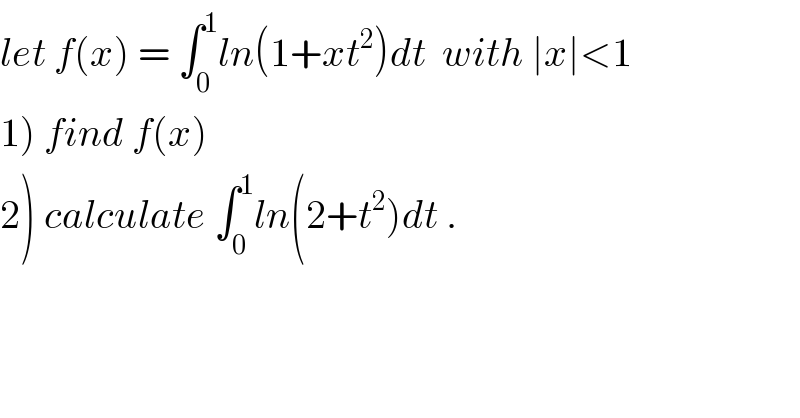
Commented bymath khazana by abdo last updated on 15/Jun/18
$if −1<x<0 we get f(x) = (1/x) −(1/x) ∫_0 ^1 (dt/(1−(−x)t^2 )) and chanvement t(√(−x))=u give f^′ (x) = (1/x) −(1/x) ∫_0 ^(√(−x)) (1/(1−u^2 )) (du/(√(−x))) =(1/x) −(1/(x(√(−x)))) ∫_0 ^(√(−x)) (du/(1−u^2 )) =(1/x) −(1/(2x(√(−x)))) ∫_0 ^(√(−x)) { (1/(1−u )) +(1/(1+u))}du =(1/x) −(1/(2x(√(−x)))) [ln∣((1+u)/(1−u))∣]_0 ^(√(−x)) = (1/x) −(1/(2x(√(−x)))) ln∣ ((1+(√(−x)))/(1−(√(−x)))) ∣ ⇒ f(x) = ∫_(−1) ^x (dt/t) −∫_(−1) ^x (1/(2t(√(−t))))ln∣ ((1+(√(−t)))/(1−(√(−t))))∣ dt +c c =f(−1) = ∫_0 ^1 ln(1−t^2 )dt ⇒ f(x)=ln(−x) −∫_(−1) ^x (1/(2t(√(−t))))ln∣ ((1+(√(−t)))/(1−(√(−t))))∣ dt + ∫_0 ^1 ln(1−t^2 )dt . with−1<x<0 ....$
$i f - 1 < x < 0 w e g e t$ $f (x) = \frac{1}{x} - \frac{1}{x} \int_{0}^{1} \frac{d t}{1 - (- x) t^{2}} a n d c h a n v e m e n t$ $t \sqrt{- x} = u g i v e$ $f^{^{'}} (x) = \frac{1}{x} - \frac{1}{x} \int_{0}^{\sqrt{- x}} \frac{1}{1 - u^{2}} \frac{d u}{\sqrt{- x}}$ $= \frac{1}{x} - \frac{1}{x \sqrt{- x}} \int_{0}^{\sqrt{- x}} \frac{d u}{1 - u^{2}}$ $= \frac{1}{x} - \frac{1}{2 x \sqrt{- x}} \int_{0}^{\sqrt{- x}} {\frac{1}{1 - u} + \frac{1}{1 + u}} d u$ $= \frac{1}{x} - \frac{1}{2 x \sqrt{- x}} {[l n ∣ \frac{1 + u}{1 - u} ∣]}_{0}^{\sqrt{- x}}$ $= \frac{1}{x} - \frac{1}{2 x \sqrt{- x}} l n ∣ \frac{1 + \sqrt{- x}}{1 - \sqrt{- x}} ∣ \Rightarrow$ $f (x) = \int_{- 1}^{x} \frac{d t}{t} - \int_{- 1}^{x} \frac{1}{2 t \sqrt{- t}} l n ∣ \frac{1 + \sqrt{- t}}{1 - \sqrt{- t}} ∣ d t + c$ $c = f (- 1) = \int_{0}^{1} l n (1 - t^{2}) d t \Rightarrow$ $f (x) = l n (- x) - \int_{- 1}^{x} \frac{1}{2 t \sqrt{- t}} l n ∣ \frac{1 + \sqrt{- t}}{1 - \sqrt{- t}} ∣ d t$ $+ \int_{0}^{1} l n (1 - t^{2}) d t . w i t h - 1 < x < 0 . . . .$
Commented bymath khazana by abdo last updated on 15/Jun/18
$1) we have f^′ (x)^ = ∫_0 ^1 (t^2 /(1+xt^2 ))dt so if x≠0 f^′ (x)=(1/x) ∫_0 ^1 ((xt^2 +1−1)/(1+xt^2 ))dt =(1/x) −(1/x) ∫_0 ^1 (dt/(1+xt^2 )) for0<x<1 changement t(√x)=u give ∫_0 ^1 (dt/(1+xt^2 )) = ∫_0 ^(√x) (du/(1+u^2 )) (du/(√x)) =(1/(√x)) arctan((√x))⇒ f^′ (x) = (1/x) −((arctan((√x)))/(x(√x))) ⇒ f(x) = ∫_1 ^x (dt/t) −∫_1 ^x ((arctan((√t)))/(t(√t))) dt+c c=f(1) =∫_0 ^1 ln(1+t^2 )dt chang.(√t)=u give ∫_1 ^(x ) ((arctan((√t)))/(t(√t))) dt = ∫_1 ^(√x) ((arctan(u))/(u^2 .u)) 2u du =2 ∫_1 ^(√x) ((arctan(u))/u^2 ) du =2{ [−(1/u) arctan(u)]_1 ^(√x) −∫_1 ^(√x) −(1/u) (1/(1+u^2 ))du} =2{ (π/4) −((arctan((√x)))/(√x)) } +2 ∫_1 ^(√x) (du/(u(1+u^2 ))) =(π/2) −((2arctan((√x)))/(√x)) +2 ∫_1 ^(√x) ((1/u) −(u/(1+u^2 )))du =(π/2) −((2 arctan((√x)))/(√x)) +2[ln((u/(√(1+u^2 ))))]_1 ^(√x) =(π/2) −2((arctan((√x)))/(√x)) +2 ln(((√x)/(√(1+x)))) ⇒ f(x)=ln(x) −(π/2) +((2 arctan((√x)))/(√x)) −2ln(((√x)/(√(1+x)))) +∫_0 ^1 ln(1+t^2 )dt f(x)=ln(x) −(π/2) +((2arctan((√x)))/(√x)) −lnx +ln(1+x) +∫_0 ^1 ln(1+t^2 )dt . and by parts ∫_0 ^1 ln(1+t^2 )dt = [t ln(1+t^2 )]_0 ^1 −∫_0 ^1 t ((2t)/(1+t^2 )) dt =ln(2) −2 ∫_0 ^1 ((t^2 +1−1)/(1+t^2 )) dt =ln(2) −2 +2 ∫_0 ^1 (dt/(1+t^2 )) =ln(2)−2 +(π/2) ⇒ f(x)= ((2 artan((√x)))/(√x)) +ln(1+x) +ln(2)−2 with 0<x<1$
$1) w e h a v e f^{^{'}} {(x)}^{} = \int_{0}^{1} \frac{t^{2}}{1 + {x t}^{2}} d t s o i f x \neq 0$ $f^{^{'}} (x) = \frac{1}{x} \int_{0}^{1} \frac{{x t}^{2} + 1 - 1}{1 + {x t}^{2}} d t = \frac{1}{x} - \frac{1}{x} \int_{0}^{1} \frac{d t}{1 + {x t}^{2}} f o r 0 < x < 1$ $c h a n g e m e n t t \sqrt{x} = u g i v e$ $\int_{0}^{1} \frac{d t}{1 + {x t}^{2}} = \int_{0}^{\sqrt{x}} \frac{d u}{1 + u^{2}} \frac{d u}{\sqrt{x}} = \frac{1}{\sqrt{x}} a r c t a n (\sqrt{x}) \Rightarrow$ $f^{^{'}} (x) = \frac{1}{x} - \frac{a r c t a n (\sqrt{x})}{x \sqrt{x}} \Rightarrow$ $f (x) = \int_{1}^{x} \frac{d t}{t} - \int_{1}^{x} \frac{a r c t a n (\sqrt{t})}{t \sqrt{t}} d t + c$ $c = f (1) = \int_{0}^{1} l n (1 + t^{2}) d t c h a n g . \sqrt{t} = u g i v e$ $\int_{1}^{x} \frac{a r c t a n (\sqrt{t})}{t \sqrt{t}} d t = \int_{1}^{\sqrt{x}} \frac{a r c t a n (u)}{u^{2} . u} 2 u d u$ $= 2 \int_{1}^{\sqrt{x}} \frac{a r c t a n (u)}{u^{2}} d u$ $= 2 {{[- \frac{1}{u} a r c t a n (u)]}_{1}^{\sqrt{x}} - \int_{1}^{\sqrt{x}} - \frac{1}{u} \frac{1}{1 + u^{2}} d u}$ $= 2 {\frac{π}{4} - \frac{a r c t a n (\sqrt{x})}{\sqrt{x}}} + 2 \int_{1}^{\sqrt{x}} \frac{d u}{u (1 + u^{2})}$ $= \frac{π}{2} - \frac{2 a r c t a n (\sqrt{x})}{\sqrt{x}} + 2 \int_{1}^{\sqrt{x}} (\frac{1}{u} - \frac{u}{1 + u^{2}}) d u$ $= \frac{π}{2} - \frac{2 a r c t a n (\sqrt{x})}{\sqrt{x}} + 2 {[l n (\frac{u}{\sqrt{1 + u^{2}}})]}_{1}^{\sqrt{x}}$ $= \frac{π}{2} - 2 \frac{a r c t a n (\sqrt{x})}{\sqrt{x}} + 2 l n (\frac{\sqrt{x}}{\sqrt{1 + x}}) \Rightarrow$ $f (x) = l n (x) - \frac{π}{2} + \frac{2 a r c t a n (\sqrt{x})}{\sqrt{x}} - 2 l n (\frac{\sqrt{x}}{\sqrt{1 + x}})$ $+ \int_{0}^{1} l n (1 + t^{2}) d t$ $f (x) = l n (x) - \frac{π}{2} + \frac{2 a r c t a n (\sqrt{x})}{\sqrt{x}} - l n x + l n (1 + x)$ $+ \int_{0}^{1} l n (1 + t^{2}) d t . a n d b y p a r t s$ $\int_{0}^{1} l n (1 + t^{2}) d t = {[t l n (1 + t^{2})]}_{0}^{1} - \int_{0}^{1} t \frac{2 t}{1 + t^{2}} d t$ $= l n (2) - 2 \int_{0}^{1} \frac{t^{2} + 1 - 1}{1 + t^{2}} d t$ $= l n (2) - 2 + 2 \int_{0}^{1} \frac{d t}{1 + t^{2}} = l n (2) - 2 + \frac{π}{2} \Rightarrow$ $f (x) = \frac{2 a r t a n (\sqrt{x})}{\sqrt{x}} + l n (1 + x) + l n (2) - 2$ $w i t h 0 < x < 1$
Commented bymath khazana by abdo last updated on 15/Jun/18

$2) \int_{0}^{1} l n (2 + t^{2}) d t = \int_{0}^{1} l n (2) d t + \int_{0}^{1} l n (1 + \frac{1}{2} t^{2}) d t$ $= l n (2) + f (\frac{1}{2})$ $= l n (2) + \frac{2 a r c t a n (\frac{1}{\sqrt{2}})}{\frac{1}{\sqrt{2}}} + l n (\frac{3}{2}) + l n (2) - 2$ $= l n (2) + l n (3) + 2 \sqrt{2} (\frac{π}{2} - a r c t a n (\sqrt{2})) - 2$ $= π \sqrt{2} - 2 \sqrt{2} a r c t a n (\sqrt{2}) + l n (6) - 2 .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum