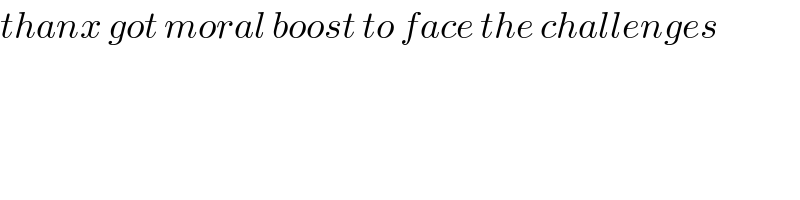f(θ,φ)=((cos φ[cos θ tan (((θ+φ)/2))−sin θ]^2 )/(cos φtan (((θ+φ)/2))+sin φ)) φ ∈ (0,(π/2)) , θ ∈ (−(π/2), (π/2)); find maximum f(θ,φ).


Question and Answers Forum

All Questions Topic List
Differentiation Questions
Previous in All Question Next in All Question
Previous in Differentiation Next in Differentiation
Question Number 37840 by ajfour last updated on 18/Jun/18

$f (θ, ϕ) = \frac{\cos ϕ {[\cos θ \tan (\frac{θ + ϕ}{2}) - \sin θ]}^{2}}{\cos ϕ \tan (\frac{θ + ϕ}{2}) + \sin ϕ}$ $ϕ \in (0, \frac{π}{2}), θ \in (- \frac{π}{2}, \frac{π}{2});$ $f i n d m a x i m u m f (θ, ϕ) .$
	Answered by tanmay.chaudhury50@gmail.com last updated on 18/Jun/18

	$f (θ, ϕ) = \frac{N_{r}}{D_{r}}$ $N_{r} = c o s ϕ {[c o s θ t a n (\frac{θ + ϕ}{2}) - s i n θ]}^{2}$ $= c o s ϕ {[\frac{c o s θ s i n (\frac{θ + ϕ}{2}) - s i n θ c o s (\frac{θ + ϕ}{2})}{c o s (\frac{θ + \emptyset}{2})}]}^{2}$ $= c o s ϕ {[\frac{s i n (\frac{ϕ - θ}{2})}{c o s (\frac{θ + ϕ}{2})}]}^{2}$ $D_{r} = c o s ϕ t a n (\frac{θ + ϕ}{2}) + s i n ϕ$ $= \frac{c o s ϕ . s i n (\frac{θ + ϕ}{2}) + c o s (\frac{θ + ϕ}{2}) s i n ϕ}{c o s (\frac{θ + \emptyset}{2})}$ $= \frac{s i n (\frac{θ + 3 ϕ}{2})}{c o s (\frac{θ + ϕ}{2})}$ $f (θ, ϕ) = \frac{c o s ϕ {[s i n (\frac{ϕ - θ}{2})]}^{2} \times c o s (\frac{θ + ϕ}{2})}{s i n (\frac{θ + 3 ϕ}{2}) \times {[c o s \frac{(θ + ϕ}{2}]}^{2}}$ $= \frac{c o s ϕ {[s i n (\frac{ϕ - θ}{2})]}^{2}}{s i n (\frac{θ + 3 ϕ}{2}) c o s (\frac{θ + ϕ}{2})}$ $= \frac{2 c o s ϕ {[s i n (\frac{ϕ - θ}{2})]}^{2}}{2 s i n (ϕ + \frac{θ + \emptyset}{2}) c o s (\frac{θ + ϕ}{2})} = \frac{2 c o s 0 [{s i n}^{2} (- \frac{Π}{4})}}{2 s i n \frac{Π}{4} \times c o s \frac{Π}{4}} = 1$ $w a i t . . . ϕ (0, \frac{Π}{2}) θ (\frac{- Π}{2}, \frac{Π}{2})$ $m a x N_{r} w h e n ϕ = 0 θ = \frac{Π}{2}$
	Commented by ajfour last updated on 18/Jun/18

	$i s h o u l d h a v e g i v e n θ, ϕ \in [- \frac{π}{2}, \frac{π}{2}] .$ $T h a n k y o u T a n m a y S i r .$ $Y o u r a n s w e r i s i n d e e d r i g h t,$ $i b e l i e v e .$
	Commented by tanmay.chaudhury50@gmail.com last updated on 18/Jun/18

	$t h a n x g o t m o r a l b o o s t t o f a c e t h e c h a l l e n g e s$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum