Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 38181 by rahul 19 last updated on 22/Jun/18

$$\mathrm{If}\mathrm{y}=x^{{\left(lnx\right)}^{ln\left(lnx\right)}}then\frac{dy}{dx}=?$$

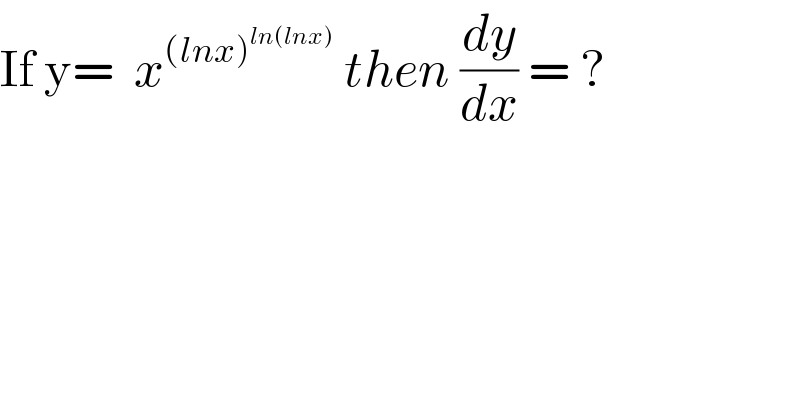
Commented by rahul 19 last updated on 22/Jun/18

$$\mathrm{I}{}^{\prime }\mathrm{ve}\mathrm{done}\mathrm{by}\mathrm{taking}\log \mathrm{and}{\mathrm{I}}^{\prime }\mathrm{m}\mathrm{getting}$$$$\frac{\mathrm{y}}{x}{\left(lnx\right)}^{ln\left(lnx\right)}(ln\left(lnx\right)+1).$$$$S\mathrm{omeone}\mathrm{pls}\mathrm{verify}.$$

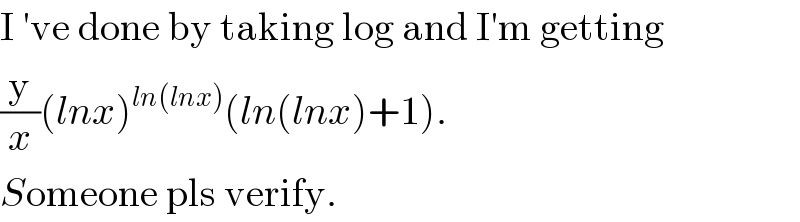
Commented by MJS last updated on 23/Jun/18

$${\mathrm{it}}^{\prime }\mathrm{s}\mathrm{almost}\mathrm{right},\mathrm{but}\mathrm{the}\mathrm{last}\mathrm{factor}\mathrm{must}\mathrm{be}$$$$(2\ln \left(\ln x\right)+1)$$

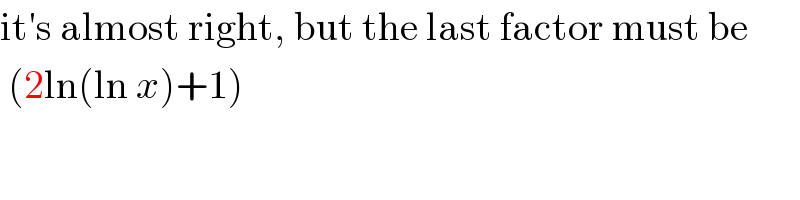
Commented by abdo mathsup 649 cc last updated on 23/Jun/18

$$letputa\left(x\right)={\left(ln\left(x\right)\right)}^{ln\left(lnx\right)}\Rightarrow y\left(x\right)=x^{a\left(x\right)}$$$$=e^{a\left(x\right)ln\left(x\right)}\Rightarrow \frac{dy}{dx}\left(x\right)={\left\{xa\left(x\right)\right\}}^{{}^{\prime }}y\left(x\right)$$$$=(a\left(x\right)+{xa}^{{}^{\prime }}\left(x\right))y\left(x\right)but$$$$a\left(x\right)=e^{ln(ln\left(x\right)ln\left(lnx\right)}=e^{{\left\{ln\left(lnx\right)\right\}}^2\Rightarrow }$$$$a^{{}^{\prime }}\left(x\right)=2\left(ln\left(lnx\right)\right){\left(ln\left(lnx\right)\right)}^{{}^{\prime }}a\left(x\right)$$$$=\frac{2}{xln\left(x\right)}ln\left(ln\left(x\right)\right)a\left(x\right)\Rightarrow$$$$y^{{}^{\prime }}\left(x\right)=a\left(x\right)(1+x\frac{2}{xln\left(x\right)}ln\left(ln\left(x\right)\right))y\left(x\right)$$$$=a\left(x\right)y\left(x\right)(1+\frac{2ln\left(ln\left(x\right)\right)}{ln\left(x\right)}).$$

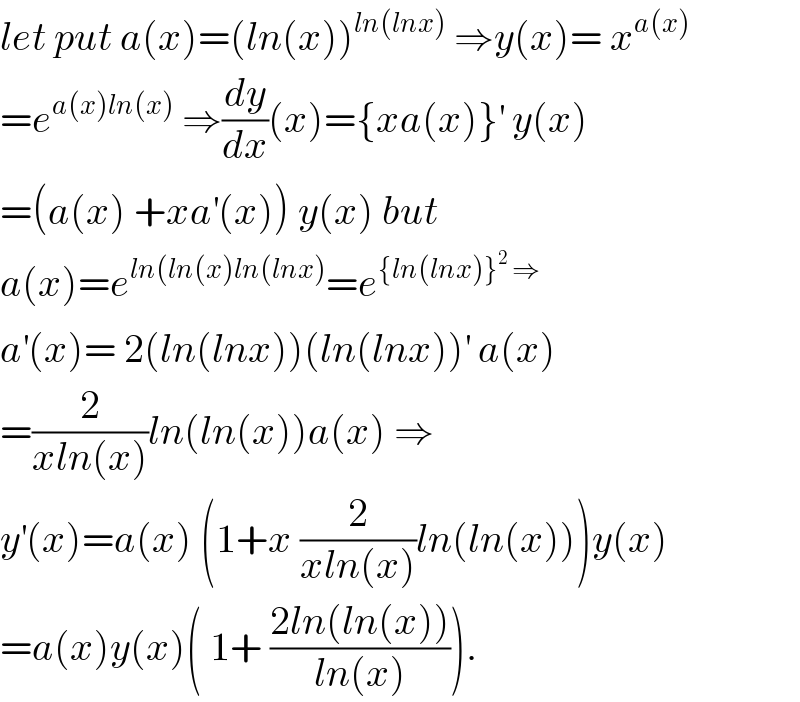
Answered by MJS last updated on 23/Jun/18

$$\frac{d}{dx}\left[x^{f\left(x\right)}\right]=x^{f\left(x\right)}\times (\frac{f\left(x\right)}{x}+\frac{d}{dx}\left[f\left(x\right)\right]\times \ln x)$$$$f\left(x\right)={\left(\ln x\right)}^{g\left(x\right)}$$$$\frac{d}{dx}\left[f\left(x\right)\right]={\left(\ln x\right)}^{g\left(x\right)}\times (\frac{g\left(x\right)}{x\ln x}+\frac{d}{dx}\left[g\left(x\right)\right]\times \ln \left(\ln x\right))$$$$g\left(x\right)=\ln \left(\ln x\right)$$$$\frac{d}{dx}\left[g\left(x\right)\right]=\frac{1}{x\ln x}$$$$$$$$\frac{d}{dx}\left[f\left(x\right)\right]={\left(\ln x\right)}^{\ln \left(\ln x\right)}\times (\frac{\ln \left(\ln x\right)}{x\ln x}+\frac{1}{x\ln x}\times \ln \left(\ln x\right))=$$$$=2\frac{\ln \left(\ln x\right)}{x\ln x}{\left(\ln x\right)}^{\ln \left(\ln x\right)}$$$$\frac{d}{dx}\left[x^{f\left(x\right)}\right]=x^{{\left(\ln x\right)}^{\ln \left(\ln x\right)}}\times (\frac{{\left(\ln x\right)}^{\ln \left(\ln x\right)}}{x}+2\frac{\ln \left(\ln x\right)}{x\ln x}{\left(\ln x\right)}^{\ln \left(\ln x\right)}\times \ln x)=$$$$=(1+2\ln \left(\ln x\right)){\left(\ln x\right)}^{\ln \left(\ln x\right)}{x}^{{\left(\ln x\right)}^{\ln \left(\ln x\right)}-1}$$

Answered by rahul 19 last updated on 23/Jun/18

$$lny={\left(lnx\right)}^{ln\left(lnx\right)}\left(lnx\right)$$$$\Rightarrow \ln \mathrm{y}=\left(lnx\right){}^{(ln\left(lnx\right)+1)}$$$$\Rightarrow \frac{\mathrm{dy}}{\mathrm{d}x}\times \frac{1}{y}=(ln\left(lnx\right)+1){\left(lnx\right)}^{\left(ln\left(lnx\right)\right)}\times \frac{1}{x}$$$$\Rightarrow \frac{\mathrm{dy}}{\mathrm{d}x}=\frac{y}{x}(1.ln\left(lnx\right)+1){\left(lnx\right)}^{\left(ln\left(lnx\right)\right)}.$$$$W\mathrm{here}\mathrm{am}\mathrm{i}\mathrm{wrong}??$$$$\mathrm{Sir}\mathrm{MJS}??$$

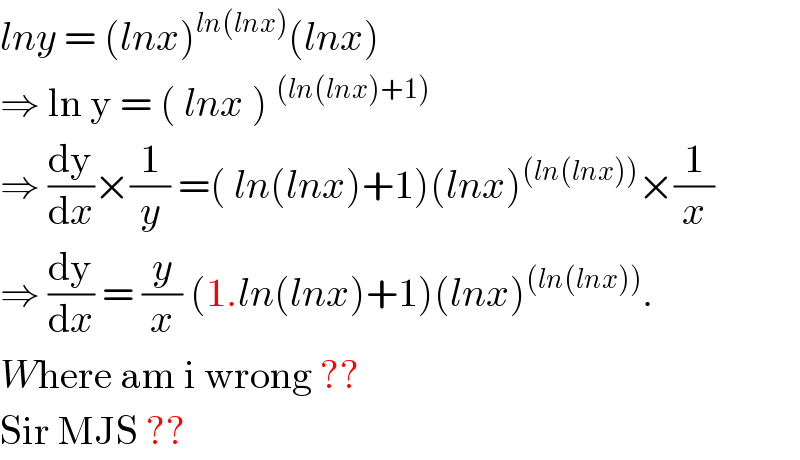
Commented by MJS last updated on 23/Jun/18

$$\mathrm{please}\mathrm{post}\mathrm{the}\mathrm{formula}{\mathrm{you}}^{\prime }\mathrm{re}\mathrm{using},\mathrm{I}{\mathrm{don}}^{\prime }\mathrm{t}$$$$\mathrm{understand}\mathrm{how}\mathrm{you}\mathrm{get}\mathrm{to}\mathrm{this}:$$$$\frac{\mathrm{dy}}{\mathrm{d}x}\times \frac{1}{y}=(ln\left(lnx\right)+1){\left(lnx\right)}^{\left(ln\left(lnx\right)\right)}\times \frac{1}{x}$$

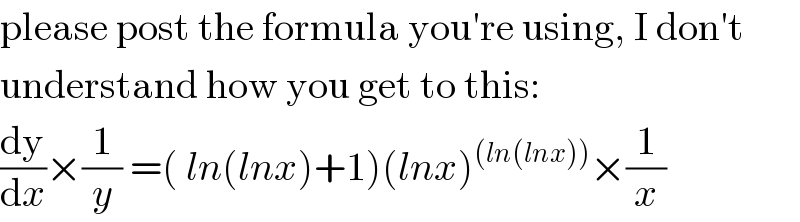
Commented by rahul 19 last updated on 24/Jun/18

$$\mathrm{Ok}\mathrm{i}\mathrm{got}\mathrm{my}\mathrm{mistake}!:)$$$$\mathrm{i}\mathrm{did}\mathrm{like}\mathrm{differentiate}{x}^x$$$$thenisimplywrotex.x^{x-1}.1which$$$$isabsolutelywrong!$$

Commented by MJS last updated on 24/Jun/18

$$\mathrm{good}!$$$$\mathrm{learning}\mathrm{by}\mathrm{doing}\mathrm{and}\mathrm{learning}\mathrm{by}\mathrm{mistakes}$$$$:-)$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com