Question and Answers Forum

All Questions      Topic List

Relation and Functions Questions

Previous in All Question      Next in All Question      

Previous in Relation and Functions      Next in Relation and Functions      

Question Number 38740 by abdo.msup.com last updated on 29/Jun/18

find tbe polynom p withdegre 5 wich verify p(x+1)−p(x)=x^4 and p(0)=0 for that put p(x)=ax^5 +bx^4 +cx^3 +dx^2 +ex +f and find the coefficients. 2) find interms of n the value of sum 1 +2^4 +3^4 +....+n^4 .

$${find}\:{tbe}\:{polynom}\:{p}\:{withdegre}\:\mathrm{5}\:{wich}\:{verify} \\ $$$${p}\left({x}+\mathrm{1}\right)−{p}\left({x}\right)={x}^{\mathrm{4}} \:\:{and}\:{p}\left(\mathrm{0}\right)=\mathrm{0} \\ $$$${for}\:{that}\:{put}\:{p}\left({x}\right)={ax}^{\mathrm{5}} \:+{bx}^{\mathrm{4}} \:+{cx}^{\mathrm{3}} \:+{dx}^{\mathrm{2}} \\ $$$$+{ex}\:+{f}\:\:{and}\:{find}\:{the}\:{coefficients}. \\ $$$$\left.\mathrm{2}\right)\:{find}\:{interms}\:{of}\:{n}\:{the}\:{value}\:{of} \\ $$$${sum}\:\mathrm{1}\:+\mathrm{2}^{\mathrm{4}} \:+\mathrm{3}^{\mathrm{4}} +....+{n}^{\mathrm{4}} \:. \\ $$

Commented by prakash jain last updated on 29/Jun/18

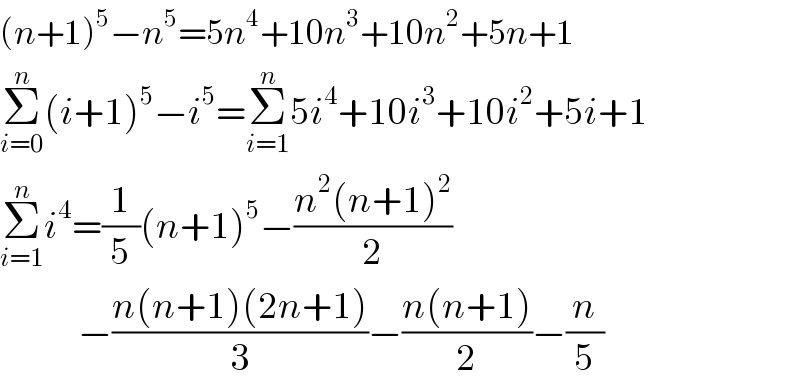
(n+1)^5 −n^5 =5n^4 +10n^3 +10n^2 +5n+1 Σ_(i=0) ^n (i+1)^5 −i^5 =Σ_(i=1) ^n 5i^4 +10i^3 +10i^2 +5i+1 Σ_(i=1) ^n i^4 =(1/5)(n+1)^5 −((n^2 (n+1)^2 )/2) −((n(n+1)(2n+1))/3)−((n(n+1))/2)−(n/5)

$$\left({n}+\mathrm{1}\right)^{\mathrm{5}} −{n}^{\mathrm{5}} =\mathrm{5}{n}^{\mathrm{4}} +\mathrm{10}{n}^{\mathrm{3}} +\mathrm{10}{n}^{\mathrm{2}} +\mathrm{5}{n}+\mathrm{1} \\ $$$$\underset{{i}=\mathrm{0}} {\overset{{n}} {\sum}}\left({i}+\mathrm{1}\right)^{\mathrm{5}} −{i}^{\mathrm{5}} =\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}\mathrm{5}{i}^{\mathrm{4}} +\mathrm{10}{i}^{\mathrm{3}} +\mathrm{10}{i}^{\mathrm{2}} +\mathrm{5}{i}+\mathrm{1} \\ $$$$\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{i}^{\mathrm{4}} =\frac{\mathrm{1}}{\mathrm{5}}\left({n}+\mathrm{1}\right)^{\mathrm{5}} −\frac{{n}^{\mathrm{2}} \left({n}+\mathrm{1}\right)^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:−\frac{{n}\left({n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)}{\mathrm{3}}−\frac{{n}\left({n}+\mathrm{1}\right)}{\mathrm{2}}−\frac{{n}}{\mathrm{5}} \\ $$

Commented by math khazana by abdo last updated on 29/Jun/18

sir Prakash are you staying in india or abroad..

$${sir}\:{Prakash}\:{are}\:{you}\:{staying}\:{in}\:{india}\:{or}\:{abroad}.. \\ $$

Commented by prakash jain last updated on 30/Jun/18

I am in India.

$$\mathrm{I}\:\mathrm{am}\:\mathrm{in}\:\mathrm{India}. \\ $$

Commented by math khazana by abdo last updated on 30/Jun/18

good sir i hope to take a trip to india and see the himalaya mountains and other places...

$${good}\:{sir}\:{i}\:{hope}\:{to}\:{take}\:{a}\:{trip}\:{to}\:{india}\:{and}\:{see}\: \\ $$$${the}\:{himalaya}\:{mountains}\:{and}\:{other}\:{places}... \\ $$

Answered by MrW3 last updated on 29/Jun/18

p(x)=ax^5 +bx^4 +cx^3 +dx^2 +ex+f p(0)=0⇒f=0 p(x+1)=a(x+1)^5 +b(x+1)^4 +c(x+1)^3 +d(x+1)^2 +e(x+1) p(x+1)−p(x)=a[(x+1)^5 −x^5 ]+b[(x+1)^4 −x^4 ]+c[(x+1)^3 −x^3 ]+d[(x+1)^2 −x^2 ]+e=x^4 a[5x^4 +10x^3 +10x^2 +5x+1]+b[4x^3 +6x^2 +4x+1]+c[3x^2 +3x+1]+d[2x+1]+e=x^4 5ax^4 +(10a+4b)x^3 +(10a+6b+3c)x^2 +(5a+4b+3c+2d)x+(a+b+c+d+e)=x^4 ⇒5a=1⇒a=(1/5) ⇒10a+4b=0⇒b=−(5/2)a=−(1/2) ⇒10a+6b+3c=0⇒c=−((10)/3)a−2b=−(1/6)+1=−(5/6) ⇒5a+4b+3c+2d=0⇒d=−(5/2)a−2b−(3/2)c=−(1/2)+1+(5/4)=(7/4) ⇒a+b+c+d+e=0⇒e=−a−b−c−d=−(1/5)+(1/2)+(5/6)−(7/4)=((−12+30+50−85)/(60))=−((17)/(60))

$${p}\left({x}\right)={ax}^{\mathrm{5}} \:+{bx}^{\mathrm{4}} \:+{cx}^{\mathrm{3}} \:+{dx}^{\mathrm{2}} +{ex}+{f} \\ $$$${p}\left(\mathrm{0}\right)=\mathrm{0}\Rightarrow{f}=\mathrm{0} \\ $$$${p}\left({x}+\mathrm{1}\right)={a}\left({x}+\mathrm{1}\right)^{\mathrm{5}} \:+{b}\left({x}+\mathrm{1}\right)^{\mathrm{4}} \:+{c}\left({x}+\mathrm{1}\right)^{\mathrm{3}} \:+{d}\left({x}+\mathrm{1}\right)^{\mathrm{2}} +{e}\left({x}+\mathrm{1}\right) \\ $$$${p}\left({x}+\mathrm{1}\right)−{p}\left({x}\right)={a}\left[\left({x}+\mathrm{1}\right)^{\mathrm{5}} −{x}^{\mathrm{5}} \right]+{b}\left[\left({x}+\mathrm{1}\right)^{\mathrm{4}} −{x}^{\mathrm{4}} \right]+{c}\left[\left({x}+\mathrm{1}\right)^{\mathrm{3}} −{x}^{\mathrm{3}} \right]+{d}\left[\left({x}+\mathrm{1}\right)^{\mathrm{2}} −{x}^{\mathrm{2}} \right]+{e}={x}^{\mathrm{4}} \\ $$$${a}\left[\mathrm{5}{x}^{\mathrm{4}} +\mathrm{10}{x}^{\mathrm{3}} +\mathrm{10}{x}^{\mathrm{2}} +\mathrm{5}{x}+\mathrm{1}\right]+{b}\left[\mathrm{4}{x}^{\mathrm{3}} +\mathrm{6}{x}^{\mathrm{2}} +\mathrm{4}{x}+\mathrm{1}\right]+{c}\left[\mathrm{3}{x}^{\mathrm{2}} +\mathrm{3}{x}+\mathrm{1}\right]+{d}\left[\mathrm{2}{x}+\mathrm{1}\right]+{e}={x}^{\mathrm{4}} \\ $$$$\mathrm{5}{ax}^{\mathrm{4}} +\left(\mathrm{10}{a}+\mathrm{4}{b}\right){x}^{\mathrm{3}} +\left(\mathrm{10}{a}+\mathrm{6}{b}+\mathrm{3}{c}\right){x}^{\mathrm{2}} +\left(\mathrm{5}{a}+\mathrm{4}{b}+\mathrm{3}{c}+\mathrm{2}{d}\right){x}+\left({a}+{b}+{c}+{d}+{e}\right)={x}^{\mathrm{4}} \\ $$$$\Rightarrow\mathrm{5}{a}=\mathrm{1}\Rightarrow{a}=\frac{\mathrm{1}}{\mathrm{5}} \\ $$$$\Rightarrow\mathrm{10}{a}+\mathrm{4}{b}=\mathrm{0}\Rightarrow{b}=−\frac{\mathrm{5}}{\mathrm{2}}{a}=−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{10}{a}+\mathrm{6}{b}+\mathrm{3}{c}=\mathrm{0}\Rightarrow{c}=−\frac{\mathrm{10}}{\mathrm{3}}{a}−\mathrm{2}{b}=−\frac{\mathrm{1}}{\mathrm{6}}+\mathrm{1}=−\frac{\mathrm{5}}{\mathrm{6}} \\ $$$$\Rightarrow\mathrm{5}{a}+\mathrm{4}{b}+\mathrm{3}{c}+\mathrm{2}{d}=\mathrm{0}\Rightarrow{d}=−\frac{\mathrm{5}}{\mathrm{2}}{a}−\mathrm{2}{b}−\frac{\mathrm{3}}{\mathrm{2}}{c}=−\frac{\mathrm{1}}{\mathrm{2}}+\mathrm{1}+\frac{\mathrm{5}}{\mathrm{4}}=\frac{\mathrm{7}}{\mathrm{4}} \\ $$$$\Rightarrow{a}+{b}+{c}+{d}+{e}=\mathrm{0}\Rightarrow{e}=−{a}−{b}−{c}−{d}=−\frac{\mathrm{1}}{\mathrm{5}}+\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{5}}{\mathrm{6}}−\frac{\mathrm{7}}{\mathrm{4}}=\frac{−\mathrm{12}+\mathrm{30}+\mathrm{50}−\mathrm{85}}{\mathrm{60}}=−\frac{\mathrm{17}}{\mathrm{60}} \\ $$

Commented by math khazana by abdo last updated on 29/Jun/18

thank you sir.

$${thank}\:{you}\:{sir}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com