Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 38907 by ajfour last updated on 01/Jul/18

$$\sqrt{c^2+x^2}=1+\frac{c}{x}$$$$howmanycomplexroots?$$

Commented by ajfour last updated on 01/Jul/18

Commented by MJS last updated on 01/Jul/18

$$\sqrt{c^2+x^2}=\frac{c+x}{x}\mathrm{has}\mathrm{only}3\mathrm{roots}.\mathrm{if}\mathrm{you}\mathrm{square}$$$$\mathrm{to}\mathrm{solve}\mathrm{it},\mathrm{you}\mathrm{get}4\mathrm{roots}\mathrm{of}\mathrm{which}\mathrm{one}\mathrm{is}\mathrm{no}$$$$\mathrm{solution}\mathrm{of}\mathrm{the}\mathrm{original}\mathrm{equation}$$

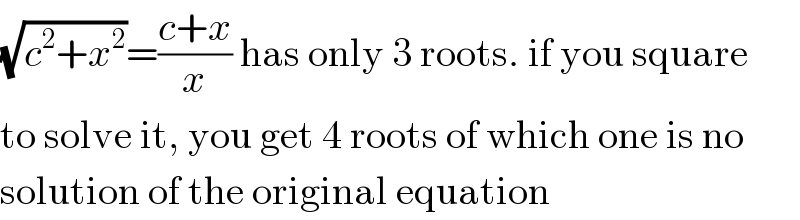
Commented by MrW3 last updated on 01/Jul/18

$${You}^{\prime }rerightsir.Sotheansweris:$$$$dependingonthevalueofc,the$$$$originaleqn.haseithernoneortwo$$$$complexroots.$$

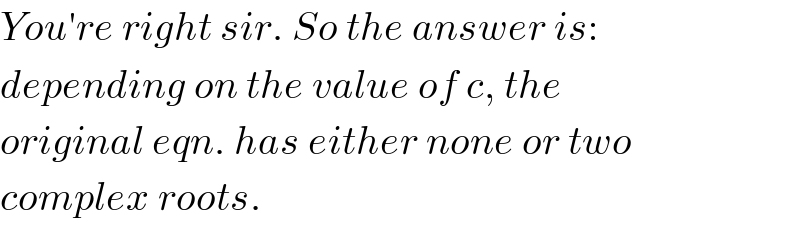
Commented by ajfour last updated on 01/Jul/18

$$Thanksforthetruth,sir.$$

Commented by MJS last updated on 01/Jul/18

$$\mathrm{we}\mathrm{can}\mathrm{find}\mathrm{the}\mathrm{value}\mathrm{of}c=\mathfrak{c}\mathrm{with}$$$$c=\mathfrak{c}\Rightarrow f\left(x\right)=\sqrt{x^2+c^2}-\frac{x+c}{x}=0\mathrm{has}\mathrm{exactly}2\mathrm{real}\mathrm{solutions}$$$$c<\mathfrak{c}\Rightarrow f\left(x\right)=0\mathrm{has}3\mathrm{real}\mathrm{solutions}$$$$c>\mathfrak{c}\Rightarrow f\left(x\right)=0\mathrm{has}1\mathrm{real}\mathrm{and}2\mathrm{complex}\mathrm{solutions}$$$$$$$$x^4+({\mathfrak{c}}^2-1)x^2-2\mathfrak{c}x-{\mathfrak{c}}^2=0$$$${(x-\alpha )}^2(x+\alpha -\beta )(x+\alpha +\beta )=0$$$${\mathrm{it}}^{\prime }\mathrm{s}\mathrm{the}\mathrm{same}\mathrm{way}\mathrm{as}\mathrm{several}\mathrm{times}\mathrm{before},\mathrm{in}$$$$\mathrm{this}\mathrm{special}\mathrm{case}\mathrm{we}\mathrm{get}3\mathrm{equations}\mathrm{in}\alpha ,\beta ,\mathfrak{c}$$$$\mathrm{with}\alpha >0,\beta >0,\mathfrak{c}<0\mathrm{and}{\beta }^2\mathrm{is}\mathrm{the}\mathrm{only}\mathrm{real}$$$$\mathrm{solution}\mathrm{of}\mathrm{a}\mathrm{polynome}\mathrm{of}{3}^{\mathrm{rd}}\mathrm{degree}.$$$$x=-\alpha +\beta \mathrm{should}\mathrm{be}\mathrm{the}‘‘{\mathrm{wrong}}^{″}\mathrm{solution}\mathrm{I}\mathrm{guess}$$$$\mathrm{I}\mathrm{might}\mathrm{post}\mathrm{it}\mathrm{later}\mathrm{but}\mathrm{it}\mathrm{should}\mathrm{be}\mathrm{easy}\mathrm{to}$$$$\mathrm{solve}$$

Answered by tanmay.chaudhury50@gmail.com last updated on 01/Jul/18

$$c^2+x^2=1+\frac{2c}{x}+\frac{c^2}{x^2}$$$$c^2+x^2=\frac{x^2+2cx+c^2}{x^2}$$$$x^2{c}^2+x^4=x^2+2cx+c^2$$$$x^4+x^2(c^2-1)-2cx-c^2=0$$$$f\left(x\right)=x^4+0.x^3+(c^2-1)x^2-2cx-c^2$$$$let{c}^2>1usingDescartsruleofsign$$$$onesignchangesoonepositiveroot$$$$f(-x)=x^4+0.(-x^3)+(c^2-1)x^2+2cx-c^2$$$$onesignchangesoonenegdtiveroot$$$$so4-1-1=2complxroot$$$$nowif{c}^2<1$$$$f\left(x\right)=x^4+0.x^3-(1-c^2)x^2+2cx-c^2$$$$2+veroot$$$$f(-x)=x^4-(1-c^2)x^2-2cx-c^2$$$$1(-ve)root$$$$soonecomplexroot$$

Commented by ajfour last updated on 01/Jul/18

$$Thankyouverymuch,SirTanmay.$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com