calculate ∫_0 ^∞ (dt/(1+t^(4 ) +t^6 ))


Question and Answers Forum

All Questions Topic List
Integration Questions
Previous in All Question Next in All Question
Previous in Integration Next in Integration
Question Number 39165 by math khazana by abdo last updated on 03/Jul/18

$c a l c u l a t e \int_{0}^{\infty} \frac{d t}{1 + t^{4} + t^{6}}$
Commented by tanmay.chaudhury50@gmail.com last updated on 03/Jul/18

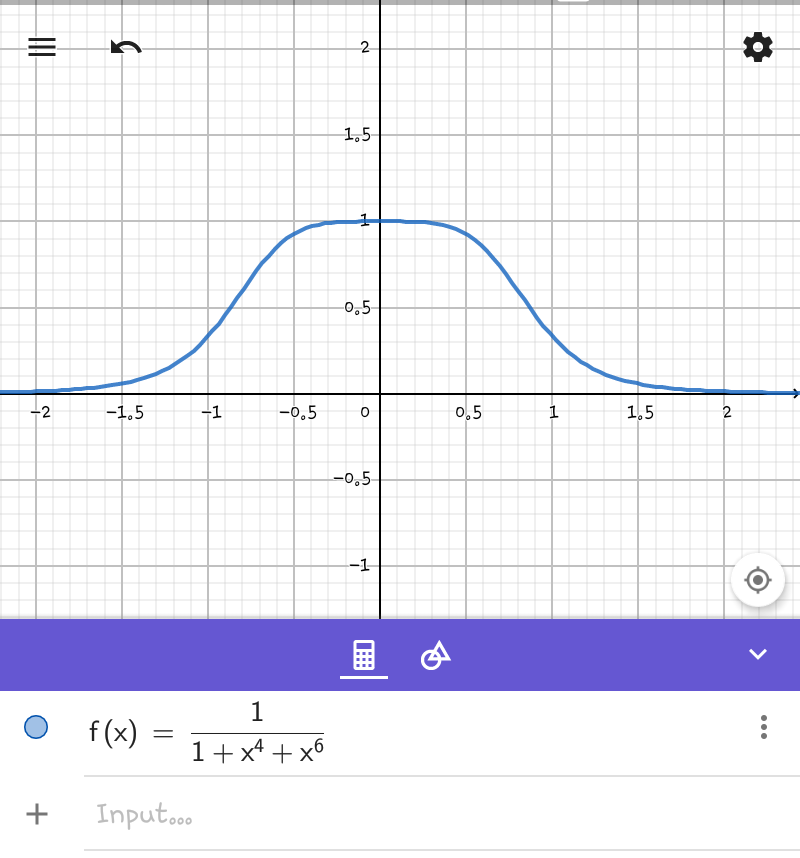
Commented by tanmay.chaudhury50@gmail.com last updated on 03/Jul/18

$1.5 \times 1 > \int_{0}^{\infty} \frac{d t}{1 + t^{4} + t^{6}} > 3 \times {(0.5)}^{2}$ $1.5 > \int_{0}^{\infty} \frac{d t}{1 + t^{4} + t^{6}} > 0.75$
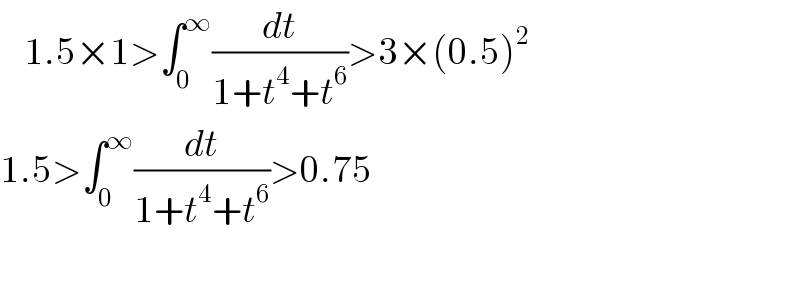
Commented by math khazana by abdo last updated on 04/Jul/18

$l e t I = \int_{0}^{\infty} \frac{d t}{1 + t^{4} + t^{6}}$ $2 I = \int_{- \infty}^{+ \infty} \frac{d t}{1 + t^{4} + t^{6}} l e t φ (z) = \frac{1}{1 + z^{4} + z^{8}}$ $p o l e s o f φ ?$ $1 + z^{4} + z^{8} = 0 \Leftrightarrow 1 + z^{4} + {(z^{4})}^{2} = 0$ $\Leftrightarrow \frac{1 - {(z^{4})}^{3}}{1 - z^{4}} = 0 a n d z^{4} \neq 1 \Leftrightarrow z^{12} = 1 a n d z^{4} \neq 1$ $z^{4} = 1 \Rightarrow 4 θ = 2 k π \Rightarrow θ_{k} = \frac{k π}{2} a n d k \in [[0, 3]]$ $z_{k} = e^{i \frac{k π}{2}} l e t z = {r e}^{i α} s o z^{12} = 1 \Rightarrow r = 1 a n d$ $12 α = 2 k π \Rightarrow α_{k} = \frac{k π}{6} w i t h k \in [[0, 11]] \Rightarrow$ $z_{k} = e^{i \frac{k π}{6}} a n d k \in [[0, 5]]$ $z_{0} = 1 (n o n p o l e o f φ)$ $z_{1} = e^{i \frac{π}{6}} z_{1}^{4} = e^{i \frac{2 π}{3}} \neq 1 \Rightarrow z_{1} p o l e o f φ$ $z_{2} = e^{i \frac{π}{3}} (z_{2}^{4} \neq 1 \Rightarrow z_{2} p o l e o f φ)$ $z_{3} = e^{i \frac{π}{2}} (z_{3}^{4} = 1 s o z_{3} n o n p o l e o f φ)$ $z_{4} = e^{i \frac{2 π}{3}} (z_{4}^{4} \neq 1 s o z_{4} p o l e o f φ)$ $z_{5} = e^{i \frac{5 π}{6}} (z_{5}^{4} \neq 1 s o z_{5} i s p o l e o f φ)$ $z_{6} = e^{i π} (z_{6}^{4} = 1 n o n p o l e o f φ)$ $z_{7} = e^{i \frac{7 π}{6}} = - e^{i \frac{π}{6}} (p o l e o f φ)$ $z_{8} = e^{i \frac{8 π}{6}} = e^{i \frac{4 π}{3}} (p o l e o f φ)$ $z_{9} = e^{i \frac{9 π}{6}} = e^{i \frac{3 π}{2}} (n o n p o l e o f φ)$ $z_{10} = e^{i \frac{10 π}{6}} = e^{i \frac{5 π}{3}} (p o l e o f φ)$ $z_{11} = e^{i \frac{11 π}{6}} = - e^{- i \frac{π}{6}} (p o l e o f φ) b e c o n t i n u e d . . .$
Commented by math khazana by abdo last updated on 04/Jul/18
$∫_(−∞) ^(+∞) ϕ(z)dz =2iπ{ Res(ϕ,z_1 ) +Res(ϕ,z_2 ) +Res(ϕ,z_4 ) +Res(ϕ,z_5 ) +Res(ϕ, z_7 ) +Res(ϕ^� ,z_8 ) +Res(ϕ,z_(10) ) +Res(ϕ,z_(11) ) } Res(ϕ,z_k ) = (1/(4z_k ^3 + 6z_k ^5 )) ⇒ Res(ϕ,z_1 ) = (1/(4(e^(i(π/6)) )^3 +6(e^((iπ)/6) )^5 )) = (1/(4i +6 e^((i5π)/6) )) Res(ϕ,z_2 ) = (1/(4(e^((iπ)/3) )^3 +6 (e^((iπ)/3) )^5 )) = (1/(−4 +6 e^((i5π)/3) )) Res(ϕ,z_4 )= (1/(4(e^((i2π)/3) )^3 +6(e^((i2π)/3) )^5 )) =(1/(4 +6 e^((i10π)/3) )) =....$
$\int_{- \infty}^{+ \infty} φ (z) d z = 2 i π {R e s (φ, z_{1}) + R e s (φ, z_{2}) + R e s (φ, z_{4})$ $+ R e s (φ, z_{5}) + R e s (φ, z_{7}) + R e s (\bar{φ}, z_{8}) + R e s (φ, z_{10})$ $+ R e s (φ, z_{11})}$ $R e s (φ, z_{k}) = \frac{1}{4 z_{k}^{3} + 6 z_{k}^{5}} \Rightarrow$ $R e s (φ, z_{1}) = \frac{1}{4 {(e^{i \frac{π}{6}})}^{3} + 6 {(e^{\frac{i π}{6}})}^{5}}$ $= \frac{1}{4 i + 6 e^{\frac{i 5 π}{6}}}$ $R e s (φ, z_{2}) = \frac{1}{4 {(e^{\frac{i π}{3}})}^{3} + 6 {(e^{\frac{i π}{3}})}^{5}} = \frac{1}{- 4 + 6 e^{\frac{i 5 π}{3}}}$ $R e s (φ, z_{4}) = \frac{1}{4 {(e^{\frac{i 2 π}{3}})}^{3} + 6 {(e^{\frac{i 2 π}{3}})}^{5}} = \frac{1}{4 + 6 e^{\frac{i 10 π}{3}}}$ $= . . . .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum