
Question Number 40143 by maxmathsup by imad last updated on 16/Jul/18
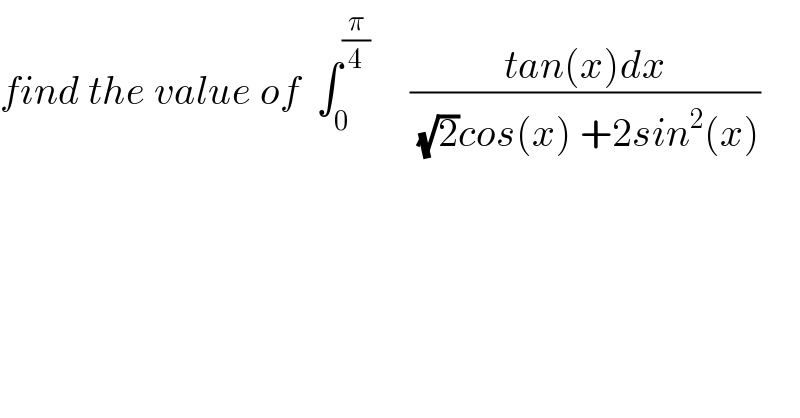
$${find}\:{the}\:{value}\:{of}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\:\frac{{tan}\left({x}\right){dx}}{\sqrt{\mathrm{2}}{cos}\left({x}\right)\:+\mathrm{2}{sin}^{\mathrm{2}} \left({x}\right)} \\ $$
Commented by math khazana by abdo last updated on 22/Jul/18
![I = ∫_0 ^(π/4) ((sinx)/(cosx((√2)cosx +2(1−cos^2 x)))dx changement cosx =t give I = ∫_1 ^(1/(√2)) ((√(1−t^2 ))/(t{(√2)t +2−2t^2 })) ((−dt)/(√(1−t^2 ))) = ∫_(1/(√2)) ^1 (dt/(t{−2t^2 +(√2)t +2})) let decompose F(t) = (1/(t{ −2t^2 +(√2) t+2})) Δ^ =2−4(−2)2=2 +16 =18 ⇒ t_1 =((−(√2) +3(√2))/(−2)) =(((√2) −3(√2))/2)=−(√2) t_2 =((−(√2) −3(√2))/(−2)) =(((√2)+3(√2))/2)=2(√2) F(t) = (1/(−2t(t−t_1 )(t−t_2 ))) =(a/t) +(b/(t−t_1 )) +(c/(t−t_2 )) a=lim_(t→0) tF(t)=(1/2) b =lim_(t→t_1 ) (t−t_1 )F(t) = ((−1)/(2t_1 (t_1 −t_2 ))) = ((−1)/(−2(√2)(−3(√2)))) = (1/(12)) c = lim_(t→t_2 ) (t−t_2 )F(t)= ((−1)/(2t_2 (t_2 −t_1 ))) =((−1)/(4(√2)(3(√2)))) =((−1)/(24)) ⇒ F(t) = (1/(2t)) +(1/(12(t−t_1 ))) −(1/(24(t−t_2 ))) ⇒ ∫_(1/(√2)) ^1 F(t)dt =(1/2) ∫_(1/(√2)) ^1 (dt/t) +(1/(12)) ∫_(1/(√2)) ^1 (dt/(t+(√2))) −(1/(24)) ∫_(1/(√2)) ^1 (dt/(t−2(√2))) =(1/2)[ln∣t∣]_(1/(√2)) ^1 +(1/(12))[ln∣t+(√2)∣]_(1/(√2)) ^1 −(1/(24))[ln∣t−2(√2)∣]_(1/(√2)) ^1 =((ln((√(2))))/2) +(1/(12)){ln(1+(√2))−ln((√2) +(1/(√2)))} −(1/(24)){ln(2(√2) −1)−ln(2(√2) −(1/(√2)))} .](Q40458.png)
$${I}\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\:\:\:\frac{{sinx}}{{cosx}\left(\sqrt{\mathrm{2}}{cosx}\:+\mathrm{2}\left(\mathrm{1}−{cos}^{\mathrm{2}} {x}\right)\right.}{dx} \\ $$$${changement}\:{cosx}\:={t}\:{give} \\ $$$${I}\:=\:\int_{\mathrm{1}} ^{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} \:\:\:\:\:\:\frac{\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }}{{t}\left\{\sqrt{\mathrm{2}}{t}\:+\mathrm{2}−\mathrm{2}{t}^{\mathrm{2}} \right\}}\:\frac{−{dt}}{\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }} \\ $$$$=\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} ^{\mathrm{1}} \:\:\:\:\:\frac{{dt}}{{t}\left\{−\mathrm{2}{t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}{t}\:+\mathrm{2}\right\}}\:{let}\:{decompose} \\ $$$${F}\left({t}\right)\:=\:\frac{\mathrm{1}}{{t}\left\{\:−\mathrm{2}{t}^{\mathrm{2}} \:+\sqrt{\mathrm{2}}\:{t}+\mathrm{2}\right\}} \\ $$$$\Delta^{} =\mathrm{2}−\mathrm{4}\left(−\mathrm{2}\right)\mathrm{2}=\mathrm{2}\:+\mathrm{16}\:=\mathrm{18}\:\:\Rightarrow \\ $$$${t}_{\mathrm{1}} =\frac{−\sqrt{\mathrm{2}}\:+\mathrm{3}\sqrt{\mathrm{2}}}{−\mathrm{2}}\:=\frac{\sqrt{\mathrm{2}}\:−\mathrm{3}\sqrt{\mathrm{2}}}{\mathrm{2}}=−\sqrt{\mathrm{2}} \\ $$$${t}_{\mathrm{2}} =\frac{−\sqrt{\mathrm{2}}\:−\mathrm{3}\sqrt{\mathrm{2}}}{−\mathrm{2}}\:=\frac{\sqrt{\mathrm{2}}+\mathrm{3}\sqrt{\mathrm{2}}}{\mathrm{2}}=\mathrm{2}\sqrt{\mathrm{2}} \\ $$$${F}\left({t}\right)\:=\:\frac{\mathrm{1}}{−\mathrm{2}{t}\left({t}−{t}_{\mathrm{1}} \right)\left({t}−{t}_{\mathrm{2}} \right)}\:=\frac{{a}}{{t}}\:+\frac{{b}}{{t}−{t}_{\mathrm{1}} }\:+\frac{{c}}{{t}−{t}_{\mathrm{2}} } \\ $$$${a}={lim}_{{t}\rightarrow\mathrm{0}} {tF}\left({t}\right)=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${b}\:={lim}_{{t}\rightarrow{t}_{\mathrm{1}} } \:\:\:\left({t}−{t}_{\mathrm{1}} \right){F}\left({t}\right)\:=\:\frac{−\mathrm{1}}{\mathrm{2}{t}_{\mathrm{1}} \left({t}_{\mathrm{1}} −{t}_{\mathrm{2}} \right)} \\ $$$$=\:\frac{−\mathrm{1}}{−\mathrm{2}\sqrt{\mathrm{2}}\left(−\mathrm{3}\sqrt{\mathrm{2}}\right)}\:=\:\frac{\mathrm{1}}{\mathrm{12}} \\ $$$${c}\:=\:{lim}_{{t}\rightarrow{t}_{\mathrm{2}} } \:\:\:\left({t}−{t}_{\mathrm{2}} \right){F}\left({t}\right)=\:\frac{−\mathrm{1}}{\mathrm{2}{t}_{\mathrm{2}} \left({t}_{\mathrm{2}} −{t}_{\mathrm{1}} \right)}\:=\frac{−\mathrm{1}}{\mathrm{4}\sqrt{\mathrm{2}}\left(\mathrm{3}\sqrt{\mathrm{2}}\right)} \\ $$$$=\frac{−\mathrm{1}}{\mathrm{24}}\:\Rightarrow \\ $$$${F}\left({t}\right)\:=\:\frac{\mathrm{1}}{\mathrm{2}{t}}\:\:+\frac{\mathrm{1}}{\mathrm{12}\left({t}−{t}_{\mathrm{1}} \right)}\:−\frac{\mathrm{1}}{\mathrm{24}\left({t}−{t}_{\mathrm{2}} \right)}\:\Rightarrow \\ $$$$\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} ^{\mathrm{1}} {F}\left({t}\right){dt}\:=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} ^{\mathrm{1}} \:\frac{{dt}}{{t}}\:+\frac{\mathrm{1}}{\mathrm{12}}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} ^{\mathrm{1}} \:\:\frac{{dt}}{{t}+\sqrt{\mathrm{2}}}\:−\frac{\mathrm{1}}{\mathrm{24}}\:\int_{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} ^{\mathrm{1}} \:\frac{{dt}}{{t}−\mathrm{2}\sqrt{\mathrm{2}}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\left[{ln}\mid{t}\mid\right]_{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} ^{\mathrm{1}} \:\:+\frac{\mathrm{1}}{\mathrm{12}}\left[{ln}\mid{t}+\sqrt{\mathrm{2}}\mid\right]_{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} ^{\mathrm{1}} \:−\frac{\mathrm{1}}{\mathrm{24}}\left[{ln}\mid{t}−\mathrm{2}\sqrt{\mathrm{2}}\mid\right]_{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} ^{\mathrm{1}} \\ $$$$=\frac{{ln}\left(\sqrt{\left.\mathrm{2}\right)}\right.}{\mathrm{2}}\:+\frac{\mathrm{1}}{\mathrm{12}}\left\{{ln}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)−{ln}\left(\sqrt{\mathrm{2}}\:+\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\right)\right\} \\ $$$$−\frac{\mathrm{1}}{\mathrm{24}}\left\{{ln}\left(\mathrm{2}\sqrt{\mathrm{2}}\:−\mathrm{1}\right)−{ln}\left(\mathrm{2}\sqrt{\mathrm{2}}\:−\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\right)\right\}\:. \\ $$$$ \\ $$
