
Question Number 4027 by Rasheed Soomro last updated on 27/Dec/15

$${Let}\:\mathbb{A}\:{denotes}\:{the}\:{Set}\:{of}\:{Algebraic}\:{Numbers} \\ $$$${and}\:\:\mathbb{T}\:\:\:{the}\:{Set}\:{of}\:{Trancedental}\:{Numbers}. \\ $$$${Discuss}\:{the}\:{following}: \\ $$$$\bullet{Are}\:\mathbb{A}\:{and}\:\mathbb{T}\:\:\boldsymbol{{closed}}\:{with}\:{respect}\:{to}\:\: \\ $$$$\boldsymbol{{addition}}\:{and}\:\boldsymbol{{multiplication}}\:? \\ $$$$\bullet{Are}\:\mathbb{A}−\left\{\mathrm{0}\right)\:\:{and}\:\mathbb{T}\:\:\boldsymbol{{closed}}\:{with}\:{respect}\:{to}\:\: \\ $$$$\boldsymbol{{division}}? \\ $$
Commented by Yozzii last updated on 27/Dec/15
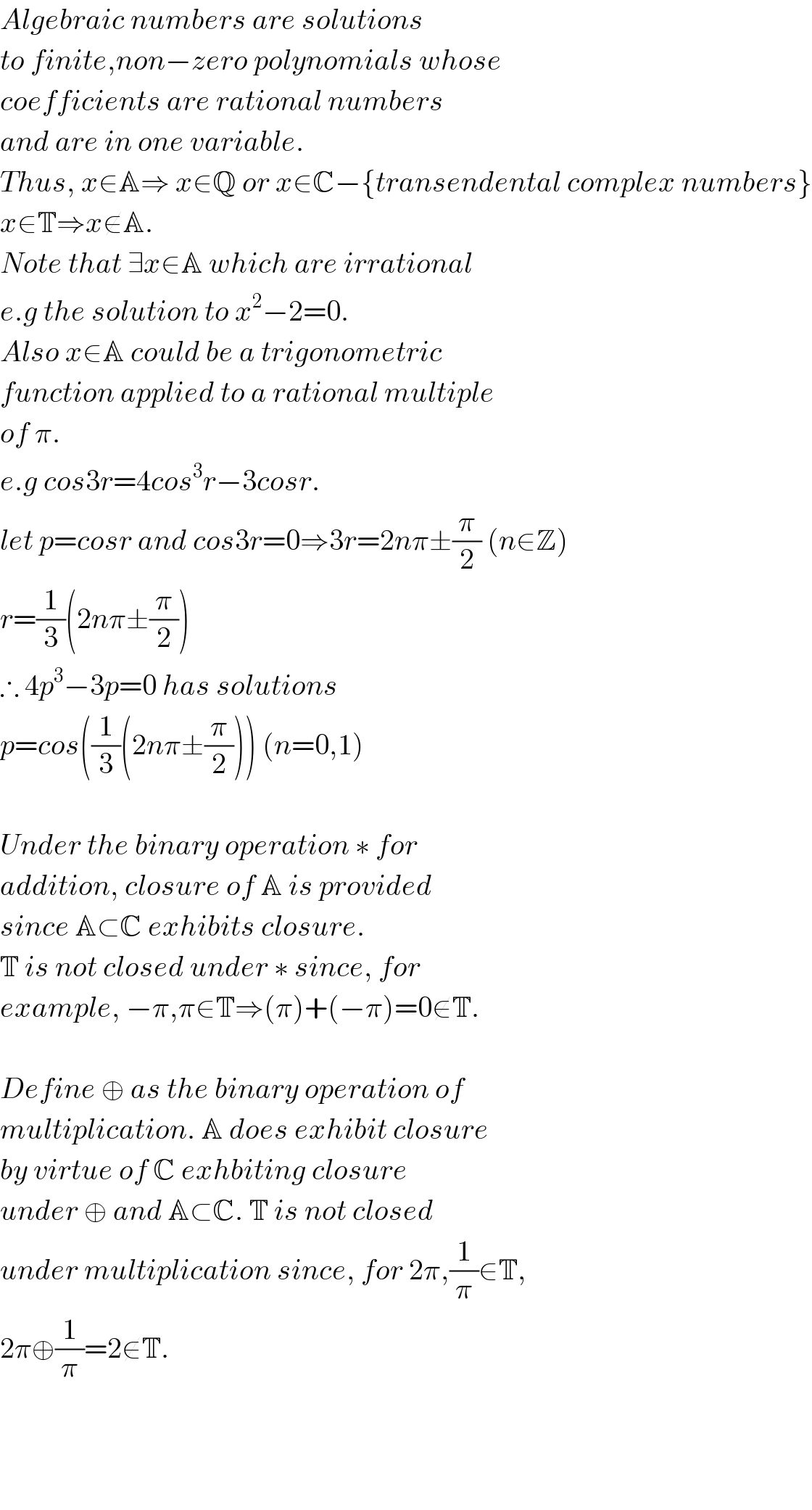
$${Algebraic}\:{numbers}\:{are}\:{solutions} \\ $$$${to}\:{finite},{non}−{zero}\:{polynomials}\:{whose} \\ $$$${coefficients}\:{are}\:{rational}\:{numbers} \\ $$$${and}\:{are}\:{in}\:{one}\:{variable}. \\ $$$${Thus},\:{x}\in\mathbb{A}\Rightarrow\:{x}\in\mathbb{Q}\:{or}\:{x}\in\mathbb{C}−\left\{{transendental}\:{complex}\:{numbers}\right\} \\ $$$${x}\in\mathbb{T}\Rightarrow{x}\notin\mathbb{A}.\: \\ $$$${Note}\:{that}\:\exists{x}\in\mathbb{A}\:{which}\:{are}\:{irrational} \\ $$$${e}.{g}\:{the}\:{solution}\:{to}\:{x}^{\mathrm{2}} −\mathrm{2}=\mathrm{0}. \\ $$$${Also}\:{x}\in\mathbb{A}\:{could}\:{be}\:{a}\:{trigonometric} \\ $$$${function}\:{applied}\:{to}\:{a}\:{rational}\:{multiple} \\ $$$${of}\:\pi. \\ $$$${e}.{g}\:{cos}\mathrm{3}{r}=\mathrm{4}{cos}^{\mathrm{3}} {r}−\mathrm{3}{cosr}. \\ $$$${let}\:{p}={cosr}\:{and}\:{cos}\mathrm{3}{r}=\mathrm{0}\Rightarrow\mathrm{3}{r}=\mathrm{2}{n}\pi\pm\frac{\pi}{\mathrm{2}}\:\left({n}\in\mathbb{Z}\right) \\ $$$${r}=\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{2}{n}\pi\pm\frac{\pi}{\mathrm{2}}\right) \\ $$$$\therefore\:\mathrm{4}{p}^{\mathrm{3}} −\mathrm{3}{p}=\mathrm{0}\:{has}\:{solutions} \\ $$$${p}={cos}\left(\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{2}{n}\pi\pm\frac{\pi}{\mathrm{2}}\right)\right)\:\left({n}=\mathrm{0},\mathrm{1}\right) \\ $$$$ \\ $$$${Under}\:{the}\:{binary}\:{operation}\:\ast\:{for} \\ $$$${addition},\:{closure}\:{of}\:\mathbb{A}\:{is}\:{provided} \\ $$$${since}\:\mathbb{A}\subset\mathbb{C}\:{exhibits}\:{closure}. \\ $$$$\mathbb{T}\:{is}\:{not}\:{closed}\:{under}\:\ast\:{since},\:{for} \\ $$$${example},\:−\pi,\pi\in\mathbb{T}\Rightarrow\left(\pi\right)+\left(−\pi\right)=\mathrm{0}\notin\mathbb{T}. \\ $$$$ \\ $$$${Define}\:\oplus\:{as}\:{the}\:{binary}\:{operation}\:{of} \\ $$$${multiplication}.\:\mathbb{A}\:{does}\:{exhibit}\:{closure} \\ $$$${by}\:{virtue}\:{of}\:\mathbb{C}\:{exhbiting}\:{closure} \\ $$$${under}\:\oplus\:{and}\:\mathbb{A}\subset\mathbb{C}.\:\mathbb{T}\:{is}\:{not}\:{closed} \\ $$$${under}\:{multiplication}\:{since},\:{for}\:\mathrm{2}\pi,\frac{\mathrm{1}}{\pi}\in\mathbb{T}, \\ $$$$\mathrm{2}\pi\oplus\frac{\mathrm{1}}{\pi}=\mathrm{2}\notin\mathbb{T}. \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by Rasheed Soomro last updated on 27/Dec/15

$$\mathcal{EX}_{\mathcal{PLANATION}} ^{\mathcal{CELLENT}} \:\:!!! \\ $$
