Math Question and Answers


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 40306 by behi83417@gmail.com last updated on 19/Jul/18

Commented by MJS last updated on 20/Jul/18

$can we show the following ?$ $\frac{3}{2} ⩽ \frac{x^{2} + y z}{y^{2} + z^{2}} + \frac{y^{2} + x z}{x^{2} + z^{2}} + \frac{z^{2} + x y}{x^{2} + y^{2}} ⩽ \frac{21}{10}$
Commented by MJS last updated on 20/Jul/18
$f(x,y,z)=((x^2 +yz)/(y^2 +z^2 ))+((y^2 +xz)/(x^2 +z^2 ))+((z^2 +xy)/(x^2 +y^2 )) z=−(x+y) f(x,y,−(x+y))= =g(x,y)=((3(x^6 +y^6 +3xy(x^4 +y^4 )+8x^2 y^2 (x^2 +y^2 )+11x^3 y^3 ))/((x^2 +y^2 )((x+y)^2 +x^2 )((x+y)^2 +y^2 ))) (d/dx)[g(x,y)]=−((15xy^2 (2(x^8 −y^8 )+9xy(x^6 −y^6 )+16x^2 y^2 (x^4 −y^4 )+14x^3 y^3 (x^2 −y^2 )))/((x^2 +y^2 )^2 ((x+_ y)^2 +x^2 )^2 ((x+y)^2 +y^2 )^2 )) (d/dx)[g(x,y)]=0 ⇒ x=0 ∨ y=0 ∨ 2(x^8 −y^8 )+9xy(x^6 −y^6 )+16x^2 y^2 (x^4 −y^4 )+14x^3 y^3 (x^2 −y^2 )=0 x={−2y; −y; −(y/2); 0; y; −(y/2)(1+i(√3)); −(y/2)(1−i(√3))} f(x, y, z)={((21)/(10)); (3/2); ((21)/(10)); (3/2); ((21)/(10)); −6; −6} so these are the minima and maxima of f(x, y, z)$
$f (x, y, z) = \frac{x^{2} + y z}{y^{2} + z^{2}} + \frac{y^{2} + x z}{x^{2} + z^{2}} + \frac{z^{2} + x y}{x^{2} + y^{2}}$ $z = - (x + y)$ $f (x, y, - (x + y)) =$ $= g (x, y) = \frac{3 (x^{6} + y^{6} + 3 x y (x^{4} + y^{4}) + 8 x^{2} y^{2} (x^{2} + y^{2}) + 11 x^{3} y^{3})}{(x^{2} + y^{2}) ({(x + y)}^{2} + x^{2}) ({(x + y)}^{2} + y^{2})}$ $\frac{d}{d x} [g (x, y)] = - \frac{15 {x y}^{2} (2 (x^{8} - y^{8}) + 9 x y (x^{6} - y^{6}) + 16 x^{2} y^{2} (x^{4} - y^{4}) + 14 x^{3} y^{3} (x^{2} - y^{2}))}{{(x^{2} + y^{2})}^{2} {({(x +_{} y)}^{2} + x^{2})}^{2} {({(x + y)}^{2} + y^{2})}^{2}}$ $\frac{d}{d x} [g (x, y)] = 0 \Rightarrow x = 0 \lor y = 0 \lor$ $2 (x^{8} - y^{8}) + 9 x y (x^{6} - y^{6}) + 16 x^{2} y^{2} (x^{4} - y^{4}) + 14 x^{3} y^{3} (x^{2} - y^{2}) = 0$ $x = {- 2 y; - y; - \frac{y}{2}; 0; y; - \frac{y}{2} (1 + i \sqrt{3}); - \frac{y}{2} (1 - i \sqrt{3})}$ $f (x, y, z) = {\frac{21}{10}; \frac{3}{2}; \frac{21}{10}; \frac{3}{2}; \frac{21}{10}; - 6; - 6}$ $so these are the minima and maxima of f (x, y, z)$
	Answered by tanmay.chaudhury50@gmail.com last updated on 19/Jul/18

	$k = \frac{x y}{x^{2} + y^{2}} + \frac{y z}{y^{2} + z^{2}} + \frac{x z}{x^{2} + z^{2}} + \frac{z^{2}}{x^{2} + y^{2}} + \frac{x^{2}}{y^{2} + z^{2}} + \frac{y^{2}}{x^{2} + z^{2}}$ $\frac{x^{2} + y^{2}}{2} ⩾ \sqrt{x^{2} y^{2}}$ $\frac{x^{2} + y^{2}}{x y} ⩾ 2 s o \frac{x y}{x^{2} + y^{2}} ⩽ \frac{1}{2}$ $\frac{z^{2}}{x^{2} + y^{2}} z = - (x + y) s o z^{2} = x^{2} + y^{2} + 2 x y$ $\frac{z^{2}}{x^{2} + y^{2}} = 1 + \frac{2 x y}{x^{2} + y^{2}}$ $1 + \frac{2 x y}{x^{2} + y^{2}} ⩽ 2$ $k ⩽ \frac{1}{2} + \frac{1}{2} + \frac{1}{2} + 2 + 2 + 2$ $k ⩽ \frac{15}{2}$
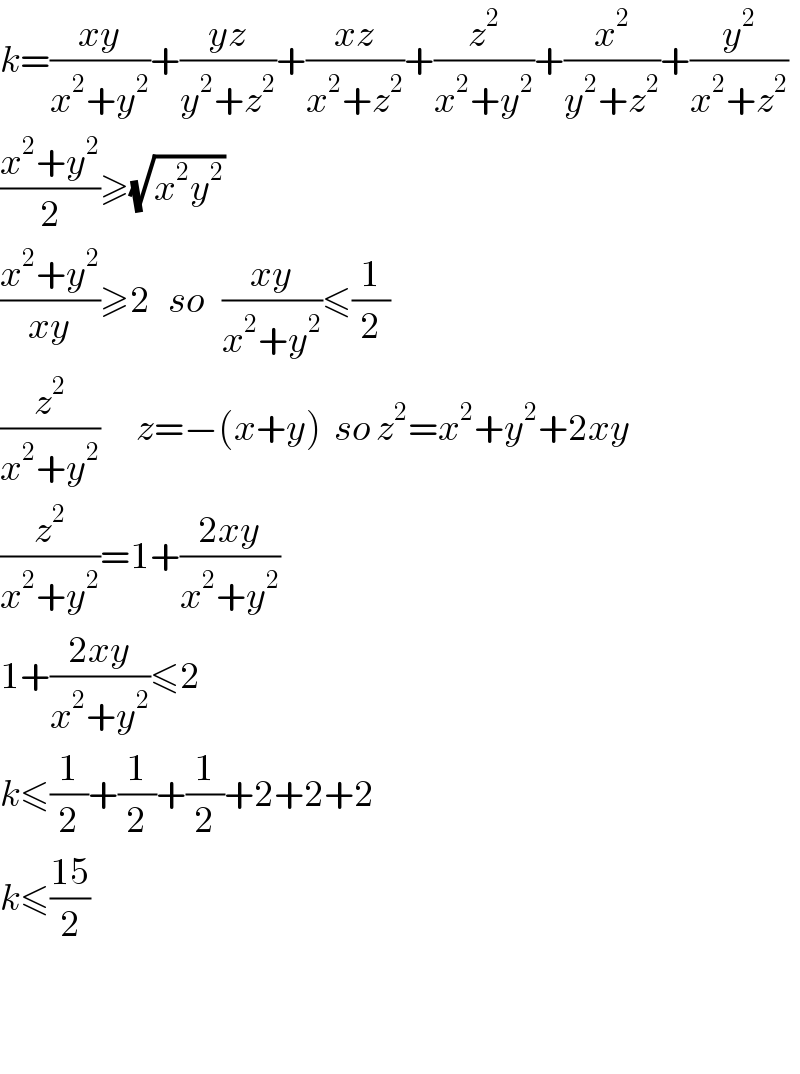
	Answered by math1967 last updated on 19/Jul/18

	$x, y, z \in R ∴ {(x - y)}^{2} ⩾ 0$ ${(y - z)}^{2} ⩾ 0, {(z - x)}^{2} ⩾ 0$ $∴ x^{2} + y^{2} ⩾ 2 x y . . . . . . . 1$ $F r o m 1 \frac{1}{x^{2} + y^{2}} ⩽ \frac{1}{2 x y}$ $\frac{x y}{x^{2} + y^{2}} ⩽ \frac{1}{2} . . . . . . (2)$ $A g a i n x + y + z = 0$ $\Rightarrow x^{2} + y^{2} + 2 x y = z^{2}$ $∴ x^{2} + y^{2} + 3 x y = x y + z^{2}$ $F r o m (2) \frac{3 x y}{x^{2} + y^{2}} ⩽ \frac{3}{2}$ $1 + \frac{3 x y}{x^{2} + y^{2}} ⩽ \frac{5}{2}$ $\frac{x^{2} + y^{2} + 3 x y}{x^{2} + y^{2}} ⩽ \frac{5}{2}$ $\frac{x y + z^{2}}{x^{2} + y^{2}} ⩽ \frac{5}{2} [x^{2} + y^{2} + 3 x y = x y + z^{2}]$ $s i m i l a r l y \frac{y z + x^{2}}{y^{2} + z^{2}} ⩽ \frac{5}{2}$ $\frac{z x + y^{2}}{z^{2} + x^{2}} ⩽ \frac{5}{2}$ $∴ \frac{x y + z^{2}}{x^{2} + y^{2}} + \frac{y z + x^{2}}{y^{2} + z^{2}} + \frac{z x + y^{2}}{z^{2} + x^{2}} ⩽ \frac{15}{2}$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum