Math Question and Answers


Question and Answers Forum

All Questions Topic List
Mechanics Questions
Previous in All Question Next in All Question
Previous in Mechanics Next in Mechanics
Question Number 41255 by ajfour last updated on 04/Aug/18

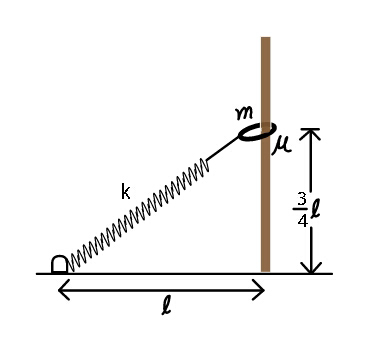
Commented by ajfour last updated on 04/Aug/18

$T h e n a t u r a l l e n g t h o f s p r i n g i s$ $l a n d i f r e a l e a s e d a s s h o w n, f i n d$ $s p e e d o f r i n g a s i t r e a c h e s t h e$ $g r o u n d . μ i s t h e f r i c t i o n c o e f f i c i e n t$ $b e t w e e n v e r t i c a l r o d a n d t h e r i n g .$
	Answered by ajfour last updated on 05/Aug/18

	$A t a n i n t e r m e d i a t e p o s i t i o n, l e t$ $t h e a n g l e o f s p r i n g l i n e w i t h$ $h o r i z o n t a l b e θ .$ $N o r m a l r e a c t i o n o n r i n g$ $N = k x \cos θ$ $x = l (\sec θ - 1)$ $f r i c t i o n f = μ k l (1 - \cos θ)$ $h e i g h t o f r i n g i s y = l \tan θ$ $d y = l \sec^{2} θ d θ$ $W_{f} = \int_{α}^{0} μ {k l}^{2} (1 - \cos θ) \sec^{2} θ d θ$ $w h e r e α = \tan^{- 1} (\frac{3}{4})$ $\Rightarrow W_{f} = - μ {k l}^{2} \int_{0}^{α} (\sec^{2} θ - \sec θ) d θ$ $= - μ {k l}^{2} [\tan α - \ln (\sec α + \tan α)]$ $= - μ {k l}^{2} [\frac{3}{4} - \ln (\frac{5}{4} + \frac{3}{4})]$ $= - μ {k l}^{2} (\frac{3}{4} - \ln 2)$ $\frac{1}{2} {m v}^{2} = m g (\frac{3 l}{4}) + \frac{1}{2} k {[l \sec α - l]}^{2}$ $- μ {k l}^{2} (\frac{3}{4} - \ln 2)$ $v^{2} = \frac{3 g l}{2} + \frac{{k l}^{2}}{16 m} - \frac{μ {k l}^{2}}{m} (\frac{3}{2} - 2 \ln 2)$ $v = \sqrt{\frac{3 g l}{2} + \frac{{k l}^{2}}{16 m} - \frac{μ {k l}^{2}}{m} (\frac{3}{2} - 2 \ln 2)} .$
	Commented by MrW3 last updated on 05/Aug/18

	$g r e a t j o b!$
	Answered by tanmay.chaudhury50@gmail.com last updated on 05/Aug/18
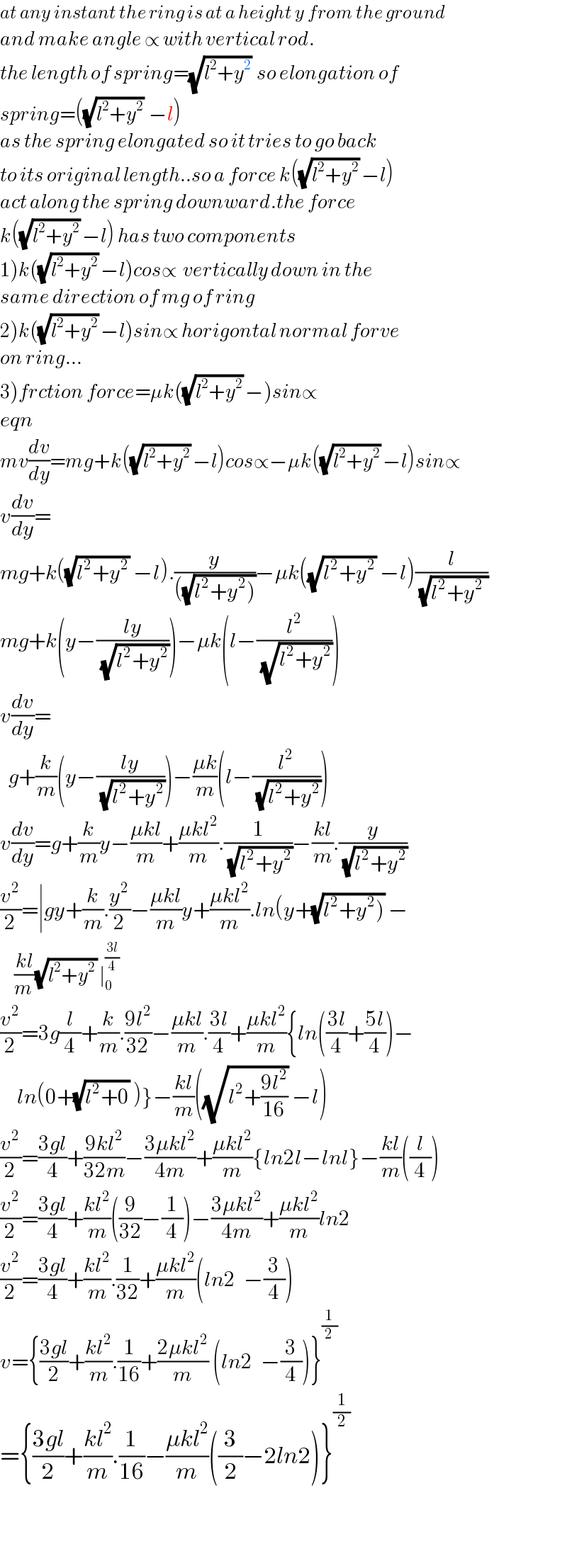
	$at any instant the ring is at a height y from the ground and make angle ∝ with vertical rod. the length of spring=(√(l^2 +y_ ^2 )) so elongation of spring=((√(l^2 +y^2 )) −l) as the spring elongated so it tries to go back to its original length..so a force k((√(l^2 +y^2 )) −l) act along the spring downward.the force k((√(l^2 +y^2 )) −l) has two components 1)k((√(l^2 +y^2 )) −l)cos∝ vertically down in the same direction of mg of ring 2)k((√(l^2 +y^2 )) −l)sin∝ horigontal normal forve on ring... 3)frction force=μk((√(l^2 +y^2 )) −)sin∝ eqn mv(dv/dy)=mg+k((√(l^2 +y^2 )) −l)cos∝−μk((√(l^2 +y^2 )) −l)sin∝ v(dv/dy)= mg+k((√(l^2 +y^2 )) −l).(y/(((√(l^2 +y^2 )))))−μk((√(l^2 +y_ ^2 )) −l)(l/(√(l^2 +y^2 ))) mg+k(y−((ly)/(√(l_ ^2 +y^2 ))))−μk(l−(l^2 /(√(l^2 +y^2 _ )))) v(dv/dy)= g+(k/m)(y−((ly)/(√(l^2 +y^2 ))))−((μk)/m)(l−(l^2 /(√(l^2 +y^2 )))) v(dv/dy)=g+(k/m)y−((μkl)/m)+((μkl^2 )/m).(1/(√(l^2 +y^2 )))−((kl)/m).(y/(√(l^2 +y^2 ))) (v^2 /2)=∣gy+(k/m).(y^2 /2)−((μkl)/m)y+((μkl^2 )/m).ln(y+(√(l^2 +y^2 ))) − ((kl)/(m ))(√(l^2 +y^(2 ) )) ∣_0 ^((3l)/4) (v^2 /2)=3g(l/4)+(k/m).((9l^2 )/(32))−((μkl)/m).((3l)/4)+((μkl^2 )/m){ln(((3l)/4)+((5l)/4))− ln(0+(√(l^2 +0)) )}−((kl)/m)((√(l^2 +((9l^2 )/(16)))) −l) (v^2 /2)=((3gl)/4)+((9kl^2 )/(32m))−((3μkl^2 )/(4m))+((μkl^2 )/m){ln2l−lnl}−((kl)/m)((l/4)) (v^2 /2)=((3gl)/4)+((kl^2 )/m)((9/(32))−(1/4))−((3μkl^2 )/(4m))+((μkl^2 )/m)ln2 (v^2 /2)=((3gl)/4)+((kl^2 )/m).(1/(32))+((μkl^2 )/m)(ln2 −(3/4)) v={((3gl)/2)+((kl^2 )/m).(1/(16))+((2μkl^2 )/m) (ln2 −(3/4))}^(1/2) ={((3gl)/2)+((kl^2 )/m).(1/(16))−((μkl^2 )/m)((3/2)−2ln2)}^(1/2)$
	$a t a n y i n s t a n t t h e r i n g i s a t a h e i g h t y f r o m t h e g r o u n d$ $a n d m a k e a n g l e \propto w i t h v e r t i c a l r o d .$ $t h e l e n g t h o f s p r i n g = \sqrt{l^{2} + y_{}^{2}} s o e l o n g a t i o n o f$ $s p r i n g = (\sqrt{l^{2} + y^{2}} - l)$ $a s t h e s p r i n g e l o n g a t e d s o i t t r i e s t o g o b a c k$ $t o i t s o r i g i n a l l e n g t h . . s o a f o r c e k (\sqrt{l^{2} + y^{2}} - l)$ $a c t a l o n g t h e s p r i n g d o w n w a r d . t h e f o r c e$ $k (\sqrt{l^{2} + y^{2}} - l) h a s t w o c o m p o n e n t s$ $1) k (\sqrt{l^{2} + y^{2}} - l) c o s \propto v e r t i c a l l y d o w n i n t h e$ $s a m e d i r e c t i o n o f m g o f r i n g$ $2) k (\sqrt{l^{2} + y^{2}} - l) s i n \propto h o r i g o n t a l n o r m a l f o r v e$ $o n r i n g . . .$ $3) f r c t i o n f o r c e = μ k (\sqrt{l^{2} + y^{2}} -) s i n \propto$ $e q n$ $m v \frac{d v}{d y} = m g + k (\sqrt{l^{2} + y^{2}} - l) c o s \propto - μ k (\sqrt{l^{2} + y^{2}} - l) s i n \propto$ $v \frac{d v}{d y} =$ $m g + k (\sqrt{l^{2} + y^{2}} - l) . \frac{y}{(\sqrt{l^{2} + y^{2})}} - μ k (\sqrt{l^{2} + y_{}^{2}} - l) \frac{l}{\sqrt{l^{2} + y^{2}}}$ $m g + k (y - \frac{l y}{\sqrt{l_{}^{2} + y^{2}}}) - μ k (l - \frac{l^{2}}{\sqrt{l^{2} + {\underset{}{y}}^{2}}})$ $v \frac{d v}{d y} =$ $g + \frac{k}{m} (y - \frac{l y}{\sqrt{l^{2} + y^{2}}}) - \frac{μ k}{m} (l - \frac{l^{2}}{\sqrt{l^{2} + y^{2}}})$ $v \frac{d v}{d y} = g + \frac{k}{m} y - \frac{μ k l}{m} + \frac{μ {k l}^{2}}{m} . \frac{1}{\sqrt{l^{2} + y^{2}}} - \frac{k l}{m} . \frac{y}{\sqrt{l^{2} + y^{2}}}$ $\frac{v^{2}}{2} =∣ g y + \frac{k}{m} . \frac{y^{2}}{2} - \frac{μ k l}{m} y + \frac{μ {k l}^{2}}{m} . l n (y + \sqrt{l^{2} + y^{2})} -$ $\frac{k l}{m} \sqrt{l^{2} + y^{2}} ∣_{0}^{\frac{3 l}{4}}$ $\frac{v^{2}}{2} = 3 g \frac{l}{4} + \frac{k}{m} . \frac{9 l^{2}}{32} - \frac{μ k l}{m} . \frac{3 l}{4} + \frac{μ {k l}^{2}}{m} {l n (\frac{3 l}{4} + \frac{5 l}{4}) -$ $l n (0 + \sqrt{l^{2} + 0})} - \frac{k l}{m} (\sqrt{l^{2} + \frac{9 l^{2}}{16}} - l)$ $\frac{v^{2}}{2} = \frac{3 g l}{4} + \frac{9 {k l}^{2}}{32 m} - \frac{3 μ {k l}^{2}}{4 m} + \frac{μ {k l}^{2}}{m} {l n 2 l - l n l} - \frac{k l}{m} (\frac{l}{4})$ $\frac{v^{2}}{2} = \frac{3 g l}{4} + \frac{{k l}^{2}}{m} (\frac{9}{32} - \frac{1}{4}) - \frac{3 μ {k l}^{2}}{4 m} + \frac{μ {k l}^{2}}{m} l n 2$ $\frac{v^{2}}{2} = \frac{3 g l}{4} + \frac{{k l}^{2}}{m} . \frac{1}{32} + \frac{μ {k l}^{2}}{m} (l n 2 - \frac{3}{4})$ $v = {\frac{3 g l}{2} + \frac{{k l}^{2}}{m} . \frac{1}{16} + \frac{2 μ {k l}^{2}}{m} (l n 2 - \frac{3}{4})}^{\frac{1}{2}}$ $= {\frac{3 g l}{2} + \frac{{k l}^{2}}{m} . \frac{1}{16} - \frac{μ {k l}^{2}}{m} (\frac{3}{2} - 2 l n 2)}^{\frac{1}{2}}$
	Commented by MrW3 last updated on 04/Aug/18

	$v e r y n i c e!$
	Commented by MrW3 last updated on 05/Aug/18

	$y o u m e a n t h e a n s w e r f r o m a j f o u r s i r .$ $y o u r a n s w e r a n d h i s a n s w e r a r e i d e n t i c a l .$ $You can't use 'macro parameter character #' in math mode$ $w o r k i n g a b o v e .$
	Commented by tanmay.chaudhury50@gmail.com last updated on 04/Aug/18

	$y e s s i r i h a v e r e c t i f i e d i t . . . y o u r s a m d m y s e l f$ $a n s w e r b e c o m e i d e n t i c a l . . . t h a n k y o u . . y o u h a h e$ $g i v e n y o u r t i m e t o g o t h r o u v h m y p o s t . . .$
	Commented by tanmay.chaudhury50@gmail.com last updated on 05/Aug/18

	$o k s i r l e t m e c h e c k . . .$
	Commented by tanmay.chaudhury50@gmail.com last updated on 05/Aug/18

	$p l s c h e c k$
	Commented by MrW3 last updated on 05/Aug/18

	$You can't use 'macro parameter character #' in math mode$ $s p r i n g = (\sqrt{l^{2} + y^{2}} - l)$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum