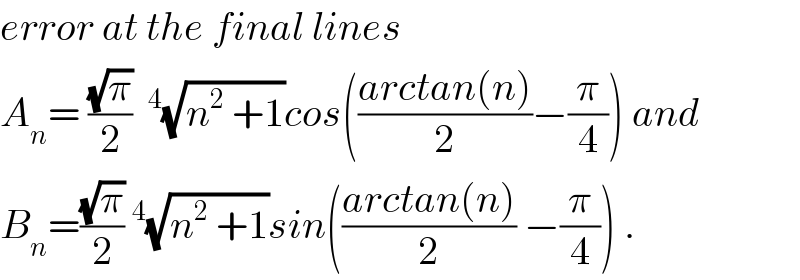let A_n = ∫_0 ^∞ e^(−nx^2 ) cos(x^2 ) dx and B_n =∫_0 ^∞ e^(−nx^2 ) sin(x^2 )dx (n∈ N^★ ) 1) calculate A_n and B_n 2) find lim_(n→+∞) (A_n /B


Question and Answers Forum

All Questions Topic List
Relation and Functions Questions
Previous in All Question Next in All Question
Previous in Relation and Functions Next in Relation and Functions
Question Number 41513 by maxmathsup by imad last updated on 08/Aug/18

$l e t A_{n} = \int_{0}^{\infty} e^{- {n x}^{2}} c o s (x^{2}) d x a n d B_{n} = \int_{0}^{\infty} e^{- {n x}^{2}} s i n (x^{2}) d x (n \in N^{★})$ $1) c a l c u l a t e A_{n} a n d B_{n}$ $2) f i n d {l i m}_{n \to + \infty} \frac{A_{n}}{B_{n}}$
	Answered by alex041103 last updated on 09/Aug/18

	$L e t A_{n} = 2 A_{n} = \int_{- \infty}^{\infty} e^{- {n x}^{2}} c o s (x^{2}) d x a n d$ $B_{n} = 2 B_{n} = \int_{- \infty}^{\infty} e^{- {n x}^{2}} s i n (x^{2}) d x$ $L e t Z_{n} = A_{n} + i B_{n} = \int_{- \infty}^{\infty} e^{- (n - i) x^{2}} d x$ $T h e n Z_{n} = \sqrt{\int_{- \infty}^{\infty} \int_{- \infty}^{\infty} e^{- (n - i) (x^{2} + y^{2})} d x d y}$ $W e c h a n g e t o p o l a r c o o r d i n a t e s$ $Z_{n} = \sqrt{\int_{0}^{2 π} \int_{0}^{\infty} {r e}^{- (n - i) r^{2}} d r d θ}$ $Z_{n}^{2} = - \frac{e^{- (n - i) r^{2}} ∣_{r = 0}^{r = \infty}}{2 n - 2 i} \int_{0}^{2 π} d θ =$ $= \frac{π}{n - i} = Z_{n}^{2} = \frac{π (n + i)}{n^{2} + 1}$ $\Rightarrow Z_{n} = \sqrt{\frac{π}{n^{2} + 1}} \sqrt{n + i}$ $\Rightarrow A_{n} = \frac{1}{2} \sqrt{\frac{π}{n^{2} + 1}} R e (\sqrt{n + i})$ $B_{n} = \frac{1}{2} \sqrt{\frac{π}{n^{2} + 1}} I m (\sqrt{n + i})$
	Commented by alex041103 last updated on 09/Aug/18

	$F o r 2)$ $\frac{A_{n}}{B_{n}} = \frac{R e (\sqrt{n + i})}{I m (\sqrt{n + i})} = c o t (a r g (\sqrt{n + i})) =$ $= c o t (\frac{1}{2} a r c c o t (n))$ $\Rightarrow \underset{n \to \infty}{l i m c o t} (\frac{1}{2} a r c c o t (n)) =$ $= c o t (\frac{1}{2} a r c c o t (+ \infty)) =$ $= c o t (\frac{1}{2} \times 0^{+}) = c o t (0^{+}) = + \infty$ $\Rightarrow \underset{n \to \infty}{l i m} \frac{A_{n}}{B_{n}} = + \infty$
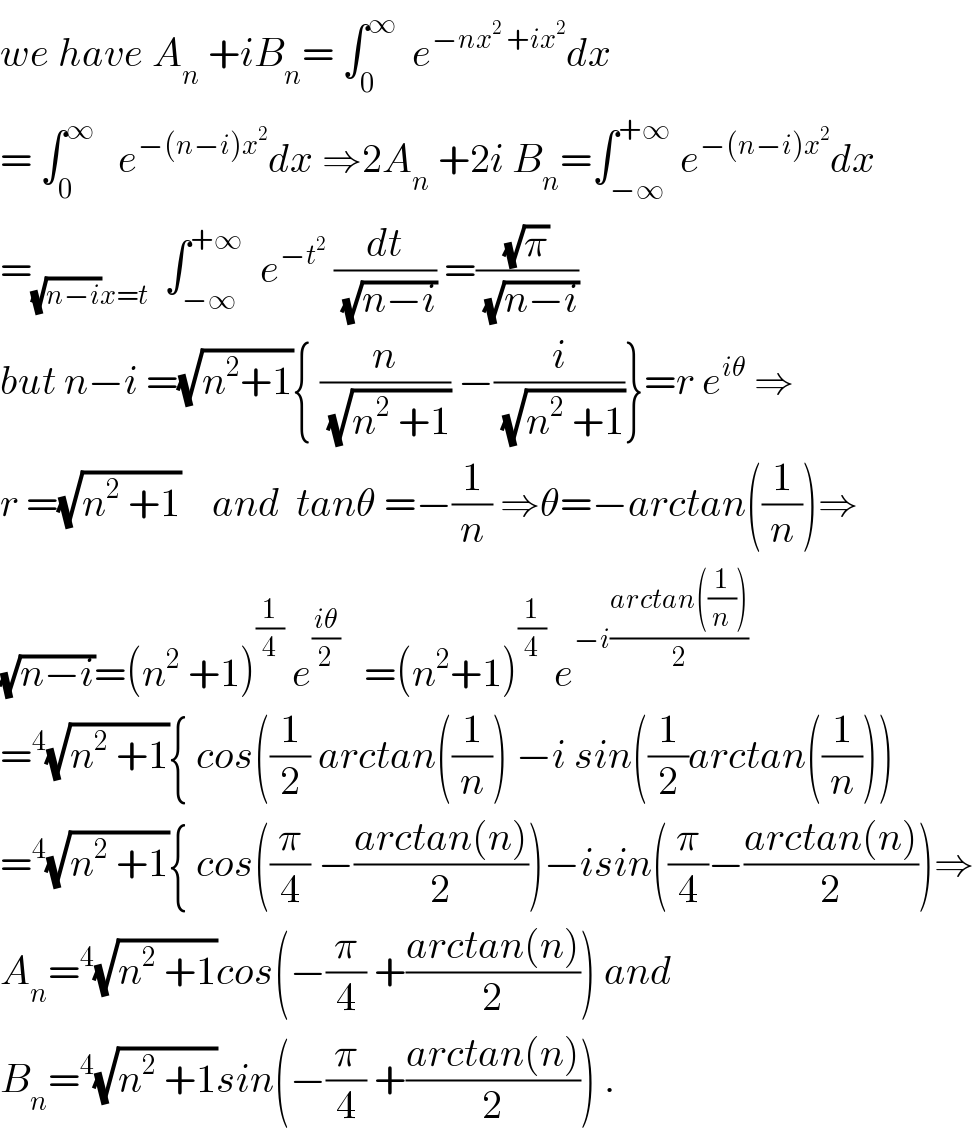
	Answered by math khazana by abdo last updated on 09/Aug/18
	$we have A_n +iB_n = ∫_0 ^∞ e^(−nx^2 +ix^2 ) dx = ∫_0 ^∞ e^(−(n−i)x^2 ) dx ⇒2A_n +2i B_n =∫_(−∞) ^(+∞) e^(−(n−i)x^2 ) dx =_((√(n−i))x=t) ∫_(−∞) ^(+∞) e^(−t^2 ) (dt/(√(n−i))) =((√π)/(√(n−i))) but n−i =(√(n^2 +1)){ (n/(√(n^2 +1))) −(i/(√(n^2 +1)))}=r e^(iθ) ⇒ r =(√(n^2 +1)) and tanθ =−(1/n) ⇒θ=−arctan((1/n))⇒ (√(n−i))=(n^2 +1)^(1/4) e^((iθ)/2) =(n^2 +1)^(1/4) e^(−i((arctan((1/n)))/2)) =^4 (√(n^2 +1)){ cos((1/2) arctan((1/n)) −i sin((1/2)arctan((1/n))) =^4 (√(n^2 +1)){ cos((π/4) −((arctan(n))/2))−isin((π/4)−((arctan(n))/2))⇒ A_n =^4 (√(n^2 +1))cos(−(π/4) +((arctan(n))/2)) and B_n =^4 (√(n^2 +1))sin(−(π/4) +((arctan(n))/2)) .$
	$w e h a v e A_{n} + {i B}_{n} = \int_{0}^{\infty} e^{- {n x}^{2} + {i x}^{2}} d x$ $= \int_{0}^{\infty} e^{- (n - i) x^{2}} d x \Rightarrow 2 A_{n} + 2 i B_{n} = \int_{- \infty}^{+ \infty} e^{- (n - i) x^{2}} d x$ $=_{\sqrt{n - i} x = t} \int_{- \infty}^{+ \infty} e^{- t^{2}} \frac{d t}{\sqrt{n - i}} = \frac{\sqrt{π}}{\sqrt{n - i}}$ $b u t n - i = \sqrt{n^{2} + 1} {\frac{n}{\sqrt{n^{2} + 1}} - \frac{i}{\sqrt{n^{2} + 1}}} = r e^{i θ} \Rightarrow$ $r = \sqrt{n^{2} + 1} a n d t a n θ = - \frac{1}{n} \Rightarrow θ = - a r c t a n (\frac{1}{n}) \Rightarrow$ $\sqrt{n - i} = {(n^{2} + 1)}^{\frac{1}{4}} e^{\frac{i θ}{2}} = {(n^{2} + 1)}^{\frac{1}{4}} e^{- i \frac{a r c t a n (\frac{1}{n})}{2}}$ $=^{4} \sqrt{n^{2} + 1} {c o s (\frac{1}{2} a r c t a n (\frac{1}{n}) - i s i n (\frac{1}{2} a r c t a n (\frac{1}{n}))$ $=^{4} \sqrt{n^{2} + 1} {c o s (\frac{π}{4} - \frac{a r c t a n (n)}{2}) - i s i n (\frac{π}{4} - \frac{a r c t a n (n)}{2}) \Rightarrow$ $A_{n} =^{4} \sqrt{n^{2} + 1} c o s (- \frac{π}{4} + \frac{a r c t a n (n)}{2}) a n d$ $B_{n} =^{4} \sqrt{n^{2} + 1} s i n (- \frac{π}{4} + \frac{a r c t a n (n)}{2}) .$
	Commented by math khazana by abdo last updated on 10/Aug/18

	$e r r o r a t t h e f i n a l l i n e s$ $A_{n} = \frac{\sqrt{π}}{2}^{4} \sqrt{n^{2} + 1} c o s (\frac{a r c t a n (n)}{2} - \frac{π}{4}) a n d$ $B_{n} = \frac{\sqrt{π}}{2}^{4} \sqrt{n^{2} + 1} s i n (\frac{a r c t a n (n)}{2} - \frac{π}{4}) .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum