Question and Answers Forum

All Questions      Topic List

Vector Questions

Previous in All Question      Next in All Question      

Previous in Vector      Next in Vector      

Question Number 41606 by psyche-ace last updated on 10/Aug/18

4x^4 +16x^3 +24x^2 −9x−1=0 using any method. find real value of x that satisfy the polynomial

$$\mathrm{4}\boldsymbol{\mathrm{x}}^{\mathrm{4}} +\mathrm{16}\boldsymbol{\mathrm{x}}^{\mathrm{3}} +\mathrm{24}\boldsymbol{\mathrm{x}}^{\mathrm{2}} −\mathrm{9}\boldsymbol{\mathrm{x}}−\mathrm{1}=\mathrm{0} \\ $$$$\boldsymbol{\mathrm{using}}\:\boldsymbol{\mathrm{any}}\:\boldsymbol{\mathrm{method}}.\:\boldsymbol{\mathrm{find}}\:\:\boldsymbol{\mathrm{real}}\:\boldsymbol{\mathrm{value}}\:\boldsymbol{\mathrm{of}}\:\:\boldsymbol{\mathrm{x}}\:\:\boldsymbol{\mathrm{that}}\:\boldsymbol{\mathrm{satisfy}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{polynomial}} \\ $$

Answered by MJS last updated on 10/Aug/18

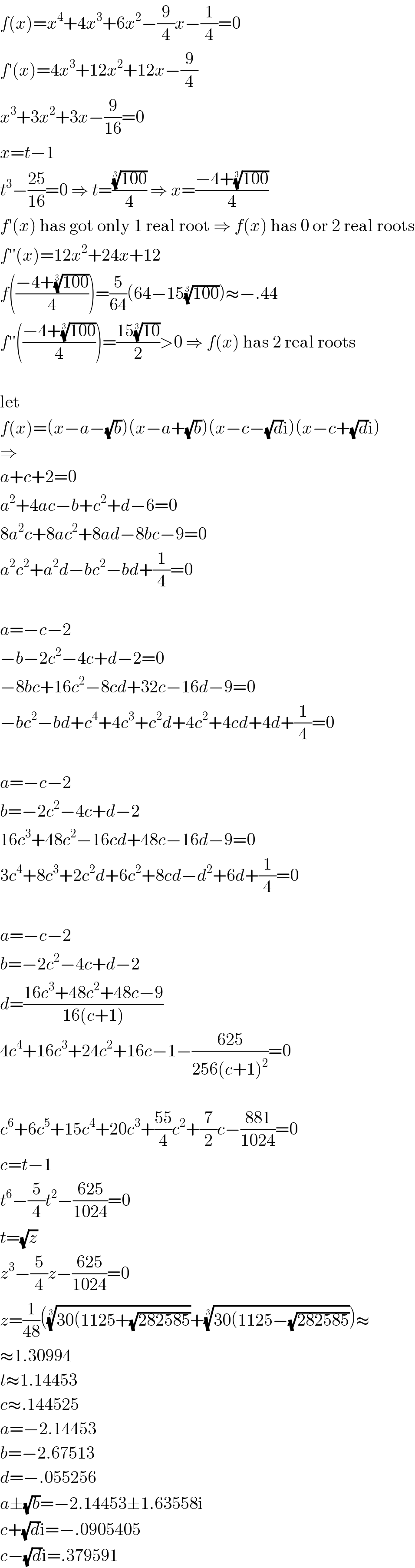
f(x)=x^4 +4x^3 +6x^2 −(9/4)x−(1/4)=0 f′(x)=4x^3 +12x^2 +12x−(9/4) x^3 +3x^2 +3x−(9/(16))=0 x=t−1 t^3 −((25)/(16))=0 ⇒ t=(((100))^(1/3) /4) ⇒ x=((−4+((100))^(1/3) )/4) f′(x) has got only 1 real root ⇒ f(x) has 0 or 2 real roots f′′(x)=12x^2 +24x+12 f(((−4+((100))^(1/3) )/4))=(5/(64))(64−15((100))^(1/3) )≈−.44 f′′(((−4+((100))^(1/3) )/4))=((15((10))^(1/3) )/2)>0 ⇒ f(x) has 2 real roots let f(x)=(x−a−(√b))(x−a+(√b))(x−c−(√d)i)(x−c+(√d)i) ⇒ a+c+2=0 a^2 +4ac−b+c^2 +d−6=0 8a^2 c+8ac^2 +8ad−8bc−9=0 a^2 c^2 +a^2 d−bc^2 −bd+(1/4)=0 a=−c−2 −b−2c^2 −4c+d−2=0 −8bc+16c^2 −8cd+32c−16d−9=0 −bc^2 −bd+c^4 +4c^3 +c^2 d+4c^2 +4cd+4d+(1/4)=0 a=−c−2 b=−2c^2 −4c+d−2 16c^3 +48c^2 −16cd+48c−16d−9=0 3c^4 +8c^3 +2c^2 d+6c^2 +8cd−d^2 +6d+(1/4)=0 a=−c−2 b=−2c^2 −4c+d−2 d=((16c^3 +48c^2 +48c−9)/(16(c+1))) 4c^4 +16c^3 +24c^2 +16c−1−((625)/(256(c+1)^2 ))=0 c^6 +6c^5 +15c^4 +20c^3 +((55)/4)c^2 +(7/2)c−((881)/(1024))=0 c=t−1 t^6 −(5/4)t^2 −((625)/(1024))=0 t=(√z) z^3 −(5/4)z−((625)/(1024))=0 z=(1/(48))(((30(1125+(√(282585))))^(1/3) +((30(1125−(√(282585))))^(1/3) )≈ ≈1.30994 t≈1.14453 c≈.144525 a=−2.14453 b=−2.67513 d=−.055256 a±(√b)=−2.14453±1.63558i c+(√d)i=−.0905405 c−(√d)i=.379591

$${f}\left({x}\right)={x}^{\mathrm{4}} +\mathrm{4}{x}^{\mathrm{3}} +\mathrm{6}{x}^{\mathrm{2}} −\frac{\mathrm{9}}{\mathrm{4}}{x}−\frac{\mathrm{1}}{\mathrm{4}}=\mathrm{0} \\ $$$${f}'\left({x}\right)=\mathrm{4}{x}^{\mathrm{3}} +\mathrm{12}{x}^{\mathrm{2}} +\mathrm{12}{x}−\frac{\mathrm{9}}{\mathrm{4}} \\ $$$${x}^{\mathrm{3}} +\mathrm{3}{x}^{\mathrm{2}} +\mathrm{3}{x}−\frac{\mathrm{9}}{\mathrm{16}}=\mathrm{0} \\ $$$${x}={t}−\mathrm{1} \\ $$$${t}^{\mathrm{3}} −\frac{\mathrm{25}}{\mathrm{16}}=\mathrm{0}\:\Rightarrow\:{t}=\frac{\sqrt[{\mathrm{3}}]{\mathrm{100}}}{\mathrm{4}}\:\Rightarrow\:{x}=\frac{−\mathrm{4}+\sqrt[{\mathrm{3}}]{\mathrm{100}}}{\mathrm{4}} \\ $$$${f}'\left({x}\right)\:\mathrm{has}\:\mathrm{got}\:\mathrm{only}\:\mathrm{1}\:\mathrm{real}\:\mathrm{root}\:\Rightarrow\:{f}\left({x}\right)\:\mathrm{has}\:\mathrm{0}\:\mathrm{or}\:\mathrm{2}\:\mathrm{real}\:\mathrm{roots} \\ $$$${f}''\left({x}\right)=\mathrm{12}{x}^{\mathrm{2}} +\mathrm{24}{x}+\mathrm{12} \\ $$$${f}\left(\frac{−\mathrm{4}+\sqrt[{\mathrm{3}}]{\mathrm{100}}}{\mathrm{4}}\right)=\frac{\mathrm{5}}{\mathrm{64}}\left(\mathrm{64}−\mathrm{15}\sqrt[{\mathrm{3}}]{\mathrm{100}}\right)\approx−.\mathrm{44} \\ $$$${f}''\left(\frac{−\mathrm{4}+\sqrt[{\mathrm{3}}]{\mathrm{100}}}{\mathrm{4}}\right)=\frac{\mathrm{15}\sqrt[{\mathrm{3}}]{\mathrm{10}}}{\mathrm{2}}>\mathrm{0}\:\Rightarrow\:{f}\left({x}\right)\:\mathrm{has}\:\mathrm{2}\:\mathrm{real}\:\mathrm{roots} \\ $$$$ \\ $$$$\mathrm{let} \\ $$$${f}\left({x}\right)=\left({x}−{a}−\sqrt{{b}}\right)\left({x}−{a}+\sqrt{{b}}\right)\left({x}−{c}−\sqrt{{d}}\mathrm{i}\right)\left({x}−{c}+\sqrt{{d}}\mathrm{i}\right) \\ $$$$\Rightarrow \\ $$$${a}+{c}+\mathrm{2}=\mathrm{0} \\ $$$${a}^{\mathrm{2}} +\mathrm{4}{ac}−{b}+{c}^{\mathrm{2}} +{d}−\mathrm{6}=\mathrm{0} \\ $$$$\mathrm{8}{a}^{\mathrm{2}} {c}+\mathrm{8}{ac}^{\mathrm{2}} +\mathrm{8}{ad}−\mathrm{8}{bc}−\mathrm{9}=\mathrm{0} \\ $$$${a}^{\mathrm{2}} {c}^{\mathrm{2}} +{a}^{\mathrm{2}} {d}−{bc}^{\mathrm{2}} −{bd}+\frac{\mathrm{1}}{\mathrm{4}}=\mathrm{0} \\ $$$$ \\ $$$${a}=−{c}−\mathrm{2} \\ $$$$−{b}−\mathrm{2}{c}^{\mathrm{2}} −\mathrm{4}{c}+{d}−\mathrm{2}=\mathrm{0} \\ $$$$−\mathrm{8}{bc}+\mathrm{16}{c}^{\mathrm{2}} −\mathrm{8}{cd}+\mathrm{32}{c}−\mathrm{16}{d}−\mathrm{9}=\mathrm{0} \\ $$$$−{bc}^{\mathrm{2}} −{bd}+{c}^{\mathrm{4}} +\mathrm{4}{c}^{\mathrm{3}} +{c}^{\mathrm{2}} {d}+\mathrm{4}{c}^{\mathrm{2}} +\mathrm{4}{cd}+\mathrm{4}{d}+\frac{\mathrm{1}}{\mathrm{4}}=\mathrm{0} \\ $$$$ \\ $$$${a}=−{c}−\mathrm{2} \\ $$$${b}=−\mathrm{2}{c}^{\mathrm{2}} −\mathrm{4}{c}+{d}−\mathrm{2} \\ $$$$\mathrm{16}{c}^{\mathrm{3}} +\mathrm{48}{c}^{\mathrm{2}} −\mathrm{16}{cd}+\mathrm{48}{c}−\mathrm{16}{d}−\mathrm{9}=\mathrm{0} \\ $$$$\mathrm{3}{c}^{\mathrm{4}} +\mathrm{8}{c}^{\mathrm{3}} +\mathrm{2}{c}^{\mathrm{2}} {d}+\mathrm{6}{c}^{\mathrm{2}} +\mathrm{8}{cd}−{d}^{\mathrm{2}} +\mathrm{6}{d}+\frac{\mathrm{1}}{\mathrm{4}}=\mathrm{0} \\ $$$$ \\ $$$${a}=−{c}−\mathrm{2} \\ $$$${b}=−\mathrm{2}{c}^{\mathrm{2}} −\mathrm{4}{c}+{d}−\mathrm{2} \\ $$$${d}=\frac{\mathrm{16}{c}^{\mathrm{3}} +\mathrm{48}{c}^{\mathrm{2}} +\mathrm{48}{c}−\mathrm{9}}{\mathrm{16}\left({c}+\mathrm{1}\right)} \\ $$$$\mathrm{4}{c}^{\mathrm{4}} +\mathrm{16}{c}^{\mathrm{3}} +\mathrm{24}{c}^{\mathrm{2}} +\mathrm{16}{c}−\mathrm{1}−\frac{\mathrm{625}}{\mathrm{256}\left({c}+\mathrm{1}\right)^{\mathrm{2}} }=\mathrm{0} \\ $$$$ \\ $$$${c}^{\mathrm{6}} +\mathrm{6}{c}^{\mathrm{5}} +\mathrm{15}{c}^{\mathrm{4}} +\mathrm{20}{c}^{\mathrm{3}} +\frac{\mathrm{55}}{\mathrm{4}}{c}^{\mathrm{2}} +\frac{\mathrm{7}}{\mathrm{2}}{c}−\frac{\mathrm{881}}{\mathrm{1024}}=\mathrm{0} \\ $$$${c}={t}−\mathrm{1} \\ $$$${t}^{\mathrm{6}} −\frac{\mathrm{5}}{\mathrm{4}}{t}^{\mathrm{2}} −\frac{\mathrm{625}}{\mathrm{1024}}=\mathrm{0} \\ $$$${t}=\sqrt{{z}} \\ $$$${z}^{\mathrm{3}} −\frac{\mathrm{5}}{\mathrm{4}}{z}−\frac{\mathrm{625}}{\mathrm{1024}}=\mathrm{0} \\ $$$${z}=\frac{\mathrm{1}}{\mathrm{48}}\left(\sqrt[{\mathrm{3}}]{\mathrm{30}\left(\mathrm{1125}+\sqrt{\mathrm{282585}}\right.}+\sqrt[{\mathrm{3}}]{\mathrm{30}\left(\mathrm{1125}−\sqrt{\mathrm{282585}}\right.}\right)\approx \\ $$$$\approx\mathrm{1}.\mathrm{30994} \\ $$$${t}\approx\mathrm{1}.\mathrm{14453} \\ $$$${c}\approx.\mathrm{144525} \\ $$$${a}=−\mathrm{2}.\mathrm{14453} \\ $$$${b}=−\mathrm{2}.\mathrm{67513} \\ $$$${d}=−.\mathrm{055256} \\ $$$${a}\pm\sqrt{{b}}=−\mathrm{2}.\mathrm{14453}\pm\mathrm{1}.\mathrm{63558i} \\ $$$${c}+\sqrt{{d}}\mathrm{i}=−.\mathrm{0905405} \\ $$$${c}−\sqrt{{d}}\mathrm{i}=.\mathrm{379591} \\ $$

Commented by MJS last updated on 10/Aug/18

to eliminate the bx^(n−1) you can always set x=t−(b/n) if the constant factor if ax^n is equal to 1 (a=1) examples: 3x^2 +4x−5=0 x^2 +(4/3)x−(5/3)=0 x=t−(2/3) (t−(2/3))^2 +(4/3)(t−(2/3))−(5/3)=0 t^2 −((19)/9)=0 (which in this case is the same as the usual formula x=−(p/2)±(√((p^2 /4)−q)) 4x^3 −5x^2 +3x−2=0 x^3 −(5/4)x^2 +(3/4)x−(1/2)=0 x=t+(5/(12)) (t+(5/(12)))^3 −(5/4)(t+(5/(12)))^2 +(3/4)(t+(5/(12)))−(1/2)=0 t^3 +((11)/(48))t−((287)/(864))=0 (which in this case leads to Cardano′s formula or in case of 3 real solutions to the trigonometric method) in case of a 4^(th) −degree polynome with 2 real and 2 complex roots my method leads to a polynome of 6^(th) degree which can be reduced to one of 3^(rd) degree... will post an example later

$$\mathrm{to}\:\mathrm{eliminate}\:\mathrm{the}\:{bx}^{{n}−\mathrm{1}} \:\mathrm{you}\:\mathrm{can}\:\mathrm{always}\:\mathrm{set} \\ $$$${x}={t}−\frac{{b}}{{n}}\:\mathrm{if}\:\mathrm{the}\:\mathrm{constant}\:\mathrm{factor}\:\mathrm{if}\:{ax}^{{n}} \:\mathrm{is}\:\mathrm{equal} \\ $$$$\mathrm{to}\:\mathrm{1}\:\left({a}=\mathrm{1}\right) \\ $$$$\mathrm{examples}: \\ $$$$\mathrm{3}{x}^{\mathrm{2}} +\mathrm{4}{x}−\mathrm{5}=\mathrm{0} \\ $$$${x}^{\mathrm{2}} +\frac{\mathrm{4}}{\mathrm{3}}{x}−\frac{\mathrm{5}}{\mathrm{3}}=\mathrm{0} \\ $$$${x}={t}−\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$\left({t}−\frac{\mathrm{2}}{\mathrm{3}}\right)^{\mathrm{2}} +\frac{\mathrm{4}}{\mathrm{3}}\left({t}−\frac{\mathrm{2}}{\mathrm{3}}\right)−\frac{\mathrm{5}}{\mathrm{3}}=\mathrm{0} \\ $$$${t}^{\mathrm{2}} −\frac{\mathrm{19}}{\mathrm{9}}=\mathrm{0} \\ $$$$\left(\mathrm{which}\:\mathrm{in}\:\mathrm{this}\:\mathrm{case}\:\mathrm{is}\:\mathrm{the}\:\mathrm{same}\:\mathrm{as}\:\mathrm{the}\:\mathrm{usual}\right. \\ $$$$\mathrm{formula}\:{x}=−\frac{{p}}{\mathrm{2}}\pm\sqrt{\frac{{p}^{\mathrm{2}} }{\mathrm{4}}−{q}} \\ $$$$ \\ $$$$\mathrm{4}{x}^{\mathrm{3}} −\mathrm{5}{x}^{\mathrm{2}} +\mathrm{3}{x}−\mathrm{2}=\mathrm{0} \\ $$$${x}^{\mathrm{3}} −\frac{\mathrm{5}}{\mathrm{4}}{x}^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}}{x}−\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{0} \\ $$$${x}={t}+\frac{\mathrm{5}}{\mathrm{12}} \\ $$$$\left({t}+\frac{\mathrm{5}}{\mathrm{12}}\right)^{\mathrm{3}} −\frac{\mathrm{5}}{\mathrm{4}}\left({t}+\frac{\mathrm{5}}{\mathrm{12}}\right)^{\mathrm{2}} +\frac{\mathrm{3}}{\mathrm{4}}\left({t}+\frac{\mathrm{5}}{\mathrm{12}}\right)−\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{0} \\ $$$${t}^{\mathrm{3}} +\frac{\mathrm{11}}{\mathrm{48}}{t}−\frac{\mathrm{287}}{\mathrm{864}}=\mathrm{0} \\ $$$$\left(\mathrm{which}\:\mathrm{in}\:\mathrm{this}\:\mathrm{case}\:\mathrm{leads}\:\mathrm{to}\:\mathrm{Cardano}'\mathrm{s}\:\mathrm{formula}\right. \\ $$$$\mathrm{or}\:\mathrm{in}\:\mathrm{case}\:\mathrm{of}\:\mathrm{3}\:\mathrm{real}\:\mathrm{solutions}\:\mathrm{to}\:\mathrm{the}\:\mathrm{trigonometric} \\ $$$$\left.\mathrm{method}\right) \\ $$$$ \\ $$$$\mathrm{in}\:\mathrm{case}\:\mathrm{of}\:\mathrm{a}\:\mathrm{4}^{\mathrm{th}} −\mathrm{degree}\:\mathrm{polynome}\:\mathrm{with}\:\mathrm{2}\:\mathrm{real} \\ $$$$\mathrm{and}\:\mathrm{2}\:\mathrm{complex}\:\mathrm{roots}\:\mathrm{my}\:\mathrm{method}\:\mathrm{leads}\:\mathrm{to}\:\mathrm{a} \\ $$$$\mathrm{polynome}\:\mathrm{of}\:\mathrm{6}^{\mathrm{th}} \:\mathrm{degree}\:\mathrm{which}\:\mathrm{can}\:\mathrm{be}\:\mathrm{reduced} \\ $$$$\mathrm{to}\:\mathrm{one}\:\mathrm{of}\:\mathrm{3}^{\mathrm{rd}} \:\mathrm{degree}... \\ $$$$\mathrm{will}\:\mathrm{post}\:\mathrm{an}\:\mathrm{example}\:\mathrm{later} \\ $$

Commented by Tawa1 last updated on 10/Aug/18

Sir how can we know that x = t − 1

$$\mathrm{Sir}\:\mathrm{how}\:\mathrm{can}\:\mathrm{we}\:\mathrm{know}\:\mathrm{that}\:\:\mathrm{x}\:=\:\mathrm{t}\:−\:\mathrm{1} \\ $$

Commented by Tawa1 last updated on 10/Aug/18

wow, please send it sir. God bless you sir

$$\mathrm{wow},\:\mathrm{please}\:\mathrm{send}\:\mathrm{it}\:\mathrm{sir}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$

Commented by Tawa1 last updated on 10/Aug/18

Am still expecting the other method and examples sir. God bless you

$$\mathrm{Am}\:\mathrm{still}\:\mathrm{expecting}\:\mathrm{the}\:\mathrm{other}\:\mathrm{method}\:\mathrm{and}\:\mathrm{examples}\:\mathrm{sir}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you} \\ $$

Commented by Tawa1 last updated on 10/Aug/18

i will love to see the formulars sir

$$\mathrm{i}\:\mathrm{will}\:\mathrm{love}\:\mathrm{to}\:\mathrm{see}\:\mathrm{the}\:\mathrm{formulars}\:\mathrm{sir} \\ $$

Commented by Tawa1 last updated on 11/Aug/18

Sir MJS am still expecting sir

$$\mathrm{Sir}\:\mathrm{MJS}\:\mathrm{am}\:\mathrm{still}\:\mathrm{expecting}\:\mathrm{sir} \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 10/Aug/18

4x^2 (x^2 +4x+4)+8x^2 −9x−1=0 {(2x)(x+2)}^2 +9x^2 −6x+1−x^2 −3x−2=0 {(2x)(x+2)}^2 +(3x−1)^2 −(x^2 +3x+2)=0 {(2x)(x+2)}^2 +(3x−1)^2 −{x^2 +2.x.(3/2)+(9/4)+2−(9/4)}=0 {(2x)(x+2)}^2 +(3x−1)^2 −{(x+(3/2))^2 −(1/4)}=0 [{(2x)(x+2)}^2 +(3x−1)^2 +(1/4)]−[(x+(3/2))^2 ]=0 now i want to say some thing the value of [{(2x)(x+2)}^2 +(3x−1)^2 +(1/4)] is +ve and say its value is p now the value of [(x+(3/2))^2 ] is also positve and say value is q now the eqn is p−q=0 that imply the value of p should be equal to q to make the equation true but {(2x)(x+2)}^2 >[(x+(3/2))^2 ] so to me p can not be equal to q to make the equation true.. to me p>>q so i think this equation is not feasible...

$$\mathrm{4}{x}^{\mathrm{2}} \left({x}^{\mathrm{2}} +\mathrm{4}{x}+\mathrm{4}\right)+\mathrm{8}{x}^{\mathrm{2}} −\mathrm{9}{x}−\mathrm{1}=\mathrm{0} \\ $$$$\left\{\left(\mathrm{2}{x}\right)\left({x}+\mathrm{2}\right)\right\}^{\mathrm{2}} +\mathrm{9}{x}^{\mathrm{2}} −\mathrm{6}{x}+\mathrm{1}−{x}^{\mathrm{2}} −\mathrm{3}{x}−\mathrm{2}=\mathrm{0} \\ $$$$\left\{\left(\mathrm{2}{x}\right)\left({x}+\mathrm{2}\right)\right\}^{\mathrm{2}} +\left(\mathrm{3}{x}−\mathrm{1}\right)^{\mathrm{2}} −\left({x}^{\mathrm{2}} +\mathrm{3}{x}+\mathrm{2}\right)=\mathrm{0} \\ $$$$\left\{\left(\mathrm{2}{x}\right)\left({x}+\mathrm{2}\right)\right\}^{\mathrm{2}} +\left(\mathrm{3}{x}−\mathrm{1}\right)^{\mathrm{2}} −\left\{{x}^{\mathrm{2}} +\mathrm{2}.{x}.\frac{\mathrm{3}}{\mathrm{2}}+\frac{\mathrm{9}}{\mathrm{4}}+\mathrm{2}−\frac{\mathrm{9}}{\mathrm{4}}\right\}=\mathrm{0} \\ $$$$\left\{\left(\mathrm{2}{x}\right)\left({x}+\mathrm{2}\right)\right\}^{\mathrm{2}} +\left(\mathrm{3}{x}−\mathrm{1}\right)^{\mathrm{2}} −\left\{\left({x}+\frac{\mathrm{3}}{\mathrm{2}}\right)^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{4}}\right\}=\mathrm{0} \\ $$$$\left[\left\{\left(\mathrm{2}{x}\right)\left({x}+\mathrm{2}\right)\right\}^{\mathrm{2}} +\left(\mathrm{3}{x}−\mathrm{1}\right)^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{4}}\right]−\left[\left({x}+\frac{\mathrm{3}}{\mathrm{2}}\right)^{\mathrm{2}} \right]=\mathrm{0} \\ $$$${now}\:{i}\:{want}\:{to}\:{say}\:{some}\:{thing} \\ $$$${the}\:{value}\:{of}\: \\ $$$$\left[\left\{\left(\mathrm{2}{x}\right)\left({x}+\mathrm{2}\right)\right\}^{\mathrm{2}} +\left(\mathrm{3}{x}−\mathrm{1}\right)^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{4}}\right]\:{is}\:+{ve}\:{and}\:{say}\:{its} \\ $$$${value}\:{is}\:{p} \\ $$$${now}\:{the}\:{value}\:{of}\:\left[\left({x}+\frac{\mathrm{3}}{\mathrm{2}}\right)^{\mathrm{2}} \right]\:{is}\:{also}\:{positve}\:{and} \\ $$$${say}\:{value}\:{is}\:{q} \\ $$$${now}\:{the}\:{eqn}\:{is}\:{p}−{q}=\mathrm{0} \\ $$$${that}\:{imply}\:{the}\:{value}\:{of}\:{p}\:{should}\:{be}\:{equal}\:{to}\:{q}\:{to} \\ $$$${make}\:{the}\:{equation}\:{true} \\ $$$${but}\:\left\{\left(\mathrm{2}{x}\right)\left({x}+\mathrm{2}\right)\right\}^{\mathrm{2}} >\left[\left({x}+\frac{\mathrm{3}}{\mathrm{2}}\right)^{\mathrm{2}} \right] \\ $$$${so}\:{to}\:{me}\:{p}\:{can}\:{not}\:{be}\:{equal}\:{to}\:{q}\:{to}\:{make} \\ $$$${the}\:{equation}\:{true}.. \\ $$$${to}\:{me}\:{p}>>{q} \\ $$$${so}\:{i}\:{think}\:{this}\:{equation}\:{is}\:{not}\:{feasible}... \\ $$$$ \\ $$$$ \\ $$

Commented by Tawa1 last updated on 10/Aug/18

God bless you sir

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com