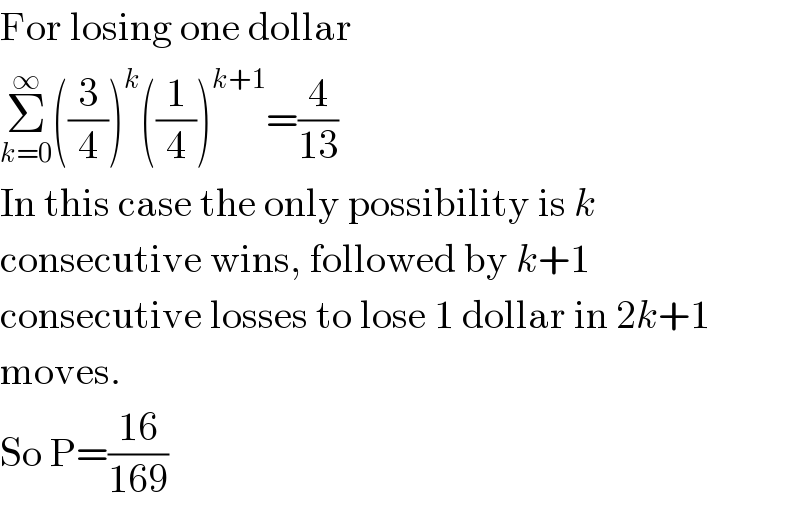Question and Answers Forum
Previous in Probability and Statistics Next in Probability and Statistics
Question Number 4166 by Filup last updated on 30/Dec/15

Commented by prakash jain last updated on 31/Dec/15
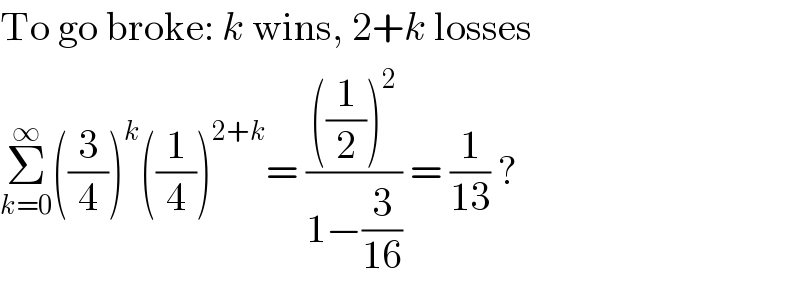
Commented by Filup last updated on 31/Dec/15
Commented by Filup last updated on 31/Dec/15

Commented by prakash jain last updated on 31/Dec/15

Commented by Yozzii last updated on 31/Dec/15

Commented by Yozzii last updated on 31/Dec/15

Commented by Yozzii last updated on 31/Dec/15

Commented by prakash jain last updated on 31/Dec/15
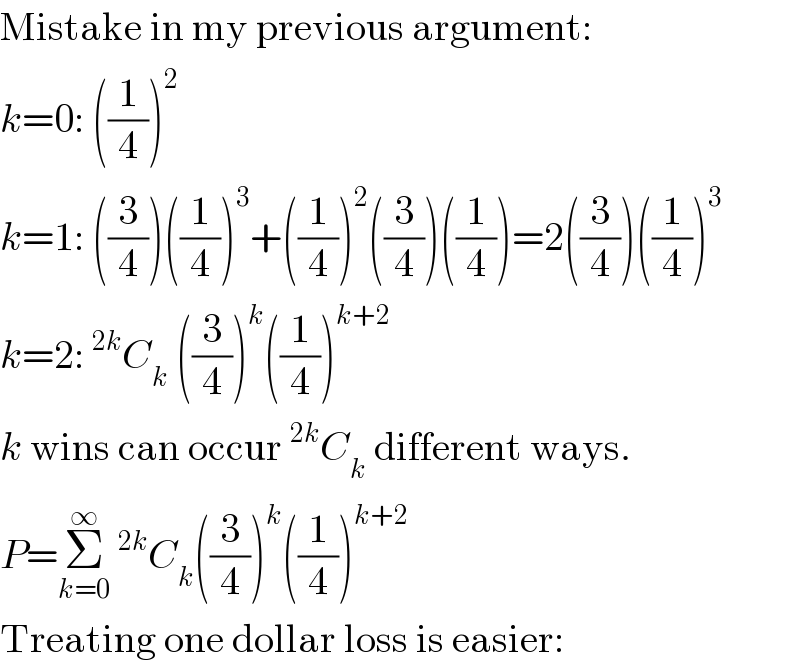
Commented by prakash jain last updated on 31/Dec/15