Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 41847 by maxmathsup by imad last updated on 13/Aug/18

let f(x) = ∫_0 ^(π/4) (dt/(x +tan(t))) 1) find anoher expression off (x) 2) calculate ∫_0 ^(π/4) (dt/(2+tan(t))) and A(θ) = ∫_0 ^(π/4) (dt/(sinθ+tant)) 3) calculate ∫_0 ^(π/4) (dt/((1+tant)^2 ))

$${let}\:{f}\left({x}\right)\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\:\frac{{dt}}{{x}\:+{tan}\left({t}\right)} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{anoher}\:{expression}\:{off}\:\left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\frac{{dt}}{\mathrm{2}+{tan}\left({t}\right)}\:\:\:{and}\:\:{A}\left(\theta\right)\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\frac{{dt}}{{sin}\theta+{tant}} \\ $$$$\left.\mathrm{3}\right)\:{calculate}\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\frac{{dt}}{\left(\mathrm{1}+{tant}\right)^{\mathrm{2}} } \\ $$

Answered by maxmathsup by imad last updated on 14/Aug/18

1) we have f(x) = ∫_0 ^(π/4) (dt/(x+tant)) changement tant =u give f(x) = ∫_0 ^1 (du/((1+u^2 )(x+u))) let decompose F(u) = (1/((x+u)(1+u^2 ))) F(u) =(a/(x+u)) +((bu +c)/(u^2 +1)) a =lim_(u→−x) (x+u)F(u) = (1/(1+x^2 )) lim_(u→+∞) uF(u) =0 =a +b ⇒b=−(1/(1+x^2 )) ⇒F(u)=(1/((1+x^2 )(u+x))) +((−(1/(1+x^2 ))u +c)/(u^2 +1)) F(0) =(1/x) = (1/(x(1+x^2 ))) +c ⇒1 =(1/(1+x^2 )) +xc ⇒xc =1−(1/(1+x^2 )) ⇒ xc =(x^2 /(1+x^2 )) ⇒ c =(x/(1+x^2 )) ( we suppose x≠0) ⇒ F(u) = (1/((1+x^2 )(u+x))) −(1/(1+x^2 )) ((u−x)/(u^2 +1)) ⇒ f(x) = (1/(1+x^2 )) { ∫_0 ^1 (du/(u+x)) −(1/2)∫_0 ^1 ((2u)/(u^2 +1))du +x ∫_0 ^1 (du/(1+u^2 ))} =(1/(1+x^2 )){ [ln∣u+x∣_0 ^1 −(1/2)[ln∣u^2 +1∣]_0 ^1 +x [arctanu ]_0 ^1 } f(x)=(1/(1+x^2 )){ ln∣1+x∣−ln∣x∣ −(1/2)ln(2) +((πx)/4)}

$$\left.\mathrm{1}\right)\:{we}\:{have}\:\:{f}\left({x}\right)\:=\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\:\frac{{dt}}{{x}+{tant}}\:\:{changement}\:{tant}\:={u}\:{give} \\ $$$${f}\left({x}\right)\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\:\:\frac{{du}}{\left(\mathrm{1}+{u}^{\mathrm{2}} \right)\left({x}+{u}\right)}\:\:{let}\:{decompose}\:{F}\left({u}\right)\:=\:\frac{\mathrm{1}}{\left({x}+{u}\right)\left(\mathrm{1}+{u}^{\mathrm{2}} \right)} \\ $$$${F}\left({u}\right)\:=\frac{{a}}{{x}+{u}}\:+\frac{{bu}\:+{c}}{{u}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${a}\:={lim}_{{u}\rightarrow−{x}} \left({x}+{u}\right){F}\left({u}\right)\:=\:\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$${lim}_{{u}\rightarrow+\infty} {uF}\left({u}\right)\:=\mathrm{0}\:={a}\:+{b}\:\Rightarrow{b}=−\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\Rightarrow{F}\left({u}\right)=\frac{\mathrm{1}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\left({u}+{x}\right)}\:+\frac{−\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }{u}\:+{c}}{{u}^{\mathrm{2}} \:+\mathrm{1}} \\ $$$${F}\left(\mathrm{0}\right)\:=\frac{\mathrm{1}}{{x}}\:=\:\frac{\mathrm{1}}{{x}\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}\:+{c}\:\Rightarrow\mathrm{1}\:=\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\:+{xc}\:\Rightarrow{xc}\:=\mathrm{1}−\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\Rightarrow \\ $$$${xc}\:=\frac{{x}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} }\:\Rightarrow\:{c}\:=\frac{{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\:\left(\:{we}\:{suppose}\:{x}\neq\mathrm{0}\right)\:\Rightarrow \\ $$$${F}\left({u}\right)\:=\:\:\frac{\mathrm{1}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\left({u}+{x}\right)}\:−\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\:\:\frac{{u}−{x}}{{u}^{\mathrm{2}} \:+\mathrm{1}}\:\Rightarrow \\ $$$${f}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\:\left\{\:\:\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{du}}{{u}+{x}}\:−\frac{\mathrm{1}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{\mathrm{2}{u}}{{u}^{\mathrm{2}} \:+\mathrm{1}}{du}\:\:+{x}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\frac{{du}}{\mathrm{1}+{u}^{\mathrm{2}} }\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\left\{\:\:\left[{ln}\mid{u}+{x}\mid_{\mathrm{0}} ^{\mathrm{1}} \:−\frac{\mathrm{1}}{\mathrm{2}}\left[{ln}\mid{u}^{\mathrm{2}} \:+\mathrm{1}\mid\right]_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:+{x}\:\left[{arctanu}\:\right]_{\mathrm{0}} ^{\mathrm{1}} \right\}\right. \\ $$$${f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }\left\{\:{ln}\mid\mathrm{1}+{x}\mid−{ln}\mid{x}\mid\:−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\mathrm{2}\right)\:+\frac{\pi{x}}{\mathrm{4}}\right\} \\ $$

Commented by maxmathsup by imad last updated on 14/Aug/18

2) ∫_0 ^(π/4) (dt/(2 +tan(t))) =f(2) = (1/5){ ln(3)−ln(2)−(1/2)ln(2) +(π/2)} =(1/5){ ln(3)−(3/2)ln(2) +(π/2)} also we have ∫_0 ^(π/4) (dt/(sinθ +tant)) =f(sinθ) =(1/(1+sin^2 θ)){ ln(1+sin^2 θ)−ln∣sinθ∣−(1/2)ln(2)+((πsinπ)/4)}

$$\left.\mathrm{2}\right)\:\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\frac{{dt}}{\mathrm{2}\:+{tan}\left({t}\right)}\:={f}\left(\mathrm{2}\right)\:=\:\frac{\mathrm{1}}{\mathrm{5}}\left\{\:{ln}\left(\mathrm{3}\right)−{ln}\left(\mathrm{2}\right)−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\mathrm{2}\right)\:+\frac{\pi}{\mathrm{2}}\right\} \\ $$$$=\frac{\mathrm{1}}{\mathrm{5}}\left\{\:{ln}\left(\mathrm{3}\right)−\frac{\mathrm{3}}{\mathrm{2}}{ln}\left(\mathrm{2}\right)\:+\frac{\pi}{\mathrm{2}}\right\}\:\:{also}\:{we}\:{have} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\frac{{dt}}{{sin}\theta\:+{tant}}\:={f}\left({sin}\theta\right)\:=\frac{\mathrm{1}}{\mathrm{1}+{sin}^{\mathrm{2}} \theta}\left\{\:{ln}\left(\mathrm{1}+{sin}^{\mathrm{2}} \theta\right)−{ln}\mid{sin}\theta\mid−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\mathrm{2}\right)+\frac{\pi{sin}\pi}{\mathrm{4}}\right\} \\ $$$$ \\ $$

Commented by maxmathsup by imad last updated on 14/Aug/18

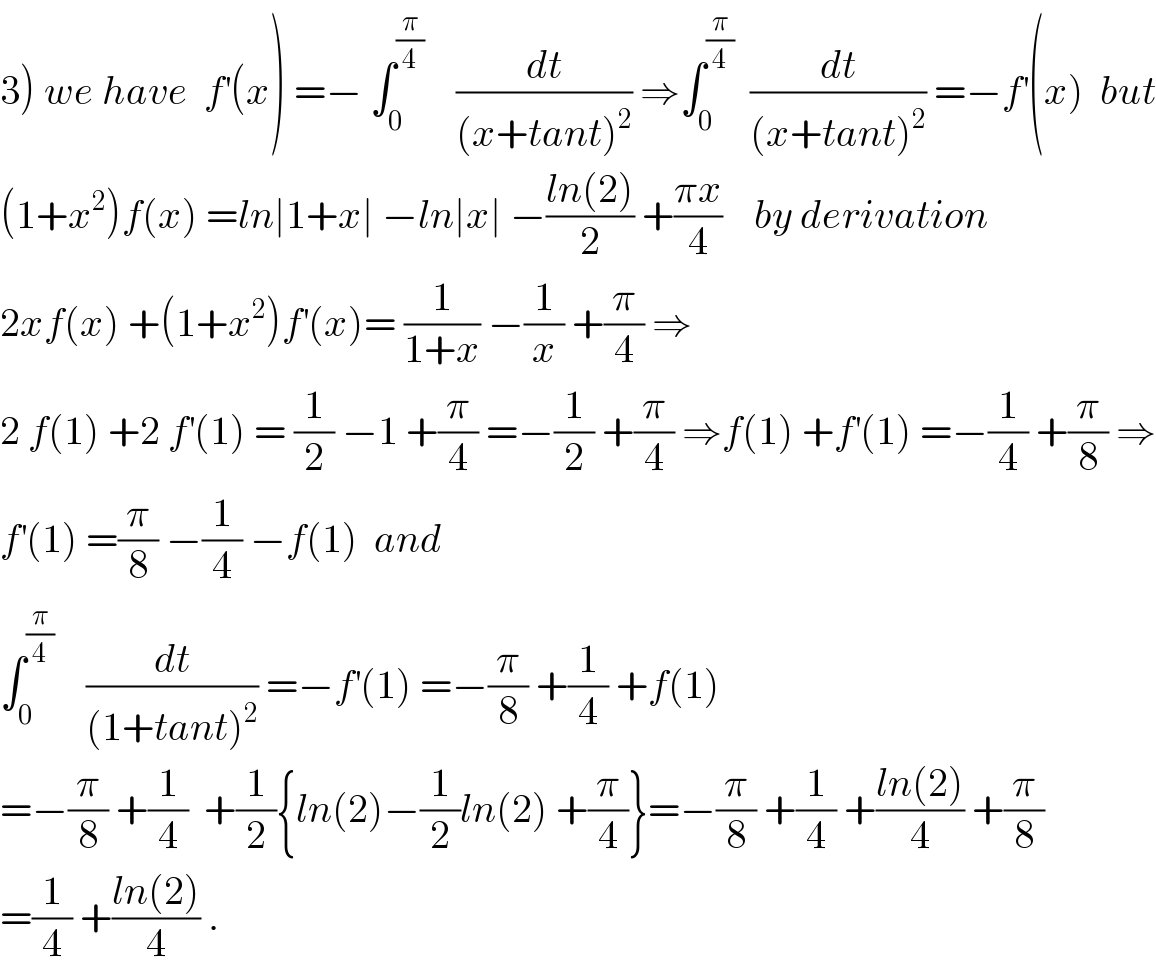
3) we have f^′ (x) =− ∫_0 ^(π/4) (dt/((x+tant)^2 )) ⇒∫_0 ^(π/4) (dt/((x+tant)^2 )) =−f^′ (x) but (1+x^2 )f(x) =ln∣1+x∣ −ln∣x∣ −((ln(2))/2) +((πx)/4) by derivation 2xf(x) +(1+x^2 )f^′ (x)= (1/(1+x)) −(1/x) +(π/4) ⇒ 2 f(1) +2 f^′ (1) = (1/2) −1 +(π/4) =−(1/2) +(π/4) ⇒f(1) +f^′ (1) =−(1/4) +(π/8) ⇒ f^′ (1) =(π/8) −(1/4) −f(1) and ∫_0 ^(π/4) (dt/((1+tant)^2 )) =−f^′ (1) =−(π/8) +(1/4) +f(1) =−(π/8) +(1/4) +(1/2){ln(2)−(1/2)ln(2) +(π/4)}=−(π/8) +(1/4) +((ln(2))/4) +(π/8) =(1/4) +((ln(2))/4) .

$$\left.\mathrm{3}\right)\:{we}\:{have}\:\:{f}^{'} \left({x}\right)\:=−\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\frac{{dt}}{\left({x}+{tant}\right)^{\mathrm{2}} }\:\Rightarrow\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\frac{{dt}}{\left({x}+{tant}\right)^{\mathrm{2}} }\:=−{f}^{'} \left({x}\right)\:\:{but} \\ $$$$\left(\mathrm{1}+{x}^{\mathrm{2}} \right){f}\left({x}\right)\:={ln}\mid\mathrm{1}+{x}\mid\:−{ln}\mid{x}\mid\:−\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{2}}\:+\frac{\pi{x}}{\mathrm{4}}\:\:\:\:{by}\:{derivation} \\ $$$$\mathrm{2}{xf}\left({x}\right)\:+\left(\mathrm{1}+{x}^{\mathrm{2}} \right){f}^{'} \left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{1}+{x}}\:−\frac{\mathrm{1}}{{x}}\:+\frac{\pi}{\mathrm{4}}\:\Rightarrow \\ $$$$\mathrm{2}\:{f}\left(\mathrm{1}\right)\:+\mathrm{2}\:{f}^{'} \left(\mathrm{1}\right)\:=\:\frac{\mathrm{1}}{\mathrm{2}}\:−\mathrm{1}\:+\frac{\pi}{\mathrm{4}}\:=−\frac{\mathrm{1}}{\mathrm{2}}\:+\frac{\pi}{\mathrm{4}}\:\Rightarrow{f}\left(\mathrm{1}\right)\:+{f}^{'} \left(\mathrm{1}\right)\:=−\frac{\mathrm{1}}{\mathrm{4}}\:+\frac{\pi}{\mathrm{8}}\:\Rightarrow \\ $$$${f}^{'} \left(\mathrm{1}\right)\:=\frac{\pi}{\mathrm{8}}\:−\frac{\mathrm{1}}{\mathrm{4}}\:−{f}\left(\mathrm{1}\right)\:\:{and}\: \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\:\:\:\frac{{dt}}{\left(\mathrm{1}+{tant}\right)^{\mathrm{2}} }\:=−{f}^{'} \left(\mathrm{1}\right)\:=−\frac{\pi}{\mathrm{8}}\:+\frac{\mathrm{1}}{\mathrm{4}}\:+{f}\left(\mathrm{1}\right) \\ $$$$=−\frac{\pi}{\mathrm{8}}\:+\frac{\mathrm{1}}{\mathrm{4}}\:\:+\frac{\mathrm{1}}{\mathrm{2}}\left\{{ln}\left(\mathrm{2}\right)−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\mathrm{2}\right)\:+\frac{\pi}{\mathrm{4}}\right\}=−\frac{\pi}{\mathrm{8}}\:+\frac{\mathrm{1}}{\mathrm{4}}\:+\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{4}}\:+\frac{\pi}{\mathrm{8}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{4}}\:+\frac{{ln}\left(\mathrm{2}\right)}{\mathrm{4}}\:. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com