Question and Answers Forum

All Questions      Topic List

Vector Questions

Previous in All Question      Next in All Question      

Previous in Vector      Next in Vector      

Question Number 42180 by rahul 19 last updated on 19/Aug/18

The median AD of triangle ABC is bisected at E and BE meets AC at F. Find AF:FC .

$$\mathrm{The}\:\mathrm{median}\:\mathrm{AD}\:\mathrm{of}\:\mathrm{triangle}\:\mathrm{ABC}\:\mathrm{is}\: \\ $$$$\mathrm{bisected}\:\mathrm{at}\:\mathrm{E}\:\mathrm{and}\:\mathrm{BE}\:\mathrm{meets}\:\mathrm{AC}\:\mathrm{at}\:\mathrm{F}. \\ $$$$\mathrm{Find}\:\mathrm{AF}:\mathrm{FC}\:. \\ $$

Answered by MJS last updated on 19/Aug/18

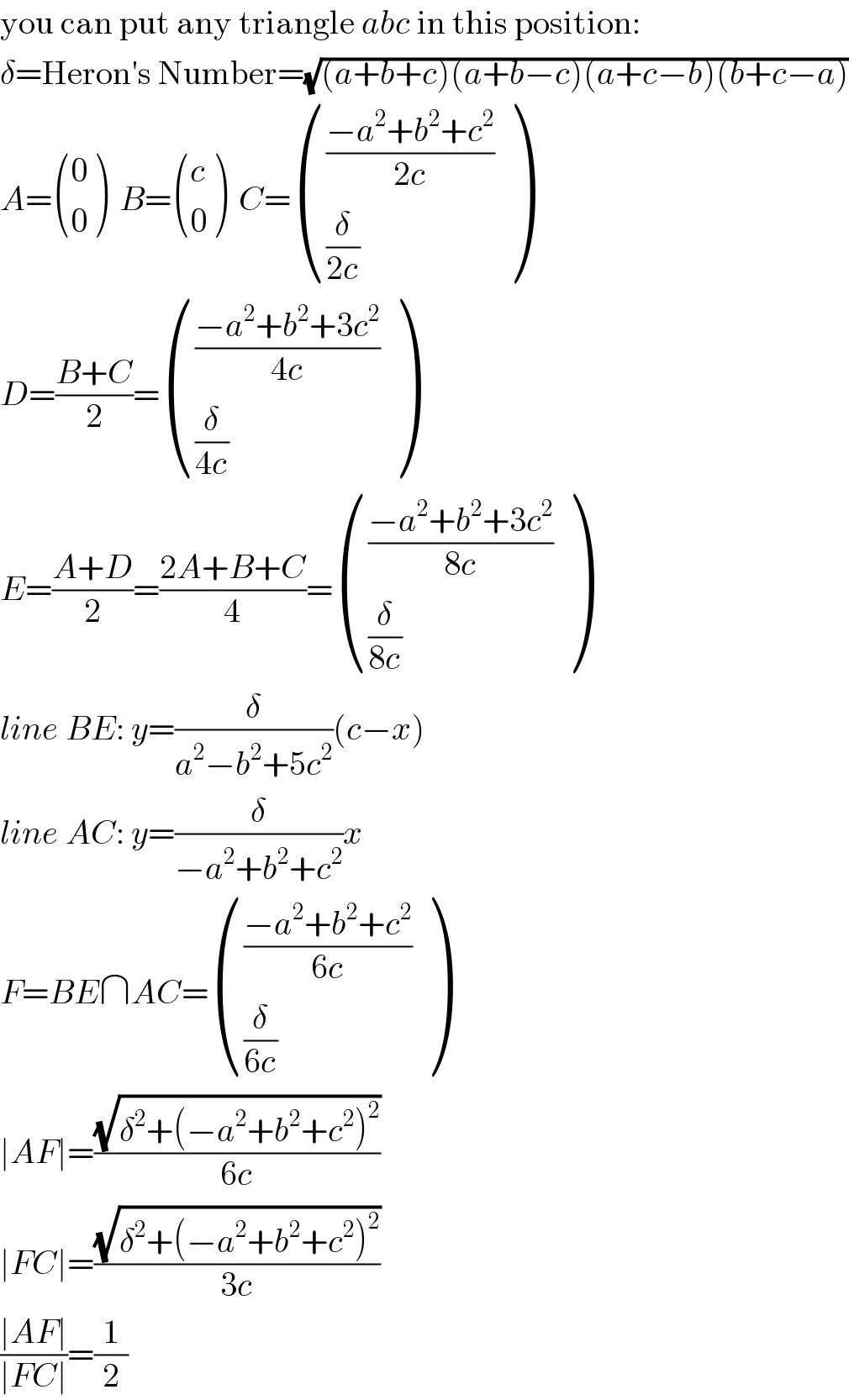
you can put any triangle abc in this position: δ=Heron′s Number=(√((a+b+c)(a+b−c)(a+c−b)(b+c−a))) A= ((0),(0) ) B= ((c),(0) ) C= ((((−a^2 +b^2 +c^2 )/(2c))),((δ/(2c))) ) D=((B+C)/2)= ((((−a^2 +b^2 +3c^2 )/(4c))),((δ/(4c))) ) E=((A+D)/2)=((2A+B+C)/4)= ((((−a^2 +b^2 +3c^2 )/(8c))),((δ/(8c))) ) line BE: y=(δ/(a^2 −b^2 +5c^2 ))(c−x) line AC: y=(δ/(−a^2 +b^2 +c^2 ))x F=BE∩AC= ((((−a^2 +b^2 +c^2 )/(6c))),((δ/(6c))) ) ∣AF∣=((√(δ^2 +(−a^2 +b^2 +c^2 )^2 ))/(6c)) ∣FC∣=((√(δ^2 +(−a^2 +b^2 +c^2 )^2 ))/(3c)) ((∣AF∣)/(∣FC∣))=(1/2)

$$\mathrm{you}\:\mathrm{can}\:\mathrm{put}\:\mathrm{any}\:\mathrm{triangle}\:{abc}\:\mathrm{in}\:\mathrm{this}\:\mathrm{position}: \\ $$$$\delta=\mathrm{Heron}'\mathrm{s}\:\mathrm{Number}=\sqrt{\left({a}+{b}+{c}\right)\left({a}+{b}−{c}\right)\left({a}+{c}−{b}\right)\left({b}+{c}−{a}\right)} \\ $$$${A}=\begin{pmatrix}{\mathrm{0}}\\{\mathrm{0}}\end{pmatrix}\:\:{B}=\begin{pmatrix}{{c}}\\{\mathrm{0}}\end{pmatrix}\:\:{C}=\begin{pmatrix}{\frac{−{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} }{\mathrm{2}{c}}}\\{\frac{\delta}{\mathrm{2}{c}}}\end{pmatrix} \\ $$$${D}=\frac{{B}+{C}}{\mathrm{2}}=\begin{pmatrix}{\frac{−{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +\mathrm{3}{c}^{\mathrm{2}} }{\mathrm{4}{c}}}\\{\frac{\delta}{\mathrm{4}{c}}}\end{pmatrix} \\ $$$${E}=\frac{{A}+{D}}{\mathrm{2}}=\frac{\mathrm{2}{A}+{B}+{C}}{\mathrm{4}}=\begin{pmatrix}{\frac{−{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +\mathrm{3}{c}^{\mathrm{2}} }{\mathrm{8}{c}}}\\{\frac{\delta}{\mathrm{8}{c}}}\end{pmatrix} \\ $$$${line}\:{BE}:\:{y}=\frac{\delta}{{a}^{\mathrm{2}} −{b}^{\mathrm{2}} +\mathrm{5}{c}^{\mathrm{2}} }\left({c}−{x}\right) \\ $$$${line}\:{AC}:\:{y}=\frac{\delta}{−{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} }{x} \\ $$$${F}={BE}\cap{AC}=\begin{pmatrix}{\frac{−{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} }{\mathrm{6}{c}}}\\{\frac{\delta}{\mathrm{6}{c}}}\end{pmatrix} \\ $$$$\mid{AF}\mid=\frac{\sqrt{\delta^{\mathrm{2}} +\left(−{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)^{\mathrm{2}} }}{\mathrm{6}{c}} \\ $$$$\mid{FC}\mid=\frac{\sqrt{\delta^{\mathrm{2}} +\left(−{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)^{\mathrm{2}} }}{\mathrm{3}{c}} \\ $$$$\frac{\mid{AF}\mid}{\mid{FC}\mid}=\frac{\mathrm{1}}{\mathrm{2}} \\ $$

Commented by rahul 19 last updated on 20/Aug/18

Sir, how u write coordinates of C?

$$\mathrm{Sir},\:\mathrm{how}\:\mathrm{u}\:\mathrm{write}\:\mathrm{coordinates}\:\mathrm{of}\:\mathrm{C}? \\ $$

Commented by MJS last updated on 20/Aug/18

a=BC b=CA c=AB I put A = point of origin and AB on x−axis ⇒ ⇒ B= ((c),(0) ) to find C we have 2 rectangular triangles c=p+q ⇒ p^2 +h_c ^2 =b^2 ∧ q^2 +h_c ^2 =a^2 C= ((p),(h_c ) ) we know that the area of ABC is (1) ((ch_c )/2) (2) (δ/4) ((ch_c )/2)=(δ/4) ⇒ h_c =(δ/(2c)) p^2 +h_c ^2 =b^2 p^2 =b^2 −h_c ^2 p^2 =((4b^2 c^2 −δ^2 )/(4c^2 )) [δ^2 =2(a^2 b^2 +a^2 c^2 +b^2 c^2 )−(a^4 +b^4 +c^4 )] p^2 =((a^4 +b^4 +c^4 −2a^2 b^2 −2a^2 c^2 +2b^2 c^2 )/(4c^2 )) p^2 =(((−a^2 +b^2 +c^2 )^2 )/(4c^2 )) p=((−a^2 +b^2 +c^2 )/(4c^2 )) C= ((p),(h_c ) ) = ((((−a^2 +b^2 +c^2 )/(4c^2 ))),((δ/(2c))) )

$${a}={BC}\:\:{b}={CA}\:\:{c}={AB} \\ $$$$\mathrm{I}\:\mathrm{put}\:{A}\:=\:\mathrm{point}\:\mathrm{of}\:\mathrm{origin}\:\mathrm{and}\:{AB}\:\mathrm{on}\:{x}−\mathrm{axis}\:\Rightarrow \\ $$$$\Rightarrow\:{B}=\begin{pmatrix}{{c}}\\{\mathrm{0}}\end{pmatrix} \\ $$$$\mathrm{to}\:\mathrm{find}\:{C}\:\mathrm{we}\:\mathrm{have}\:\mathrm{2}\:\mathrm{rectangular}\:\mathrm{triangles} \\ $$$${c}={p}+{q}\:\Rightarrow\:{p}^{\mathrm{2}} +{h}_{{c}} ^{\mathrm{2}} ={b}^{\mathrm{2}} \:\wedge\:{q}^{\mathrm{2}} +{h}_{{c}} ^{\mathrm{2}} ={a}^{\mathrm{2}} \\ $$$${C}=\begin{pmatrix}{{p}}\\{{h}_{{c}} }\end{pmatrix} \\ $$$$\mathrm{we}\:\mathrm{know}\:\mathrm{that}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:{ABC}\:\mathrm{is} \\ $$$$\left(\mathrm{1}\right)\:\frac{{ch}_{{c}} }{\mathrm{2}} \\ $$$$\left(\mathrm{2}\right)\:\frac{\delta}{\mathrm{4}} \\ $$$$\frac{{ch}_{{c}} }{\mathrm{2}}=\frac{\delta}{\mathrm{4}}\:\Rightarrow\:{h}_{{c}} =\frac{\delta}{\mathrm{2}{c}} \\ $$$${p}^{\mathrm{2}} +{h}_{{c}} ^{\mathrm{2}} ={b}^{\mathrm{2}} \\ $$$${p}^{\mathrm{2}} ={b}^{\mathrm{2}} −{h}_{{c}} ^{\mathrm{2}} \\ $$$${p}^{\mathrm{2}} =\frac{\mathrm{4}{b}^{\mathrm{2}} {c}^{\mathrm{2}} −\delta^{\mathrm{2}} }{\mathrm{4}{c}^{\mathrm{2}} } \\ $$$$\:\:\:\:\:\left[\delta^{\mathrm{2}} =\mathrm{2}\left({a}^{\mathrm{2}} {b}^{\mathrm{2}} +{a}^{\mathrm{2}} {c}^{\mathrm{2}} +{b}^{\mathrm{2}} {c}^{\mathrm{2}} \right)−\left({a}^{\mathrm{4}} +{b}^{\mathrm{4}} +{c}^{\mathrm{4}} \right)\right] \\ $$$${p}^{\mathrm{2}} =\frac{{a}^{\mathrm{4}} +{b}^{\mathrm{4}} +{c}^{\mathrm{4}} −\mathrm{2}{a}^{\mathrm{2}} {b}^{\mathrm{2}} −\mathrm{2}{a}^{\mathrm{2}} {c}^{\mathrm{2}} +\mathrm{2}{b}^{\mathrm{2}} {c}^{\mathrm{2}} }{\mathrm{4}{c}^{\mathrm{2}} } \\ $$$${p}^{\mathrm{2}} =\frac{\left(−{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)^{\mathrm{2}} }{\mathrm{4}{c}^{\mathrm{2}} } \\ $$$${p}=\frac{−{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} }{\mathrm{4}{c}^{\mathrm{2}} } \\ $$$${C}=\begin{pmatrix}{{p}}\\{{h}_{{c}} }\end{pmatrix}\:=\begin{pmatrix}{\frac{−{a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} }{\mathrm{4}{c}^{\mathrm{2}} }}\\{\frac{\delta}{\mathrm{2}{c}}}\end{pmatrix} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com