Math Question and Answers


Question and Answers Forum

All Questions Topic List
Differentiation Questions
Previous in All Question Next in All Question
Previous in Differentiation Next in Differentiation
Question Number 42446 by ajfour last updated on 25/Aug/18

Commented by ajfour last updated on 26/Aug/18

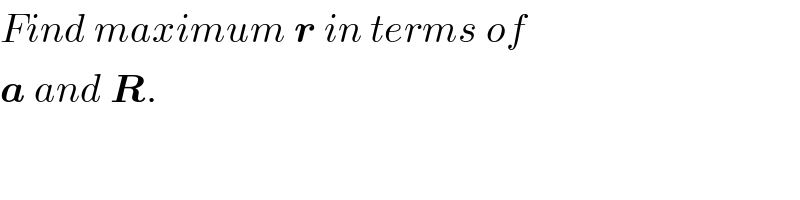
$F i n d m a x i m u m r i n t e r m s o f$ $a a n d R .$
	Answered by tanmay.chaudhury50@gmail.com last updated on 26/Aug/18

	$s o l v e x^{2} + {(y - R)}^{2} = R^{2} a n d y = {a x}^{2}$ $x^{2} + y^{2} - 2 y R = 0$ $x^{2} + a^{2} x^{4} - 2 {a x}^{2} R = 0$ $x^{2} (1 + a^{2} x^{2} - 2 a R) = 0$ $a^{2} x^{2} = 2 a R - 1$ $x = \frac{\pm \sqrt{2 a R - 1}}{a}$ $y = {a x}^{2} = a (\frac{2 a R - 1}{a^{2}}) = \frac{2 a R - 1}{a}$ $p o i n t o f i n t e r s e c t i o n a r e$ $(\frac{\sqrt{2 a R - 1}}{a}, \frac{2 a R - 1}{a}) a n d (\frac{- \sqrt{2 a R - 1}}{a}, \frac{2 a R - 1}{a})$ $c o n s i d e r i n g + v e s i d e$ $n o w a r e a b e t w e e n y = {a x}^{2} a n d x^{2} + y^{2} - 2 y R = 0$ $a n d y a x i s s a y = A r e m a i n i n g a r e a b e t w e e n$ $p a r a b o l a a n d c i r c l e = B$ $s o 2 A + 2 B = Π R^{2}$ $B = \frac{Π R^{2}}{2} - A$ $n o w a r e a B = Π r^{2} + r e m a i n i n g a r e a s y m e t r i c a l$ $w . r . t Π r^{2}$ $B = Π r^{2} + 2 S$ $t o m a x i m i z e B$ $c o n t d$
	Answered by MJS last updated on 27/Aug/18

	$tangents parallel but not of interest$ $normals in tangential points must be the same$ $distance between these points = 2 r$ $y = {a x}^{2}$ $T_{p} = (\begin{matrix} p \\ {a p}^{2} \end{matrix})$ $n_{p} : y = k x + d$ $k = - \frac{1}{y^{'}} = - \frac{1}{2 a p} d = \frac{2 a^{2} p^{2} + 1}{2 a}$ $n_{p} : - \frac{1}{2 a p} x + \frac{2 a^{2} p^{2} + 1}{2 a}$ $y = R - \sqrt{R^{2} - x^{2}}$ $T_{c} = (\begin{matrix} q \\ R - \sqrt{R^{2} - q^{2}} \end{matrix})$ $n_{c} : y = k x + d$ $k = - \frac{1}{y^{'}} = - \frac{\sqrt{R^{2} - q^{2}}}{q} d = R$ $n_{c} : y = - \frac{\sqrt{R^{2} - q^{2}}}{q} x + R$ $n_{p} = n_{c}$ $(1) - \frac{1}{2 a p} = - \frac{\sqrt{R^{2} - q^{2}}}{q}$ $(2) \frac{2 a^{2} p^{2} + 1}{2 a} = R$ $\Rightarrow p = - \frac{\sqrt{2 (2 a R - 1)}}{2 a} \land q = - R \sqrt{\frac{2 (2 a R - 1)}{4 a R - 1}}$ $T_{p} = (\begin{matrix} - \frac{\sqrt{2 (2 a R - 1)}}{2 a} \\ \frac{2 a r - 1}{2 a} \end{matrix}) T_{c} = (\begin{matrix} - R \sqrt{\frac{2 (2 a R - 1)}{4 a R - 1}} \\ R (\frac{- 1 + \sqrt{4 a R - 1}}{\sqrt{4 a R - 1}}) \end{matrix})$ $r = \frac{∣ T_{p} T_{c} ∣}{2} = \frac{\sqrt{a R (a R + 1 - \frac{1}{4 a R} - \sqrt{4 a R - 1})}}{2 a}$ $please check, if the idea is ok . . .$
	Commented by ajfour last updated on 27/Aug/18

	$N o s i r, i h a d c o m m i t e d s o m e e r r o r;$ $i h a v e n o t b e e n a b l e t o s o l v e i t y e t . .$
	Commented by MJS last updated on 27/Aug/18

	$ok I^{'} ll check mine . . .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum