
Question and Answers Forum
Question Number 42487 by maxmathsup by imad last updated on 26/Aug/18

Commented by maxmathsup by imad last updated on 28/Aug/18
![1) we have f(x) = ∫_0 ^1 (dt/(x+((e^t +e^(−t) )/2))) =∫_0 ^1 ((2dt)/(2x +e^t +e^(−t) )) changement e^t =u give f(x) = ∫_1 ^e (2/(2x +u +(1/u))) (du/u) = ∫_1 ^e ((2du)/(2xu +u^2 +1)) = ∫_1 ^x ((2du)/(u^2 +2xu +1)) Δ^′ =x^2 −1 case 1 ∣x∣>1 ⇒u_1 =−x+(√(x^2 −1)) and u_2 =−x−(√(x^2 −1)) ⇒ F(u) = (2/(u^2 +2xu +1)) = (a/(u−u_1 )) +(b/(u−u_2 )) (=(2/((u−u_1 )(u−u_2 )))) a = (2/(u_1 −u_2 )) =(2/(2(√(x^2 −1)))) =(1/(√(x^2 −1))) b =(2/(u_2 −u_1 )) =(2/(−2(√(x^2 −1)))) =−(1/(√(x^2 −1))) ⇒ f(x) = ∫_1 ^e F(u)du =(1/(√(x^2 −1))) ∫_1 ^e ( (1/(u−u_1 )) −(1/(u−u_2 )))du =(1/(√(x^2 −1)))[ln∣((u−u_1 )/(u−u_2 ))∣]_1 ^e =(1/(√(x^2 −1))){ln∣ ((e+x−(√(x^2 −1)))/(e+x+(√(x^2 −1))))∣ −ln∣((1+x−(√(x^2 −1)))/(1+x +(√(x^2 −1))))∣} case 2 ∣x∣<1 ⇒f(x)= ∫_1 ^e ((2du)/(u^2 +2xu +x^2 +1−x^2 )) =∫_1 ^e ((2du)/((u+x)^2 +1−x^2 )) =_(u+x =(√(1−x^2 ))α) ∫_((1+x)/(√(1−x^2 ))) ^((e+x)/(√(1−x^2 ))) ((2(√(1−x^2 ))dα)/((1−x^2 )(1+α^2 ))) =(2/(√(1−x^2 ))) [ arctan(α)]_((1+x)/(√(1−x^2 ))) ^((e+x)/(√(1−x^2 ))) =(2/(√(1−x^2 ))){ arctan(((e+x)/(√(1−x^2 ))))−arctan(((1+x)/(√(1−x^2 ))))} .](Q42576.png)
Commented by maxmathsup by imad last updated on 28/Aug/18
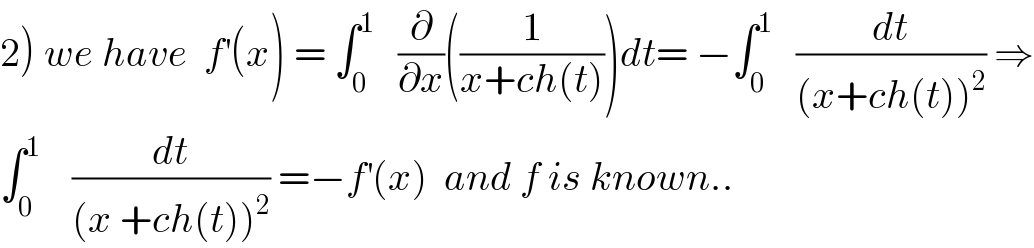
Commented by maxmathsup by imad last updated on 28/Aug/18

