
Question and Answers Forum
Question Number 43386 by ajfour last updated on 10/Sep/18
Commented by ajfour last updated on 10/Sep/18

Commented by Tawa1 last updated on 10/Sep/18

Commented by ajfour last updated on 10/Sep/18

Commented by Tawa1 last updated on 10/Sep/18

Answered by MrW3 last updated on 10/Sep/18
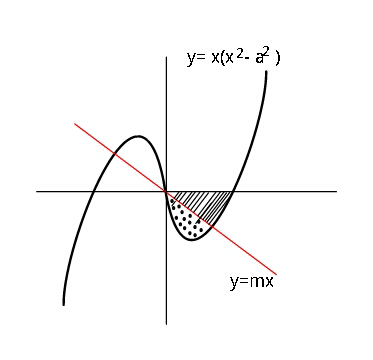
![y=x(x^2 −a^2 ) (dy/dx)=3x^2 −a^2 at x=0: tan θ_0 =−a^2 ⇒r sin θ=r cos θ(r^2 cos^2 θ−a^2 ) ⇒r^2 =((tan θ+a^2 )/(cos^2 θ)) A=∫_θ_0 ^(2π) ((r^2 dθ)/2)=(1/2)∫_θ_0 ^(2π) ((tan θ+a^2 )/(cos^2 θ)) dθ =(1/2)∫_θ_0 ^(2π) (tan θ+a^2 ) d(tan θ) =(1/2)[((tan^2 θ)/2)+a^2 tan θ]_θ_0 ^(2π) =(1/2)[−(a^4 /2)+a^4 ]=(a^4 /4) let tan θ_1 =m A_1 =(1/2)[((tan^2 θ)/2)+a^2 tan θ]_θ_1 ^(2π) =(1/2)[−((tan^2 θ_1 )/2)−a^2 tan θ_1 ] =−(1/2)[(m^2 /2)+a^2 m]=(A/2)=(a^4 /8) ⇒2m^2 +4a^2 m+a^4 =0 ⇒m=((−4a^2 ±(√(16a^4 −8a^4 )))/4) = { ((−((2+(√2))/2)a^2 )),((−((2−(√2))/2)a^2 )) :} since ∣θ_1 ∣<∣θ_0 ∣⇒∣m∣=∣tan θ_1 ∣<∣tan θ_0 ∣=a^2 the suitable solution is: m=−((2−(√2))/2)a^2 ≈−0.293a^2](Q43399.png)
Commented by ajfour last updated on 10/Sep/18

Answered by ajfour last updated on 10/Sep/18
![Intersection points of line and curve: y=mx=x(x^2 −a^2 ) ⇒ O(0,0) and P ((√(a^2 +m)) , y_P ) A=−∫_0 ^( a) (x^3 −a^2 x)dx =−( (a^4 /4)−(a^4 /2)) ⇒ A=(a^4 /4) ∫_0 ^( (√(a^2 +m))) (mx−x^3 +a^2 x)dx = (a^4 /8) (=(A/2)) ⇒ ((m(a^2 +m))/2)−(((a^2 +m)^2 )/4)+((a^2 (a^2 +m))/2) = (a^4 /8) ⇒ (a^2 +m)^2 = (a^4 /2) ⇒ m= ((1/(√2))−1)a^2 same as m= −(((2−(√2))/2))a^2 . [ (dy/dx)∣_(x=0) = −a^2 ; so we reject m=−(1+(1/(√2)))a^2 ].](Q43405.png)
Answered by tanmay.chaudhury50@gmail.com last updated on 10/Sep/18
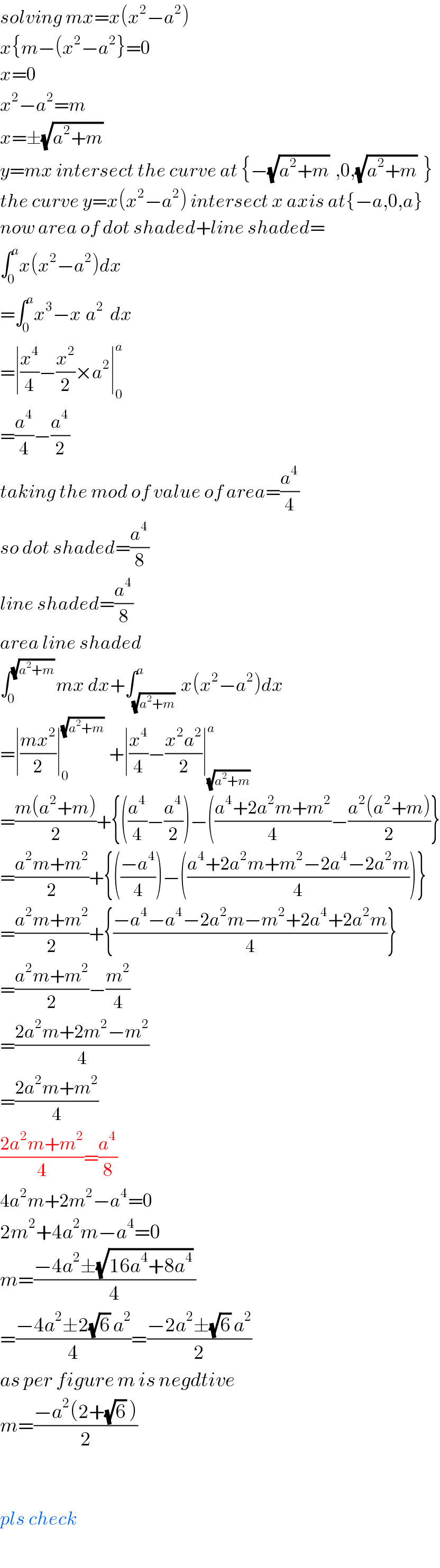
Commented by ajfour last updated on 10/Sep/18
Commented by tanmay.chaudhury50@gmail.com last updated on 10/Sep/18
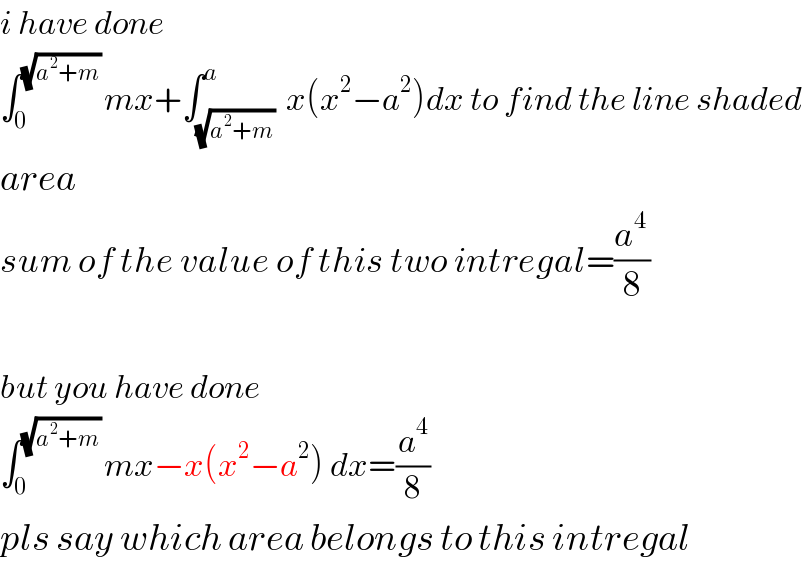
Commented by ajfour last updated on 10/Sep/18

Commented by tanmay.chaudhury50@gmail.com last updated on 10/Sep/18
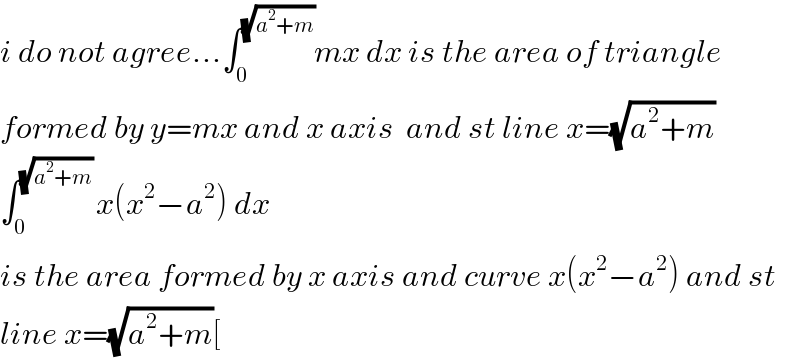
Commented by tanmay.chaudhury50@gmail.com last updated on 10/Sep/18

Commented by ajfour last updated on 10/Sep/18

Commented by tanmay.chaudhury50@gmail.com last updated on 10/Sep/18
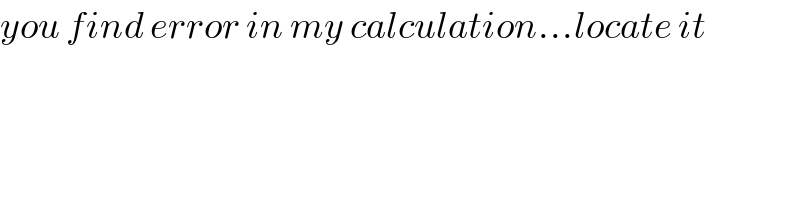
Commented by ajfour last updated on 10/Sep/18
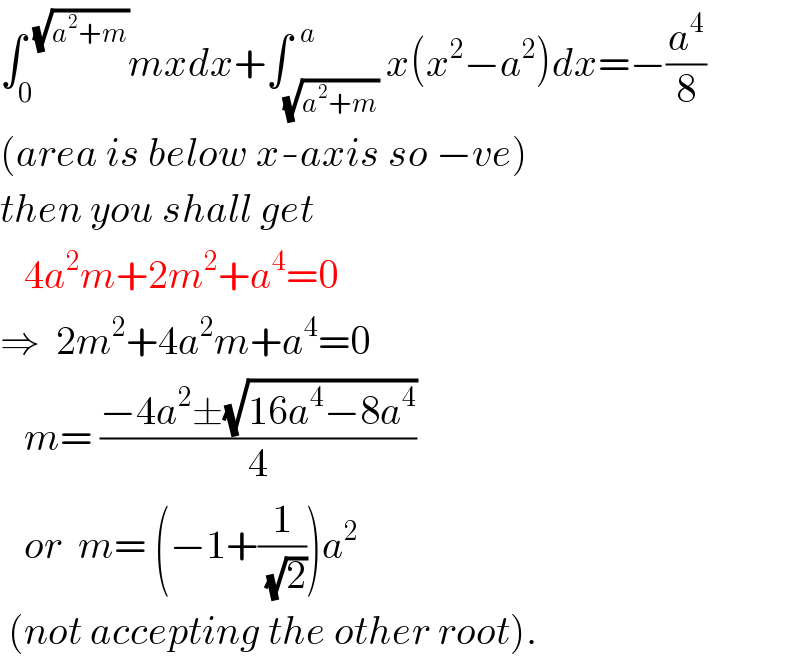
Commented by tanmay.chaudhury50@gmail.com last updated on 11/Sep/18

Answered by MJS last updated on 10/Sep/18
![p: y=x^3 −a^2 x l: y=mx p∩l x^3 −(m+a^2 )x=0 ⇒ x=(√(m+a^2 )) dotted area: D=∫_0 ^(√(m+a^2 )) (x^3 −a^2 x)dx−m∫_0 ^(√(m+a^2 )) xdx shaded area: S=m∫_0 ^(√(m+a^2 )) xdx+∫_(√(m+a^2 )) ^a (x^3 −a^2 x)dx D=(1/4)(m^2 −a^4 )−(1/2)m(m+a^2 )=−(1/4)(m+a^2 )^2 S=(1/2)m(m+a^2 )−(1/4)m^2 =(1/4)m(m+2a^2 ) S−D=0 m^2 +2a^2 m+(a^4 /2)=0 m=(−1+((√2)/2))a^2 [the 2^(nd) solution doesn′t give a real value for (√(m+a^2 ))]](Q43439.png)
