Question and Answers Forum

All Questions      Topic List

Mechanics Questions

Previous in All Question      Next in All Question      

Previous in Mechanics      Next in Mechanics      

Question Number 43585 by ajfour last updated on 12/Sep/18

Commented by ajfour last updated on 12/Sep/18

The small ball has radius r, while the larger ball has radius R, they collide on smooth horizontal ground, impact parameter is equal to r. The small ball hits the larger ball (at rest initially) with speed u. Find 𝛉, 𝛗, v, and V in terms of m, M, r, R . (collision is completly elastic).

$${The}\:{small}\:{ball}\:{has}\:{radius}\:\boldsymbol{{r}},\: \\ $$$${while}\:{the}\:{larger}\:{ball}\:{has}\:{radius} \\ $$$$\boldsymbol{{R}},\:{they}\:{collide}\:{on}\:{smooth} \\ $$$${horizontal}\:{ground},\:{impact} \\ $$$${parameter}\:{is}\:{equal}\:{to}\:\boldsymbol{{r}}. \\ $$$${The}\:{small}\:{ball}\:{hits}\:{the}\:{larger} \\ $$$${ball}\:\left({at}\:{rest}\:{initially}\right)\:{with} \\ $$$${speed}\:\boldsymbol{{u}}. \\ $$$$\boldsymbol{{Find}}\:\boldsymbol{\theta},\:\boldsymbol{\phi},\:\boldsymbol{{v}},\:{and}\:\boldsymbol{{V}}\:\:{in}\:{terms}\:{of} \\ $$$$\:\boldsymbol{{m}},\:\boldsymbol{{M}},\:\boldsymbol{{r}},\:\boldsymbol{{R}}\:. \\ $$$$\left({collision}\:{is}\:{completly}\:{elastic}\right). \\ $$

Commented by MrW3 last updated on 12/Sep/18

Now I know you are a curling sport fan.

$${Now}\:{I}\:\:{know}\:{you}\:{are}\:{a}\:{curling}\:{sport} \\ $$$${fan}. \\ $$

Answered by MrW3 last updated on 14/Sep/18

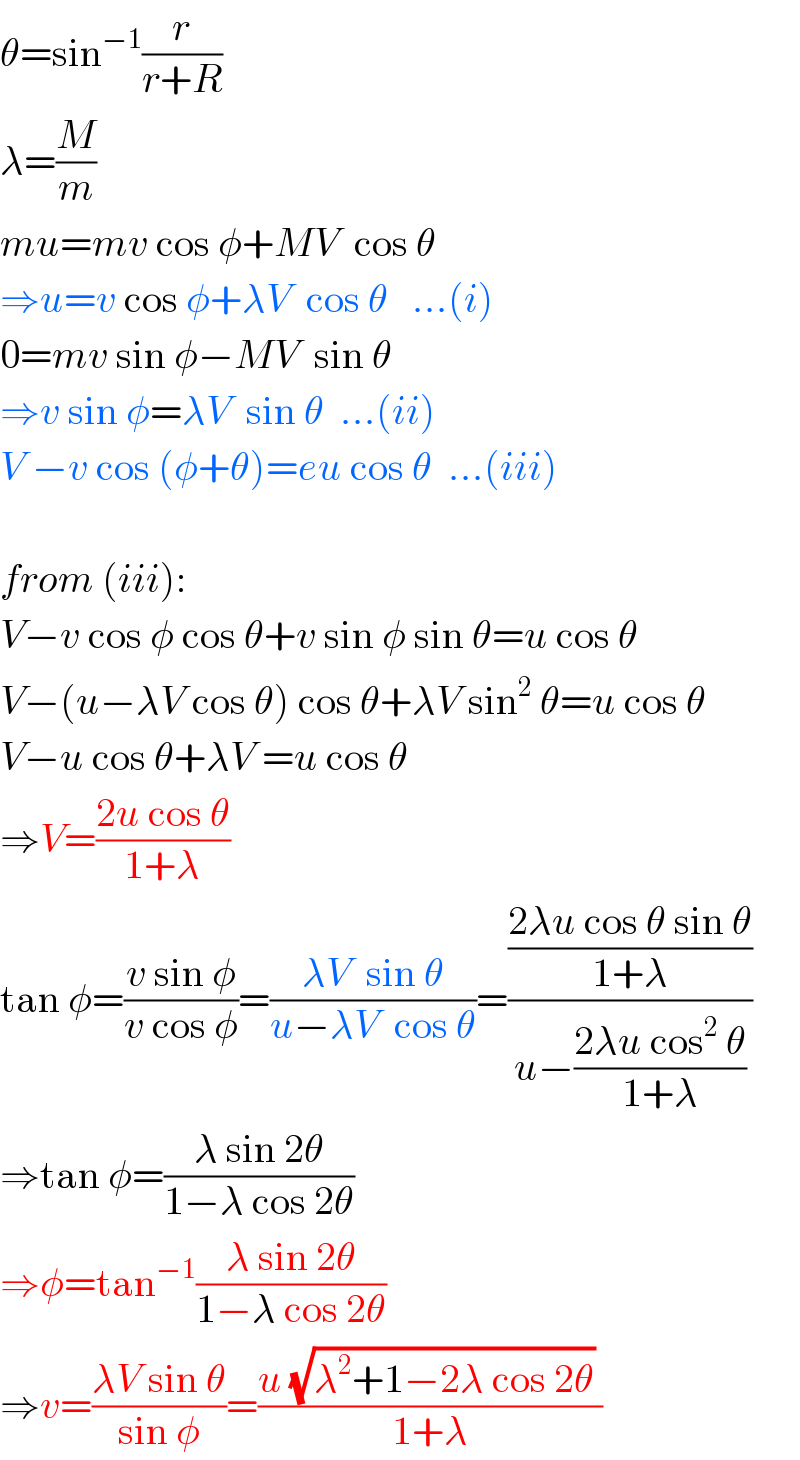
θ=sin^(−1) (r/(r+R)) λ=(M/m) mu=mv cos φ+MV cos θ ⇒u=v cos φ+λV cos θ ...(i) 0=mv sin φ−MV sin θ ⇒v sin φ=λV sin θ ...(ii) V −v cos (φ+θ)=eu cos θ ...(iii) from (iii): V−v cos φ cos θ+v sin φ sin θ=u cos θ V−(u−λV cos θ) cos θ+λV sin^2 θ=u cos θ V−u cos θ+λV =u cos θ ⇒V=((2u cos θ)/(1+λ)) tan φ=((v sin φ)/(v cos φ))=((λV sin θ)/(u−λV cos θ))=(((2λu cos θ sin θ)/(1+λ))/(u−((2λu cos^2 θ)/(1+λ)))) ⇒tan φ=((λ sin 2θ)/(1−λ cos 2θ)) ⇒φ=tan^(−1) ((λ sin 2θ)/(1−λ cos 2θ)) ⇒v=((λV sin θ)/(sin φ))=((u (√(λ^2 +1−2λ cos 2θ)) )/(1+λ))

$$\theta=\mathrm{sin}^{−\mathrm{1}} \frac{{r}}{{r}+{R}} \\ $$$$\lambda=\frac{{M}}{{m}} \\ $$$${mu}={mv}\:\mathrm{cos}\:\phi+{MV}\:\:\mathrm{cos}\:\theta \\ $$$$\Rightarrow{u}={v}\:\mathrm{cos}\:\phi+\lambda{V}\:\:\mathrm{cos}\:\theta\:\:\:...\left({i}\right) \\ $$$$\mathrm{0}={mv}\:\mathrm{sin}\:\phi−{MV}\:\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow{v}\:\mathrm{sin}\:\phi=\lambda{V}\:\:\mathrm{sin}\:\theta\:\:...\left({ii}\right) \\ $$$${V}\:−{v}\:\mathrm{cos}\:\left(\phi+\theta\right)={eu}\:\mathrm{cos}\:\theta\:\:...\left({iii}\right) \\ $$$$ \\ $$$${from}\:\left({iii}\right): \\ $$$${V}−{v}\:\mathrm{cos}\:\phi\:\mathrm{cos}\:\theta+{v}\:\mathrm{sin}\:\phi\:\mathrm{sin}\:\theta={u}\:\mathrm{cos}\:\theta \\ $$$${V}−\left({u}−\lambda{V}\:\mathrm{cos}\:\theta\right)\:\mathrm{cos}\:\theta+\lambda{V}\:\mathrm{sin}^{\mathrm{2}} \:\theta={u}\:\mathrm{cos}\:\theta \\ $$$${V}−{u}\:\mathrm{cos}\:\theta+\lambda{V}\:={u}\:\mathrm{cos}\:\theta \\ $$$$\Rightarrow{V}=\frac{\mathrm{2}{u}\:\mathrm{cos}\:\theta}{\mathrm{1}+\lambda} \\ $$$$\mathrm{tan}\:\phi=\frac{{v}\:\mathrm{sin}\:\phi}{{v}\:\mathrm{cos}\:\phi}=\frac{\lambda{V}\:\:\mathrm{sin}\:\theta}{{u}−\lambda{V}\:\:\mathrm{cos}\:\theta}=\frac{\frac{\mathrm{2}\lambda{u}\:\mathrm{cos}\:\theta\:\mathrm{sin}\:\theta}{\mathrm{1}+\lambda}}{{u}−\frac{\mathrm{2}\lambda{u}\:\mathrm{cos}^{\mathrm{2}} \:\theta}{\mathrm{1}+\lambda}} \\ $$$$\Rightarrow\mathrm{tan}\:\phi=\frac{\lambda\:\mathrm{sin}\:\mathrm{2}\theta}{\mathrm{1}−\lambda\:\mathrm{cos}\:\mathrm{2}\theta} \\ $$$$\Rightarrow\phi=\mathrm{tan}^{−\mathrm{1}} \frac{\lambda\:\mathrm{sin}\:\mathrm{2}\theta}{\mathrm{1}−\lambda\:\mathrm{cos}\:\mathrm{2}\theta} \\ $$$$\Rightarrow{v}=\frac{\lambda{V}\:\mathrm{sin}\:\theta}{\mathrm{sin}\:\phi}=\frac{{u}\:\sqrt{\lambda^{\mathrm{2}} +\mathrm{1}−\mathrm{2}\lambda\:\mathrm{cos}\:\mathrm{2}\theta}\:}{\mathrm{1}+\lambda} \\ $$

Commented by ajfour last updated on 14/Sep/18

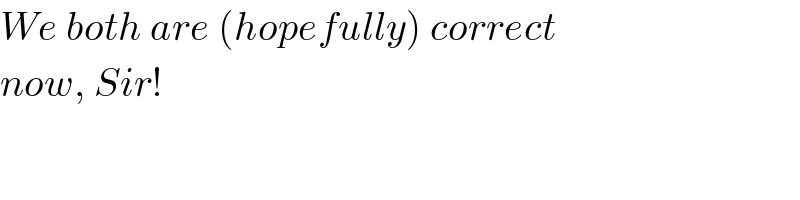
We both are (hopefully) correct now, Sir!

$${We}\:{both}\:{are}\:\left({hopefully}\right)\:{correct} \\ $$$${now},\:{Sir}! \\ $$

Commented by MrW3 last updated on 14/Sep/18

thanks for helping me to get the right solution!

$${thanks}\:{for}\:{helping}\:{me}\:{to}\:{get}\:{the} \\ $$$${right}\:{solution}! \\ $$

Answered by ajfour last updated on 14/Sep/18

sin θ = (r/(R+r)) MVcos θ +mvcos φ = mu ...(i) MVsin θ = mvsin φ ....(ii) V−vcos (θ+φ)= ucos θ ....(iii) using (ii) in (i) MVcos θ+MVsin θcot φ=mu let (m/M)=q ⇒ V(cos θ+sin θcot φ)=qu ...(a) using (ii) in (iii) V−((MVsin θcos (θ+φ))/(msin φ)) = ucos θ V(1−((sin θcos (θ+φ))/(qsin φ)))=ucos θ ..(b) eq.(b) ÷ eq.(a) gives (([1−((sin θcos (θ+φ))/(qsin φ))])/(cos θ+sin θcot φ)) = ((cos θ)/q) ⇒ qsin φ−sin θcos θcos φ +sin^2 θsin φ = cos^2 θsin φ +sin θcos θcos φ ⇒ (q−cos^2 θ+sin^2 θ)sin φ = 2sin θcos θcos φ ________________________ or tan φ = ((sin 2θ)/(q−cos 2θ)) ________________________ Now V=((qu)/(cos θ+sin θcot φ)) [see(a)] = ((qusin 2θ)/(cos θsin 2θ+qsin θ−sin θcos 2θ)) ________________________ ⇒ V = ((2qucos θ)/(1+q)) ________________________ v = ((MVsin θ)/(msin φ)) = ((2usin θcos θ)/(1+q))×((√(sin^2 2θ+(q−cos 2θ)^2 ))/(sin 2θ)) ________________________ v = u(((√(1+q^2 −2qcos 2θ))/(1+q)) ) ________________________ .

$$\mathrm{sin}\:\theta\:=\:\frac{{r}}{{R}+{r}}\: \\ $$$${MV}\mathrm{cos}\:\theta\:+{mv}\mathrm{cos}\:\phi\:=\:{mu}\:\:\:...\left({i}\right) \\ $$$${MV}\mathrm{sin}\:\theta\:=\:{mv}\mathrm{sin}\:\phi\:\:\:\:\:\:\:\:\:\:\:\:\:....\left({ii}\right) \\ $$$${V}−{v}\mathrm{cos}\:\left(\theta+\phi\right)=\:{u}\mathrm{cos}\:\theta\:\:\:\:....\left({iii}\right) \\ $$$${using}\:\left({ii}\right)\:{in}\:\left({i}\right) \\ $$$${MV}\mathrm{cos}\:\theta+{MV}\mathrm{sin}\:\theta\mathrm{cot}\:\phi={mu}\: \\ $$$${let}\:\frac{{m}}{{M}}={q} \\ $$$$\Rightarrow\:\:{V}\left(\mathrm{cos}\:\theta+\mathrm{sin}\:\theta\mathrm{cot}\:\phi\right)={qu}\:\:\:...\left({a}\right) \\ $$$${using}\:\left({ii}\right)\:{in}\:\left({iii}\right) \\ $$$${V}−\frac{{MV}\mathrm{sin}\:\theta\mathrm{cos}\:\left(\theta+\phi\right)}{{m}\mathrm{sin}\:\phi}\:=\:{u}\mathrm{cos}\:\theta\:\: \\ $$$${V}\left(\mathrm{1}−\frac{\mathrm{sin}\:\theta\mathrm{cos}\:\left(\theta+\phi\right)}{{q}\mathrm{sin}\:\phi}\right)={u}\mathrm{cos}\:\theta\:\:..\left({b}\right) \\ $$$${eq}.\left({b}\right)\:\boldsymbol{\div}\:{eq}.\left({a}\right)\:\:{gives} \\ $$$$\frac{\left[\mathrm{1}−\frac{\mathrm{sin}\:\theta\mathrm{cos}\:\left(\theta+\phi\right)}{{q}\mathrm{sin}\:\phi}\right]}{\mathrm{cos}\:\theta+\mathrm{sin}\:\theta\mathrm{cot}\:\phi}\:=\:\frac{\mathrm{cos}\:\theta}{{q}} \\ $$$$ \\ $$$$\Rightarrow\:{q}\mathrm{sin}\:\phi−\mathrm{sin}\:\theta\mathrm{cos}\:\theta\mathrm{cos}\:\phi \\ $$$$\:\:\:+\mathrm{sin}\:^{\mathrm{2}} \theta\mathrm{sin}\:\phi\:=\:\mathrm{cos}\:^{\mathrm{2}} \theta\mathrm{sin}\:\phi \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:+\mathrm{sin}\:\theta\mathrm{cos}\:\theta\mathrm{cos}\:\phi \\ $$$$\Rightarrow\:\left({q}−\mathrm{cos}\:^{\mathrm{2}} \theta+\mathrm{sin}\:^{\mathrm{2}} \theta\right)\mathrm{sin}\:\phi \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{2sin}\:\theta\mathrm{cos}\:\theta\mathrm{cos}\:\phi \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$${or}\:\:\:\mathrm{tan}\:\phi\:=\:\frac{\mathrm{sin}\:\mathrm{2}\theta}{{q}−\mathrm{cos}\:\mathrm{2}\theta} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$${Now}\:\:\:{V}=\frac{{qu}}{\mathrm{cos}\:\theta+\mathrm{sin}\:\theta\mathrm{cot}\:\phi}\:\:\:\left[{see}\left({a}\right)\right] \\ $$$$\:\:\:\:\:\:\:\:=\:\frac{{qu}\mathrm{sin}\:\mathrm{2}\theta}{\mathrm{cos}\:\theta\mathrm{sin}\:\mathrm{2}\theta+{q}\mathrm{sin}\:\theta−\mathrm{sin}\:\theta\mathrm{cos}\:\mathrm{2}\theta} \\ $$$$ \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$$\:\:\:\:\Rightarrow\:\:\boldsymbol{{V}}\:=\:\frac{\mathrm{2}{qu}\mathrm{cos}\:\theta}{\mathrm{1}+{q}}\:\: \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$$\:\:\:\:\:\:\:\:\:{v}\:=\:\frac{{MV}\mathrm{sin}\:\theta}{{m}\mathrm{sin}\:\phi} \\ $$$$\:\:\:\:\:\:=\:\frac{\mathrm{2}{u}\mathrm{sin}\:\theta\mathrm{cos}\:\theta}{\mathrm{1}+{q}}×\frac{\sqrt{\mathrm{sin}\:^{\mathrm{2}} \mathrm{2}\theta+\left({q}−\mathrm{cos}\:\mathrm{2}\theta\right)^{\mathrm{2}} }}{\mathrm{sin}\:\mathrm{2}\theta} \\ $$$$ \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$$\:\:\boldsymbol{{v}}\:=\:\boldsymbol{{u}}\left(\frac{\sqrt{\mathrm{1}+{q}^{\mathrm{2}} −\mathrm{2}{q}\mathrm{cos}\:\mathrm{2}\theta}}{\mathrm{1}+{q}}\:\right)\: \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\:. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com