Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 43907 by abdo.msup.com last updated on 17/Sep/18

find ∫ ((cos^2 x −sin^2 x)/(3 +tan^2 x))dx

$${find}\:\int\:\:\frac{{cos}^{\mathrm{2}} {x}\:−{sin}^{\mathrm{2}} {x}}{\mathrm{3}\:+{tan}^{\mathrm{2}} {x}}{dx} \\ $$

Commented by maxmathsup by imad last updated on 18/Sep/18

let I = ∫ ((cos^2 x −sin^2 x)/(3+tan^2 x))dx ⇒ I = ∫ ((cos(2x))/(3+tan^2 x))dx changement tanx =t give I = ∫ (((1−t^2 )/(1+t^2 ))/(3+t^2 )) (dt/(1+t^2 )) = ∫ ((1−t^2 )/((1+t^2 )^2 (3+t^2 )))dt let decokpose F(t)=((1−t^2 )/((1+t^2 )^2 (3+t^2 ))) F(t) =((at +b)/(t^2 +1)) +((ct +d)/((t^2 +1)^2 )) +((et +f)/(t^2 +3)) F(−t) =F(t) ⇒((−at +b)/(t^2 +1)) +((−ct +d)/((t^(2 ) +1)^2 )) +((−et +f)/(t^2 +3)) =F(t) ⇒a=0 ,c=0 and e=0 ⇒ F(t)= (b/(t^2 +1)) +(d/((t^2 +1)^2 )) +(f/(t^2 +3)) lim_(t→+∞) t^2 F(t)=0 =b+f ⇒f =−b ⇒F(t)=(b/(t^2 +1)) +(d/((t^2 +1)^2 )) −(b/(t^2 +3)) F(o) =(1/3) = b +d−(b/3) ⇒1=3b+3d−b =2b +3d ⇒3d=1−2b ⇒d=((1−2b)/3) F(t) = (b/(t^2 +1)) +((1−2b)/(3(t^2 +1)^2 )) −(b/(t^2 +3)) F(1)=0 =(b/2) +((1−2b)/(12)) −(b/4) ⇒0 =6b +1−2b−3b ⇒b+1 =0 ⇒b=−1 ⇒ F(t) =−(1/(t^2 +1)) +(1/((t^2 +1)^2 )) +(1/(t^2 +3)) ⇒ I =−∫ (dt/(t^2 +1)) + ∫ (dt/((t^2 +1)^2 )) +∫ (dt/(t^2 +3)) but ∫ (dt/(t^2 +1)) =arctant +c_1 ∫ (dt/(t^2 +3)) =_(t=(√3)u) ∫ (((√3)du)/(3(1+u^2 ))) =(1/(√3)) arctan((t/(√3)))+c_2 changement t =tanθ give ∫ (dt/((t^2 +1)^2 )) = ∫ ((1+tan^2 θ)/((1+tan^2 θ)^2 ))dθ = ∫ (dθ/(1+tan^2 θ)) =∫ cos^2 θ dθ =∫ ((1+cos(2θ))/2)dθ =(θ/2) + (1/4) sin(2θ) =(1/2)arctant +(1/4) ((2t)/(1+t^2 )) +c_3 =(1/2) arctant +(t/(2(1+t^2 ))) +c_3 ⇒ I =−arctan(t)+c_1 +(1/(√3)) arctan((t/(√3))) +c_2 +((arctant)/2) + (t/(2(1+t^2 ))) +c_3 =−(1/2) arctan(t) +(1/(√3)) arctan((t/(√3))) +(t/(2(1+t^2 ))) +c .

$${let}\:{I}\:=\:\int\:\:\frac{{cos}^{\mathrm{2}} {x}\:−{sin}^{\mathrm{2}} {x}}{\mathrm{3}+{tan}^{\mathrm{2}} {x}}{dx}\:\:\Rightarrow\:{I}\:=\:\int\:\:\frac{{cos}\left(\mathrm{2}{x}\right)}{\mathrm{3}+{tan}^{\mathrm{2}} {x}}{dx}\:{changement}\:{tanx}\:={t}\:{give} \\ $$$${I}\:=\:\int\:\frac{\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }}{\mathrm{3}+{t}^{\mathrm{2}} }\:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }\:=\:\int\:\:\:\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} \left(\mathrm{3}+{t}^{\mathrm{2}} \right)}{dt}\:\:{let}\:{decokpose}\:{F}\left({t}\right)=\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} \left(\mathrm{3}+{t}^{\mathrm{2}} \right)} \\ $$$${F}\left({t}\right)\:=\frac{{at}\:+{b}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:\:+\frac{{ct}\:+{d}}{\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{{et}\:+{f}}{{t}^{\mathrm{2}} \:+\mathrm{3}} \\ $$$${F}\left(−{t}\right)\:={F}\left({t}\right)\:\Rightarrow\frac{−{at}\:+{b}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{−{ct}\:+{d}}{\left({t}^{\mathrm{2}\:} \:+\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{−{et}\:+{f}}{{t}^{\mathrm{2}} \:+\mathrm{3}}\:={F}\left({t}\right)\:\Rightarrow{a}=\mathrm{0}\:,{c}=\mathrm{0}\:{and}\:{e}=\mathrm{0}\:\Rightarrow \\ $$$${F}\left({t}\right)=\:\frac{{b}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{{d}}{\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{{f}}{{t}^{\mathrm{2}} \:+\mathrm{3}} \\ $$$${lim}_{{t}\rightarrow+\infty} {t}^{\mathrm{2}} {F}\left({t}\right)=\mathrm{0}\:={b}+{f}\:\Rightarrow{f}\:=−{b}\:\Rightarrow{F}\left({t}\right)=\frac{{b}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{{d}}{\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\:−\frac{{b}}{{t}^{\mathrm{2}} \:+\mathrm{3}} \\ $$$${F}\left({o}\right)\:=\frac{\mathrm{1}}{\mathrm{3}}\:=\:{b}\:+{d}−\frac{{b}}{\mathrm{3}}\:\Rightarrow\mathrm{1}=\mathrm{3}{b}+\mathrm{3}{d}−{b}\:=\mathrm{2}{b}\:+\mathrm{3}{d}\:\Rightarrow\mathrm{3}{d}=\mathrm{1}−\mathrm{2}{b}\:\Rightarrow{d}=\frac{\mathrm{1}−\mathrm{2}{b}}{\mathrm{3}} \\ $$$${F}\left({t}\right)\:=\:\frac{{b}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{\mathrm{1}−\mathrm{2}{b}}{\mathrm{3}\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\:−\frac{{b}}{{t}^{\mathrm{2}} \:+\mathrm{3}} \\ $$$${F}\left(\mathrm{1}\right)=\mathrm{0}\:=\frac{{b}}{\mathrm{2}}\:+\frac{\mathrm{1}−\mathrm{2}{b}}{\mathrm{12}}\:−\frac{{b}}{\mathrm{4}}\:\Rightarrow\mathrm{0}\:=\mathrm{6}{b}\:+\mathrm{1}−\mathrm{2}{b}−\mathrm{3}{b}\:\Rightarrow{b}+\mathrm{1}\:=\mathrm{0}\:\Rightarrow{b}=−\mathrm{1}\:\Rightarrow \\ $$$${F}\left({t}\right)\:=−\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\frac{\mathrm{1}}{\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\:+\frac{\mathrm{1}}{{t}^{\mathrm{2}} \:+\mathrm{3}}\:\Rightarrow \\ $$$${I}\:=−\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:+\:\int\:\:\frac{{dt}}{\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\:+\int\:\:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{3}}\:{but} \\ $$$$\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{1}}\:={arctant}\:+{c}_{\mathrm{1}} \\ $$$$\int\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{3}}\:=_{{t}=\sqrt{\mathrm{3}}{u}} \:\int\:\:\:\frac{\sqrt{\mathrm{3}}{du}}{\mathrm{3}\left(\mathrm{1}+{u}^{\mathrm{2}} \right)}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\:{arctan}\left(\frac{{t}}{\sqrt{\mathrm{3}}}\right)+{c}_{\mathrm{2}} \\ $$$${changement}\:{t}\:={tan}\theta\:{give} \\ $$$$\int\:\:\:\frac{{dt}}{\left({t}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }\:=\:\int\:\:\:\:\:\frac{\mathrm{1}+{tan}^{\mathrm{2}} \theta}{\left(\mathrm{1}+{tan}^{\mathrm{2}} \theta\right)^{\mathrm{2}} }{d}\theta\:=\:\int\:\:\frac{{d}\theta}{\mathrm{1}+{tan}^{\mathrm{2}} \theta}\:=\int\:{cos}^{\mathrm{2}} \theta\:{d}\theta \\ $$$$=\int\:\:\frac{\mathrm{1}+{cos}\left(\mathrm{2}\theta\right)}{\mathrm{2}}{d}\theta\:=\frac{\theta}{\mathrm{2}}\:+\:\frac{\mathrm{1}}{\mathrm{4}}\:{sin}\left(\mathrm{2}\theta\right)\:=\frac{\mathrm{1}}{\mathrm{2}}{arctant}\:+\frac{\mathrm{1}}{\mathrm{4}}\:\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{2}} }\:+{c}_{\mathrm{3}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:{arctant}\:+\frac{{t}}{\mathrm{2}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}\:+{c}_{\mathrm{3}} \:\Rightarrow \\ $$$${I}\:\:=−{arctan}\left({t}\right)+{c}_{\mathrm{1}} \:+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\:{arctan}\left(\frac{{t}}{\sqrt{\mathrm{3}}}\right)\:+{c}_{\mathrm{2}} \:+\frac{{arctant}}{\mathrm{2}}\:+\:\frac{{t}}{\mathrm{2}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}\:+{c}_{\mathrm{3}} \\ $$$$=−\frac{\mathrm{1}}{\mathrm{2}}\:{arctan}\left({t}\right)\:+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\:{arctan}\left(\frac{{t}}{\sqrt{\mathrm{3}}}\right)\:+\frac{{t}}{\mathrm{2}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}\:+{c}\:. \\ $$$$ \\ $$

Commented by maxmathsup by imad last updated on 18/Sep/18

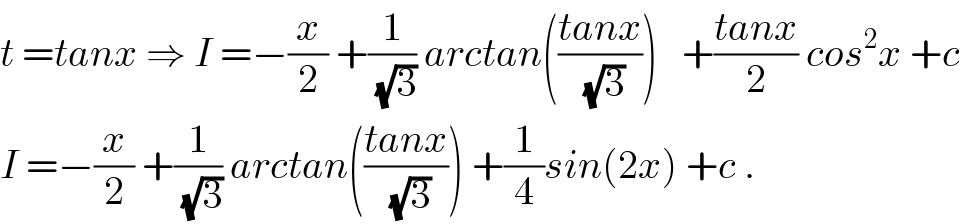
t =tanx ⇒ I =−(x/2) +(1/(√3)) arctan(((tanx)/(√3))) +((tanx)/2) cos^2 x +c I =−(x/2) +(1/(√3)) arctan(((tanx)/(√3))) +(1/4)sin(2x) +c .

$${t}\:={tanx}\:\Rightarrow\:{I}\:=−\frac{{x}}{\mathrm{2}}\:+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\:{arctan}\left(\frac{{tanx}}{\sqrt{\mathrm{3}}}\right)\:\:\:+\frac{{tanx}}{\mathrm{2}}\:{cos}^{\mathrm{2}} {x}\:+{c} \\ $$$${I}\:=−\frac{{x}}{\mathrm{2}}\:+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}\:{arctan}\left(\frac{{tanx}}{\sqrt{\mathrm{3}}}\right)\:+\frac{\mathrm{1}}{\mathrm{4}}{sin}\left(\mathrm{2}{x}\right)\:+{c}\:. \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 18/Sep/18

∫((cos2x)/(3+tan^2 x))dx t=tanx (dt/(1+t^2 ))=dx ∫((1−t^2 )/((1+t^2 )))×(1/(3+t^2 ))×(dt/(1+t^2 )) ∫((1−t^2 )/((1+t^2 )^2 (3+t^2 )))dt ∫((3+t^2 −2(1+t^2 ))/((1+t^2 )^2 (3+t^2 )))dt ∫(dt/((1+t^2 )^2 ))−∫((2dt)/((1+t^2 )(3+t^2 ))) ∫(dt/((1+t^2 )^2 ))−∫(((3+t^2 )−(1+t^2 ))/((1+t^2 )((3+t^2 )))dt ∫(dt/((1+t^2 )^2 ))−∫(dt/(1+t^2 ))+∫(dt/(3+t^2 )) I_1 −tan^(−1) (t)+(1/(√3))tan^(−1) ((t/(√3)))+c I_1 −tan^(−1) (tanx)+(1/(√3))tan^(−1) (((tanx)/(√3)))+c I_1 −x+(1/(√3))tan^(−1) (((tanx)/(√3)))+c now calculation of I_1 ∫(dt/((1+t^2 )^2 )) t=tanx dt=sec^2 xdx ∫((sec^2 xdx)/(sec^4 x)) ∫cos^2 xdx ∫(((1+cos2x)/2))dx (1/2)x+(1/2)×((sin2x)/2) so ans is ((x/2)+((sin2x)/4))−x+(1/(√3))tan^(−1) (((tanx)/(√3)))+c =((sin2x)/4)−(x/2)+(1/(√3))tan^(−1) (((tanx)/(√3)))+c

$$\int\frac{{cos}\mathrm{2}{x}}{\mathrm{3}+{tan}^{\mathrm{2}} {x}}{dx}\:\:\:\:\:\:{t}={tanx}\:\:\:\:\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }={dx} \\ $$$$\int\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}×\frac{\mathrm{1}}{\mathrm{3}+{t}^{\mathrm{2}} }×\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$$\int\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} \left(\mathrm{3}+{t}^{\mathrm{2}} \right)}{dt} \\ $$$$\int\frac{\mathrm{3}+{t}^{\mathrm{2}} −\mathrm{2}\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} \left(\mathrm{3}+{t}^{\mathrm{2}} \right)}{dt} \\ $$$$\int\frac{{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} }−\int\frac{\mathrm{2}{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\left(\mathrm{3}+{t}^{\mathrm{2}} \right)} \\ $$$$\int\frac{{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} }−\int\frac{\left(\mathrm{3}+{t}^{\mathrm{2}} \right)−\left(\mathrm{1}+{t}^{\mathrm{2}} \right)}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\left(\left(\mathrm{3}+{t}^{\mathrm{2}} \right)\right.}{dt} \\ $$$$\int\frac{{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} }−\int\frac{{dt}}{\mathrm{1}+{t}^{\mathrm{2}} }+\int\frac{{dt}}{\mathrm{3}+{t}^{\mathrm{2}} } \\ $$$${I}_{\mathrm{1}} −{tan}^{−\mathrm{1}} \left({t}\right)+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}{tan}^{−\mathrm{1}} \left(\frac{{t}}{\sqrt{\mathrm{3}}}\right)+{c} \\ $$$${I}_{\mathrm{1}} −{tan}^{−\mathrm{1}} \left({tanx}\right)+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}{tan}^{−\mathrm{1}} \left(\frac{{tanx}}{\sqrt{\mathrm{3}}}\right)+{c} \\ $$$${I}_{\mathrm{1}} −{x}+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}{tan}^{−\mathrm{1}} \left(\frac{{tanx}}{\sqrt{\mathrm{3}}}\right)+{c} \\ $$$${now}\:{calculation}\:{of}\:{I}_{\mathrm{1}} \\ $$$$\int\frac{{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)^{\mathrm{2}} }\:\:\:{t}={tanx}\:\:\:\:{dt}={sec}^{\mathrm{2}} {xdx} \\ $$$$\int\frac{{sec}^{\mathrm{2}} {xdx}}{{sec}^{\mathrm{4}} {x}} \\ $$$$\int{cos}^{\mathrm{2}} {xdx} \\ $$$$\int\left(\frac{\mathrm{1}+{cos}\mathrm{2}{x}}{\mathrm{2}}\right){dx} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}{x}+\frac{\mathrm{1}}{\mathrm{2}}×\frac{{sin}\mathrm{2}{x}}{\mathrm{2}} \\ $$$${so}\:{ans}\:{is} \\ $$$$\left(\frac{{x}}{\mathrm{2}}+\frac{{sin}\mathrm{2}{x}}{\mathrm{4}}\right)−{x}+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}{tan}^{−\mathrm{1}} \left(\frac{{tanx}}{\sqrt{\mathrm{3}}}\right)+{c} \\ $$$$=\frac{{sin}\mathrm{2}{x}}{\mathrm{4}}−\frac{{x}}{\mathrm{2}}+\frac{\mathrm{1}}{\sqrt{\mathrm{3}}}{tan}^{−\mathrm{1}} \left(\frac{{tanx}}{\sqrt{\mathrm{3}}}\right)+{c} \\ $$$$ \\ $$$$ \\ $$

Commented by maxmathsup by imad last updated on 18/Sep/18

your answer is correct sir Tanmay thanks.

$${your}\:{answer}\:{is}\:{correct}\:{sir}\:{Tanmay}\:{thanks}. \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 18/Sep/18

you are most welcome...

$${you}\:{are}\:{most}\:{welcome}... \\ $$

Commented by maxmathsup by imad last updated on 18/Sep/18

thank you sir..

$${thank}\:{you}\:{sir}.. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com