
Question and Answers Forum
Question Number 43999 by maxmathsup by imad last updated on 19/Sep/18
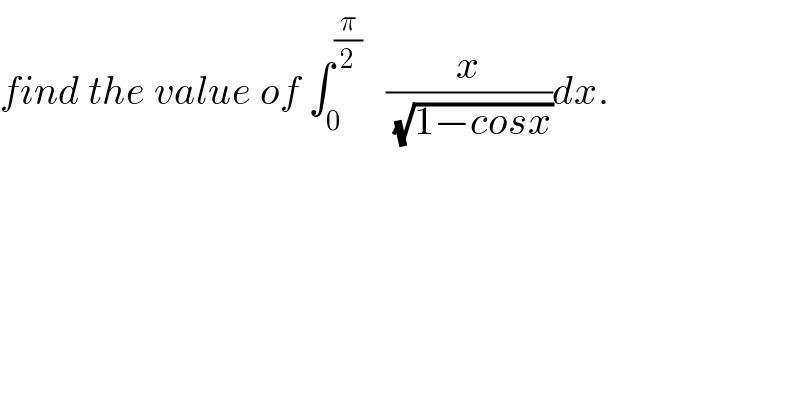
Commented by maxmathsup by imad last updated on 20/Sep/18
![let I = ∫_0 ^(π/2) (x/(√(1−cosx)))dx ⇒I =∫_0 ^(π/2) (x/(√(2sin^2 ((x/2)))))dx =(1/(√2)) ∫_0 ^(π/2) (x/(sin((x/2))))dx =_((x/2)=t) (1/(√2)) ∫_0 ^(π/4) ((2t)/(sin(t))) 2 dt =(4/(√2)) ∫_0 ^(π/4) (t/(sint))dt changement tan((t/2))=u give I = (4/(√2)) ∫_0 ^((√2)−1) ((2arctan(u))/((2u)/(1+u^2 ))) ((2du)/(1+u^2 )) =4(√( 2)) ∫_0 ^((√2)−1) ((arctan(u))/u) du let f(x)= ∫_0 ^((√2)−1) ((arctan(xu))/u) du ⇒ I =4(√2) f(1) we have f^′ (x) = ∫_0 ^((√2)−1) (du/(1+x^2 u^2 )) =_(xu =α) ∫_0 ^(x((√2)−1)) (1/(1+α^2 )) (dα/x) = (1/x) ∫_0 ^(x((√2)−1)) (dα/(1+α^2 )) =(1/x) [arctan(α)]_0 ^(x((√2)−1)) =((arctan{x((√2)−1)})/x) ⇒f(x) =∫_0 ^x ((arctan{t((√2)−1)})/t) dt +k k=f(0) =0 ⇒f(x) =∫_0 ^x ((arctan{t((√2)−1)})/t)dt ⇒ f(1) =∫_0 ^1 ((arctan{t((√2) −1)})/t)dtwe have arctan^′ (x) =(1/(1+x^2 )) =Σ_(n=0) ^∞ (−1)^n x^(2n) ⇒arctanx =Σ_(n=0) ^∞ (((−1)^n )/(2n+1)) x^(2n+1) ⇒ arctan{t((√2)−1)} =Σ_(n=0) ^∞ (((−1)^n )/(2n+1)) t^(2n+1) ((√2)−1)^(2n+1) ⇒ f(1) =Σ_(n=0) ^∞ ∫_0 ^1 (((−1)^n ((√2)−1)^(2n+1) )/(2n+1)) t^(2n) dt =Σ_(n=0) ^∞ (((−1)^n ((√2)−1)^(2n+1) )/((2n+1)^2 )) and I =4(√2)f(1)...](Q44033.png)
| ||
Question and Answers Forum | ||
Question Number 43999 by maxmathsup by imad last updated on 19/Sep/18 | ||
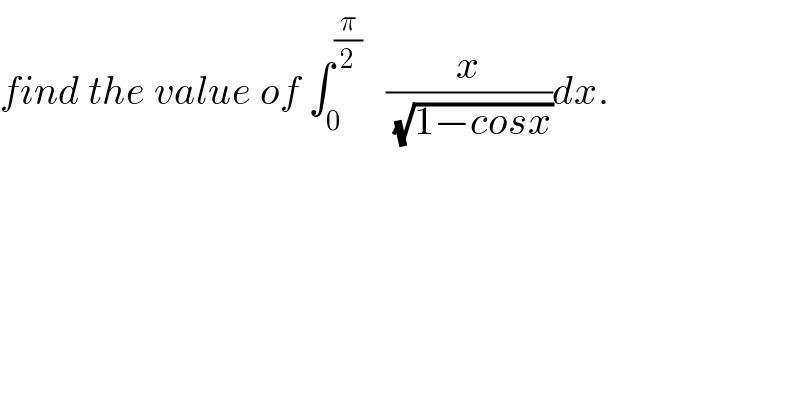 | ||
Commented by maxmathsup by imad last updated on 20/Sep/18 | ||
![let I = ∫_0 ^(π/2) (x/(√(1−cosx)))dx ⇒I =∫_0 ^(π/2) (x/(√(2sin^2 ((x/2)))))dx =(1/(√2)) ∫_0 ^(π/2) (x/(sin((x/2))))dx =_((x/2)=t) (1/(√2)) ∫_0 ^(π/4) ((2t)/(sin(t))) 2 dt =(4/(√2)) ∫_0 ^(π/4) (t/(sint))dt changement tan((t/2))=u give I = (4/(√2)) ∫_0 ^((√2)−1) ((2arctan(u))/((2u)/(1+u^2 ))) ((2du)/(1+u^2 )) =4(√( 2)) ∫_0 ^((√2)−1) ((arctan(u))/u) du let f(x)= ∫_0 ^((√2)−1) ((arctan(xu))/u) du ⇒ I =4(√2) f(1) we have f^′ (x) = ∫_0 ^((√2)−1) (du/(1+x^2 u^2 )) =_(xu =α) ∫_0 ^(x((√2)−1)) (1/(1+α^2 )) (dα/x) = (1/x) ∫_0 ^(x((√2)−1)) (dα/(1+α^2 )) =(1/x) [arctan(α)]_0 ^(x((√2)−1)) =((arctan{x((√2)−1)})/x) ⇒f(x) =∫_0 ^x ((arctan{t((√2)−1)})/t) dt +k k=f(0) =0 ⇒f(x) =∫_0 ^x ((arctan{t((√2)−1)})/t)dt ⇒ f(1) =∫_0 ^1 ((arctan{t((√2) −1)})/t)dtwe have arctan^′ (x) =(1/(1+x^2 )) =Σ_(n=0) ^∞ (−1)^n x^(2n) ⇒arctanx =Σ_(n=0) ^∞ (((−1)^n )/(2n+1)) x^(2n+1) ⇒ arctan{t((√2)−1)} =Σ_(n=0) ^∞ (((−1)^n )/(2n+1)) t^(2n+1) ((√2)−1)^(2n+1) ⇒ f(1) =Σ_(n=0) ^∞ ∫_0 ^1 (((−1)^n ((√2)−1)^(2n+1) )/(2n+1)) t^(2n) dt =Σ_(n=0) ^∞ (((−1)^n ((√2)−1)^(2n+1) )/((2n+1)^2 )) and I =4(√2)f(1)...](Q44033.png) | ||