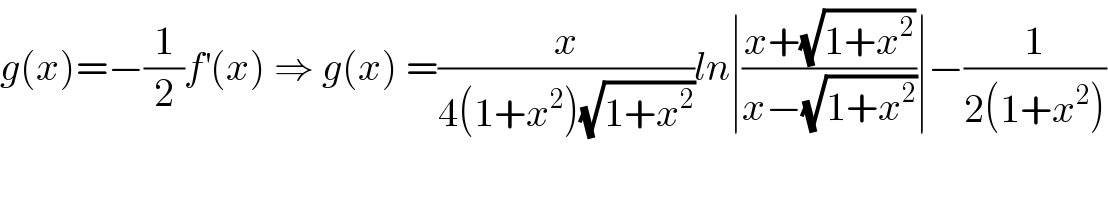Question and Answers Forum
Question Number 44476 by abdo.msup.com last updated on 29/Sep/18
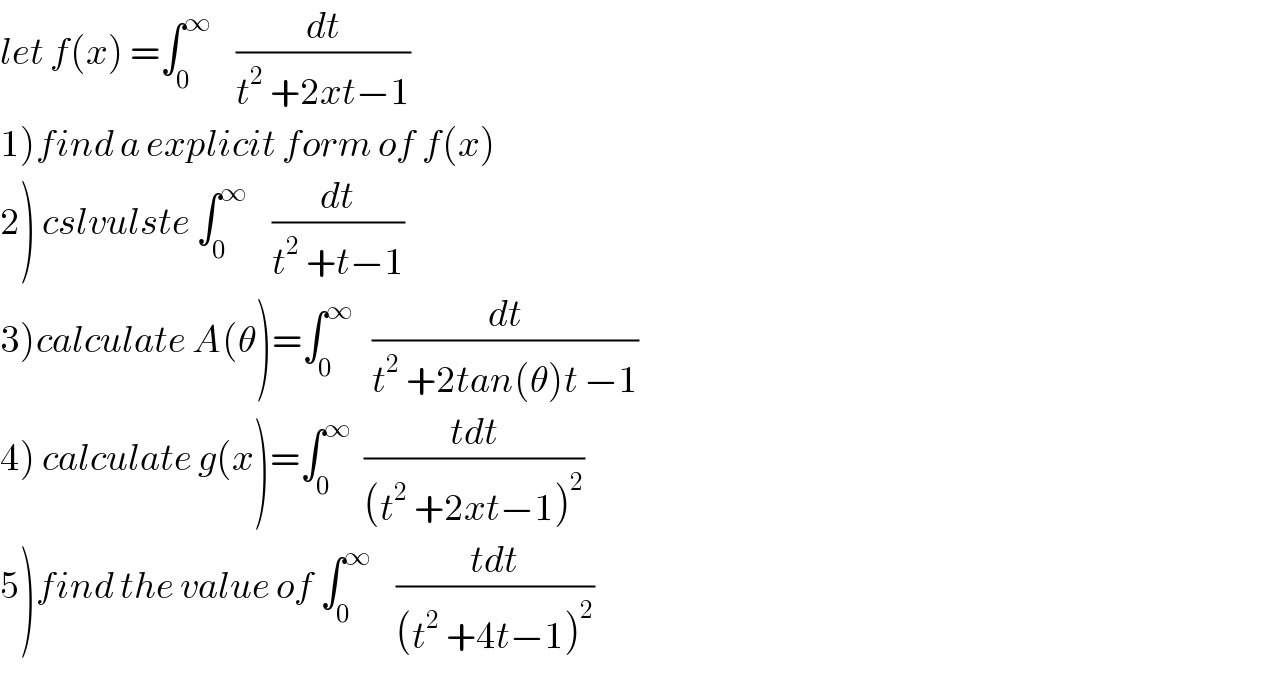
Commented by maxmathsup by imad last updated on 01/Oct/18
![1) we have f(x) =∫_0 ^∞ (dt/(t^2 +2xt+x^2 −x^2 −1)) = ∫_0 ^∞ (dt/((t+x)^2 −(x^2 +1))) =∫_0 ^∞ (dt/((t+x+(√(1+x^2 )))(t+x−(√(1+x^2 ))))) =(1/(2(√(1+x^2 )))) ∫_0 ^∞ ((1/(t+x−(√(1+x^2 )))) −(1/(t+x +(√(1+x^2 )))))dt =(1/(2(√(1+x^2 ))))[ln∣((t+x−(√(1+x^2 )))/(t+x+(√(1+x^2 ))))]_0 ^(+∞) =(1/(2(√(1+x^2 ))))(−ln∣((x−(√(1+x^2 )))/(x+(√(1+x^2 ))))∣) ⇒f(x)=(1/(2(√(1+x^2 ))))ln∣((x+(√(1+x^2 )))/(x−(√(1+x^2 ))))∣ .](Q44588.png)
Commented by maxmathsup by imad last updated on 01/Oct/18

Commented by maxmathsup by imad last updated on 01/Oct/18
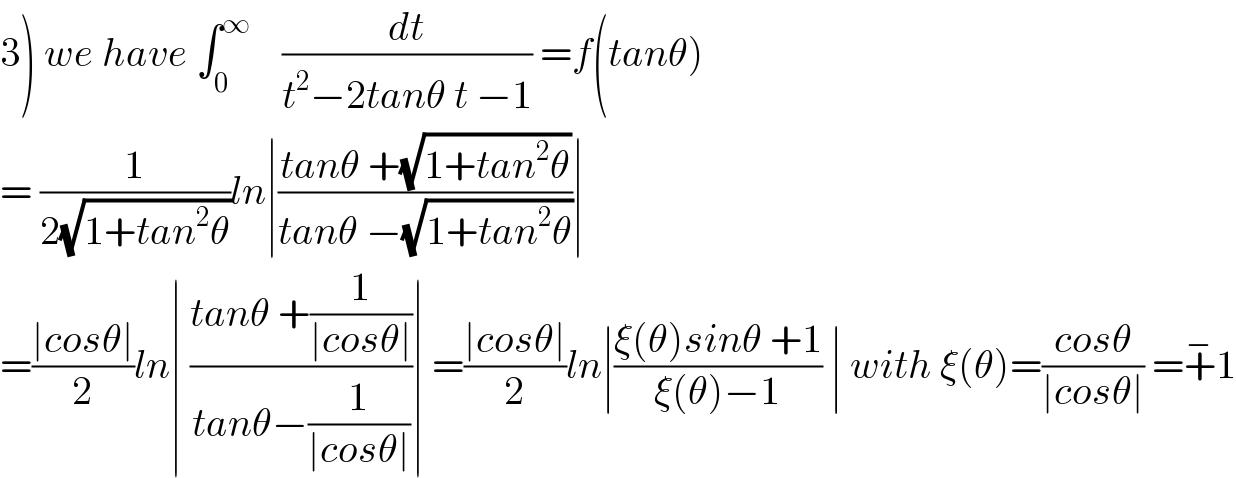
Commented by maxmathsup by imad last updated on 01/Oct/18
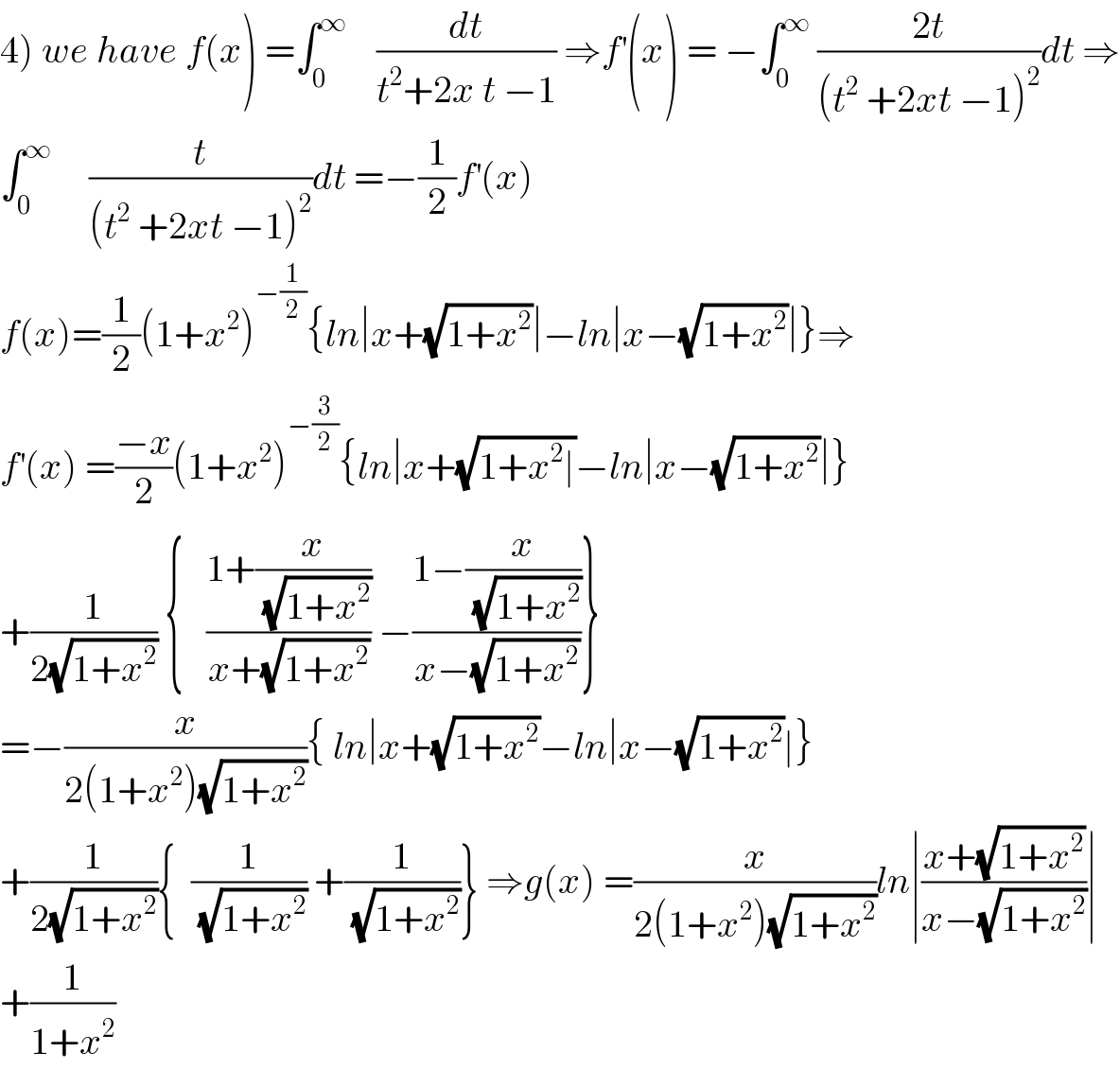
Commented by maxmathsup by imad last updated on 01/Oct/18

Commented by maxmathsup by imad last updated on 01/Oct/18