Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 44512 by arvinddayama01@gmail.com last updated on 30/Sep/18

prove that:−∫2^(ln x) dx = ((x.2^(ln x) )/(ln(xe))) +C

$$\boldsymbol{{prove}}\:\boldsymbol{{that}}:−\int\mathrm{2}^{\boldsymbol{\mathrm{ln}}\:\boldsymbol{\mathrm{x}}} \:\boldsymbol{\mathrm{dx}}\:=\:\frac{\boldsymbol{\mathrm{x}}.\mathrm{2}^{\boldsymbol{\mathrm{ln}}\:\boldsymbol{\mathrm{x}}} }{\boldsymbol{\mathrm{ln}}\left(\boldsymbol{\mathrm{xe}}\right)}\:+\boldsymbol{\mathrm{C}} \\ $$$$ \\ $$

Commented by maxmathsup by imad last updated on 30/Sep/18

let I = ∫ 2^(ln(x)) dx cha7gement ln(x)=t give I = ∫ 2^t e^t dt =∫ e^(tln(2) +t) dt = ∫ e^((1+ln(2))t) dt = (1/(1+ln(2))) e^((1+ln(2))t) +c =(1/(1+ln(2))) e^((1+ln(2))ln(x)) +c =(1/(1+ln(2))) x .2^(ln(x)) +c so there is aerror at the question...!

$${let}\:{I}\:=\:\int\:\:\mathrm{2}^{{ln}\left({x}\right)} {dx}\:{cha}\mathrm{7}{gement}\:{ln}\left({x}\right)={t}\:{give} \\ $$$${I}\:\:=\:\int\:\:\mathrm{2}^{{t}} \:\:{e}^{{t}} \:{dt}\:\:\:=\int\:\:{e}^{{tln}\left(\mathrm{2}\right)\:+{t}} \:{dt}\:=\:\int\:\:{e}^{\left(\mathrm{1}+{ln}\left(\mathrm{2}\right)\right){t}} {dt} \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{1}+{ln}\left(\mathrm{2}\right)}\:{e}^{\left(\mathrm{1}+{ln}\left(\mathrm{2}\right)\right){t}} \:+{c}\:=\frac{\mathrm{1}}{\mathrm{1}+{ln}\left(\mathrm{2}\right)}\:{e}^{\left(\mathrm{1}+{ln}\left(\mathrm{2}\right)\right){ln}\left({x}\right)} \:+{c} \\ $$$$=\frac{\mathrm{1}}{\mathrm{1}+{ln}\left(\mathrm{2}\right)}\:{x}\:\:.\mathrm{2}^{{ln}\left({x}\right)} \:+{c}\:\:\:\:{so}\:{there}\:{is}\:{aerror}\:{at}\:{the}\:{question}...! \\ $$$$ \\ $$

Answered by MJS last updated on 01/Oct/18

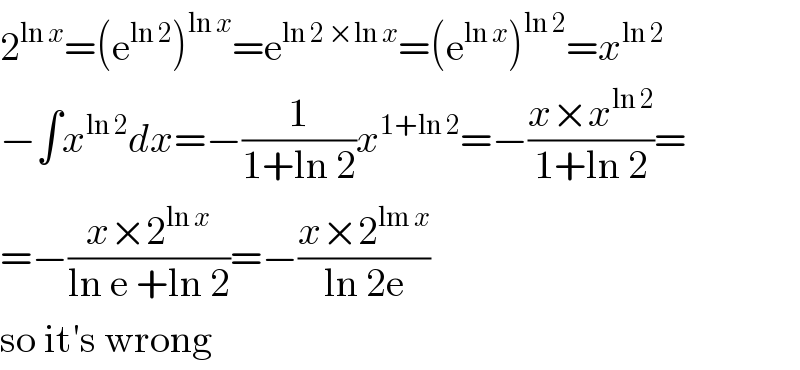
2^(ln x) =(e^(ln 2) )^(ln x) =e^(ln 2 ×ln x) =(e^(ln x) )^(ln 2) =x^(ln 2) −∫x^(ln 2) dx=−(1/(1+ln 2))x^(1+ln 2) =−((x×x^(ln 2) )/(1+ln 2))= =−((x×2^(ln x) )/(ln e +ln 2))=−((x×2^(lm x) )/(ln 2e)) so it′s wrong

$$\mathrm{2}^{\mathrm{ln}\:{x}} =\left(\mathrm{e}^{\mathrm{ln}\:\mathrm{2}} \right)^{\mathrm{ln}\:{x}} =\mathrm{e}^{\mathrm{ln}\:\mathrm{2}\:×\mathrm{ln}\:{x}} =\left(\mathrm{e}^{\mathrm{ln}\:{x}} \right)^{\mathrm{ln}\:\mathrm{2}} ={x}^{\mathrm{ln}\:\mathrm{2}} \\ $$$$−\int{x}^{\mathrm{ln}\:\mathrm{2}} {dx}=−\frac{\mathrm{1}}{\mathrm{1}+\mathrm{ln}\:\mathrm{2}}{x}^{\mathrm{1}+\mathrm{ln}\:\mathrm{2}} =−\frac{{x}×{x}^{\mathrm{ln}\:\mathrm{2}} }{\mathrm{1}+\mathrm{ln}\:\mathrm{2}}= \\ $$$$=−\frac{{x}×\mathrm{2}^{\mathrm{ln}\:{x}} }{\mathrm{ln}\:\mathrm{e}\:+\mathrm{ln}\:\mathrm{2}}=−\frac{{x}×\mathrm{2}^{\mathrm{lm}\:{x}} }{\mathrm{ln}\:\mathrm{2e}} \\ $$$$\mathrm{so}\:\mathrm{it}'\mathrm{s}\:\mathrm{wrong} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com