Question and Answers Forum
Question Number 44676 by ajfour last updated on 03/Oct/18

Commented by ajfour last updated on 03/Oct/18
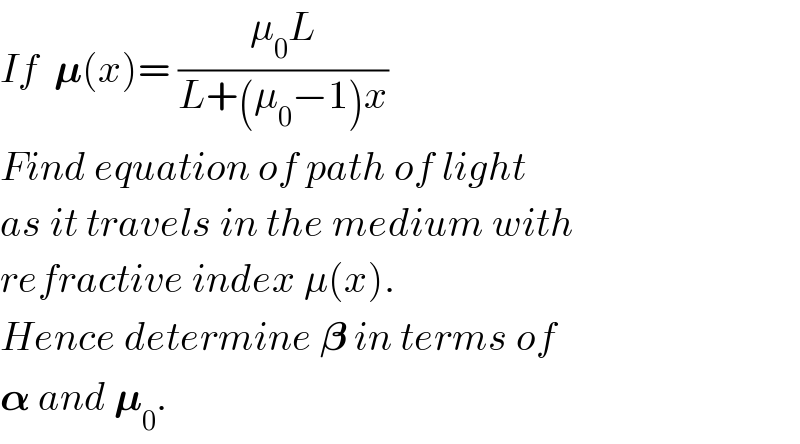
Commented by MrW3 last updated on 03/Oct/18
![𝛍(x)= ((μ_0 L)/(L+(μ_0 −1)x)) (dμ/dx)=−(((μ_0 −1)μ_0 L)/([L+(μ_0 −1)x]^2 ))=−(((μ_0 −1)μ)/(L+(μ_0 −1)x)) μ sin θ=constant sin θ (dμ/dx)+μ cos θ (dθ/dx)=0 −(((μ_0 −1)μ)/(L+(μ_0 −1)x))+μ((cos θ)/(sin θ))×(dθ/dx)=0 −(((μ_0 −1))/(L+(μ_0 −1)x))+((d(ln sin θ))/dx)=0 let Φ=ln sin θ −(((μ_0 −1))/(L+(μ_0 −1)x))+(dΦ/dx)=0 dΦ=(((μ_0 −1)dx)/(L+(μ_0 −1)x)) ∫_Φ_0 ^Φ dΦ=∫_0 ^x (((μ_0 −1)dx)/(L+(μ_0 −1)x)) Φ−Φ_0 =[ln {L+(μ_0 −1)x}]_0 ^x ln ((sin θ)/(sin θ_0 ))=ln ((L+(μ_0 −1)x)/L) sin θ=sin θ_0 ((L+(μ_0 −1)x)/L) at x=L: sin θ_1 =μ_0 sin θ_0 ⇒θ_1 =sin^(−1) (μ_0 sin θ_0 ) sin θ=sin θ_0 ((L+(μ_0 −1)x)/L)=sin θ_0 [1+(μ_0 −1)(x/L)]=λ(x) λ(x)=sin θ_0 [1+(μ_0 −1)(x/L)] dλ=sin θ_0 ((μ_0 −1)/L) dx tan θ=((sin θ)/(√(1−sin^2 θ)))=(λ/(√(1−λ^2 ))) (dy/dx)=(λ/(√(1−λ^2 ))) dy=(λ/(√(1−λ^2 )))dx=(λ/(√(1−λ^2 )))×(L/((μ_0 −1)sin θ_0 ))×dλ (((μ_0 −1)sin θ_0 )/L)dy=(λ/(√(1−λ^2 )))dλ (((μ_0 −1)sin θ_0 )/L)∫_0 ^y dy=∫_λ_0 ^λ (λ/(√(1−λ^2 )))dλ (((μ_0 −1)sin θ_0 )/L)y=−(1/2)∫_λ_0 ^λ (1/(√(1−λ^2 )))d(1−λ^2 ) (((μ_0 −1)sin θ_0 )/L)y=−[(√(1−λ^2 ))]_λ_0 ^λ =(√(1−λ_0 ^2 ))−(√(1−λ^2 )) λ_0 =sin θ_0 (((μ_0 −1)sin θ_0 )/L)y=cos θ_0 −cos θ=cos θ_0 −(√(1−sin^2 θ_0 [1+(μ_0 −1)(x/L)]^2 )) ⇒y=(L/((μ_0 −1)sin θ_0 )){cos θ_0 −(√(1−sin^2 θ_0 [1+(μ_0 −1)(x/L)]^2 ))} at x=0: μ(0)=μ_0 from air into medium sin α=μ_0 sin θ_0 ⇒sin θ_0 =((sin α)/μ_0 ) at x=L: μ(1)=1 from medium into air μ(1)×sin θ_1 =sin β ⇒β=θ_1 =sin^(−1) (μ_0 sin θ_0 )=sin^(−1) (sin α)=α ⇒y=((μ_0 L)/((μ_0 −1)sin α)){(√(1−(((sin α)/μ_0 ))^2 ))−(√(1−(((sin α)/μ_0 ))^2 [1+(μ_0 −1)(x/L)]^2 ))}](Q44682.png)
Commented by ajfour last updated on 03/Oct/18

Commented by MrW3 last updated on 03/Oct/18

Commented by ajfour last updated on 03/Oct/18
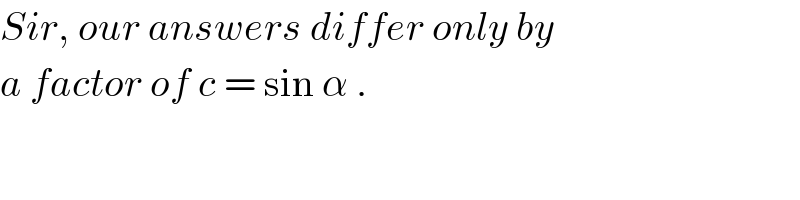
Commented by MrW3 last updated on 03/Oct/18
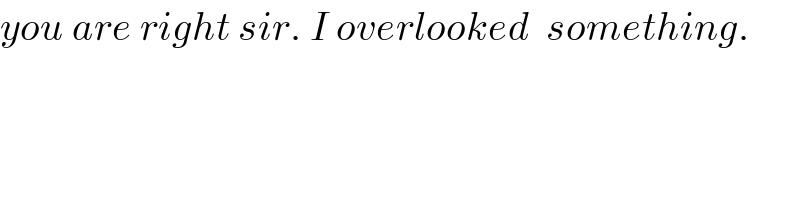
Answered by ajfour last updated on 03/Oct/18
![sin α = μ_0 sin θ_0 = sin β = μsin θ =c ((dy/dx))^2 = tan^2 θ = ((sin^2 θ)/(1−sin^2 θ)) (dy/dx) = ((c/μ)/(√(1−(c^2 /μ^2 )))) = (c/(√(μ^2 −c^2 ))) y = ∫_μ_0 ^( μ) (c/(√(μ^2 −c^2 )))×((dx/dμ))dμ 𝛍= ((𝛍_0 L)/(L+(𝛍_0 −1)x)) (μ_0 −1)x = ((μ_0 L)/μ) − L ⇒ (dx/dμ) = −((μ_0 L)/(μ^2 (μ_0 −1))) y = −∫_μ_0 ^( μ) (c/(√(μ^2 −c^2 )))(((μ_0 L)/(μ^2 (μ_0 −1))))dμ = ((μ_0 cL)/(μ_0 −1))∫_μ ^( μ_0 ) (dμ/(μ^2 (√(μ^2 −c^2 )))) let μ= csec φ ⇒ dμ = csec φtan φdφ y = ((μ_0 cL)/(μ_0 −1))∫_φ ^( φ_0 ) ((csec φtan φdφ)/(c^2 sec^2 φ(ctan φ))) = ((μ_0 L)/(c(μ_0 −1))) [sin φ_0 −sin φ] y = ((𝛍_0 L)/(c(𝛍_0 −1)))[((√(𝛍_0 ^2 −c^2 ))/𝛍_0 )−((√(𝛍^2 −c^2 ))/𝛍) ] with 𝛍 = ((𝛍_0 L)/(L+(𝛍_0 −1)x)) & c=sin 𝛂 .](Q44701.png)
Commented by MrW3 last updated on 03/Oct/18