
Question and Answers Forum
Question Number 45044 by maxmathsup by imad last updated on 07/Oct/18
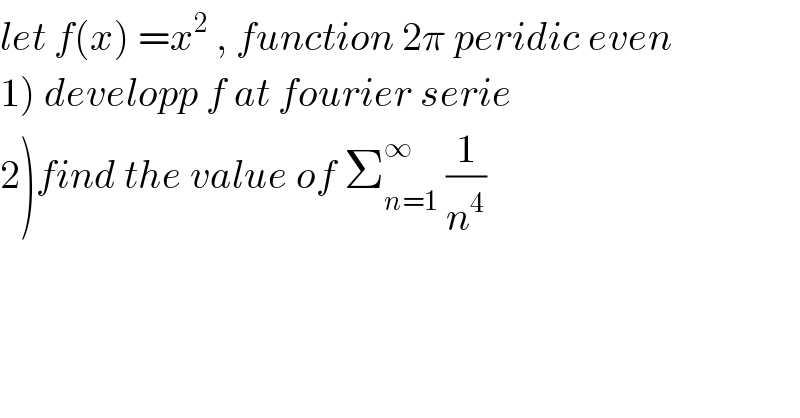
Commented by maxmathsup by imad last updated on 08/Oct/18
![f is even ⇒f(x)=(a_0 /2) +Σ_(n=1) ^∞ a_n cos(nx) with a_n =(2/T)∫_([T]) f(x)cos(nx) =(2/(2π)) ∫_(−π) ^π x^2 cos(nx)dx =(2/π) ∫_0 ^π x^2 cos(nx) ⇒(π/2)a_n = ∫_0 ^π x^2 cos(nx) by psrts we get ∫_0 ^π x^2 cos(nx)dx =[(x^2 /n)sin(nx)]_0 ^π −∫_0 ^π ((2x)/n)sin(nx)dx =−(2/n) ∫_0 ^π x sin(nx)dx =−(2/n){ [−(x/n)cos(nx)]_0 ^π −∫_0 ^π −(1/n)cos(nx)dx} =(2/n^2 ) ( π(−1)^n ) −(2/n^2 )∫_0 ^π cos(nx)dx =((2π(−1)^n )/n^2 ) ⇒(π/2)a_n =((2π(−1)^n )/n^2 ) ⇒ a_n = ((4(−1)^n )/n^2 ) also a_0 =(2/π) ∫_0 ^π x^2 dx =(2/π) (π^3 /3) =((2π^2 )/3) ⇒(a_0 /2) =(π^2 /3) ⇒ ★x^2 =(π^2 /3) +4 Σ_(n=1) ^∞ (((−1)^n )/n^2 ) cos(nx)★ 2) the parseval formulae give (1/T)∫_([T]) f^2 (x)dx =((a_o /2))^2 +(1/2)Σ_(n≥1) (a_n ^2 +b_n ^2 ) ⇒ (1/(2π)) ∫_(−π) ^π x^4 dx =(π^4 /9) +8 Σ_(n=1) ^∞ (1/n^4 ) ⇒(1/π) ∫_0 ^π x^4 dx =(π^4 /9) +8 Σ_(n=1) ^∞ (1/n^4 ) ⇒ (π^4 /5) −(π^4 /9) =8Σ_(n=1) ^∞ (1/n^4 ) ⇒ Σ_(n=1) ^∞ (1/n^4 ) =(1/8)(((4π^4 )/(45))) = (π^4 /(90)) ★ Σ_(n=1) ^∞ (1/n^4 ) =(π^4 /(90)) ★](Q45073.png)
| ||
Question and Answers Forum | ||
Question Number 45044 by maxmathsup by imad last updated on 07/Oct/18 | ||
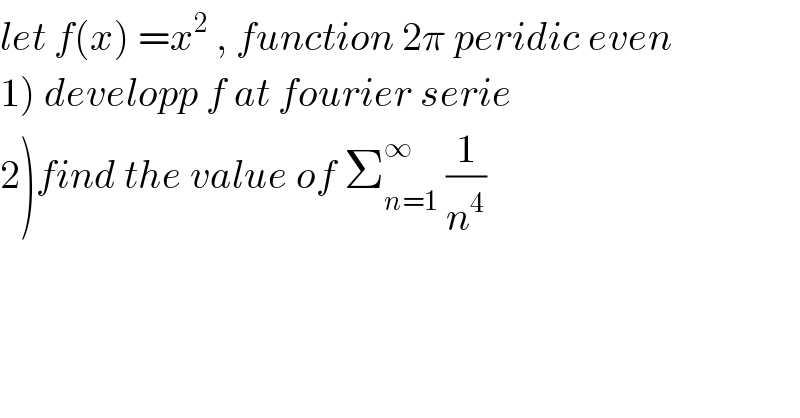 | ||
Commented by maxmathsup by imad last updated on 08/Oct/18 | ||
![f is even ⇒f(x)=(a_0 /2) +Σ_(n=1) ^∞ a_n cos(nx) with a_n =(2/T)∫_([T]) f(x)cos(nx) =(2/(2π)) ∫_(−π) ^π x^2 cos(nx)dx =(2/π) ∫_0 ^π x^2 cos(nx) ⇒(π/2)a_n = ∫_0 ^π x^2 cos(nx) by psrts we get ∫_0 ^π x^2 cos(nx)dx =[(x^2 /n)sin(nx)]_0 ^π −∫_0 ^π ((2x)/n)sin(nx)dx =−(2/n) ∫_0 ^π x sin(nx)dx =−(2/n){ [−(x/n)cos(nx)]_0 ^π −∫_0 ^π −(1/n)cos(nx)dx} =(2/n^2 ) ( π(−1)^n ) −(2/n^2 )∫_0 ^π cos(nx)dx =((2π(−1)^n )/n^2 ) ⇒(π/2)a_n =((2π(−1)^n )/n^2 ) ⇒ a_n = ((4(−1)^n )/n^2 ) also a_0 =(2/π) ∫_0 ^π x^2 dx =(2/π) (π^3 /3) =((2π^2 )/3) ⇒(a_0 /2) =(π^2 /3) ⇒ ★x^2 =(π^2 /3) +4 Σ_(n=1) ^∞ (((−1)^n )/n^2 ) cos(nx)★ 2) the parseval formulae give (1/T)∫_([T]) f^2 (x)dx =((a_o /2))^2 +(1/2)Σ_(n≥1) (a_n ^2 +b_n ^2 ) ⇒ (1/(2π)) ∫_(−π) ^π x^4 dx =(π^4 /9) +8 Σ_(n=1) ^∞ (1/n^4 ) ⇒(1/π) ∫_0 ^π x^4 dx =(π^4 /9) +8 Σ_(n=1) ^∞ (1/n^4 ) ⇒ (π^4 /5) −(π^4 /9) =8Σ_(n=1) ^∞ (1/n^4 ) ⇒ Σ_(n=1) ^∞ (1/n^4 ) =(1/8)(((4π^4 )/(45))) = (π^4 /(90)) ★ Σ_(n=1) ^∞ (1/n^4 ) =(π^4 /(90)) ★](Q45073.png) | ||