Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 45498 by Sanjarbek last updated on 13/Oct/18

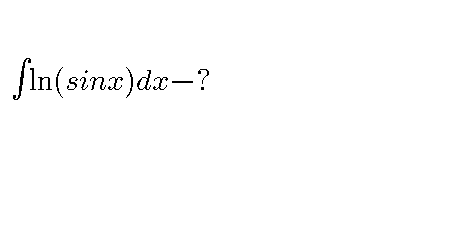
Commented by Meritguide1234 last updated on 13/Oct/18

$$notsolvable$$

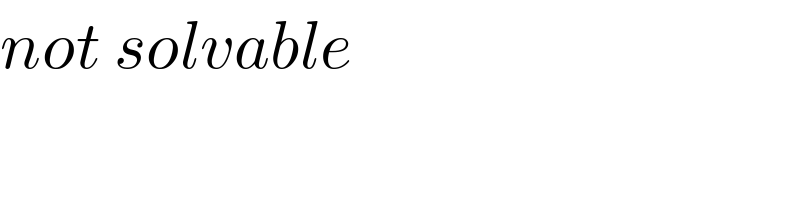
Commented by MJS last updated on 13/Oct/18

$$\mathrm{possible}\mathrm{but}\mathrm{not}\mathrm{elementary}.{\mathrm{I}}^{\prime }\mathrm{ll}\mathrm{post}\mathrm{it}\mathrm{later}$$

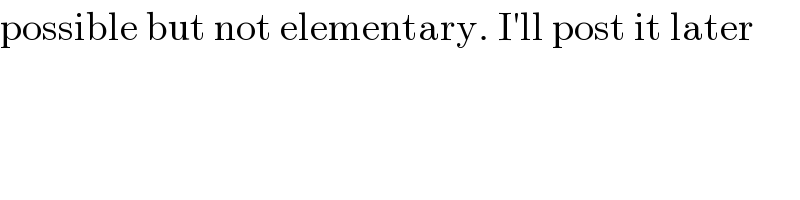
Commented by maxmathsup by imad last updated on 13/Oct/18

$$letf\left(t\right)=\int ln\left(sin\left(tx\right)\right)dx\Rightarrow f^{{}^{\prime }}\left(t\right)=\int \frac{xcos\left(tx\right)}{sin\left(tx\right)}dx$$$${=}_{tx=u}\int \frac{u}{t}\frac{cos\left(u\right)}{sin\left(u\right)}\frac{du}{t}=\frac{1}{t^2}\int u\frac{cos\left(u\right)}{sin\left(u\right)}dualsochangementtan\left(\frac{u}{2}\right)=\alpha$$$$givef\left(t\right)=\frac{1}{t^2}\int 2arctan\left(\alpha \right)\frac{\frac{1-{\alpha }^2}{1+{\alpha }^2}}{\frac{2\alpha }{1+{\alpha }^2}}\frac{2d\alpha }{1+{\alpha }^2}$$$$=\frac{4}{t^2}\int \frac{arctan\left(\alpha \right)}{1+{\alpha }^2}d\alpha letthenintroducetheparametricfunction$$$$\phi \left(u\right)=\int \frac{arctan\left(u\alpha \right)}{1+{\alpha }^2}d\alpha \Rightarrow {\phi }^{{}^{\prime }}\left(u\right)=\int \frac{\alpha }{(1+{\alpha }^2)(1+u^2{\alpha }^2)}d\alpha letdecompose$$$$F\left(\alpha \right)=\frac{a\alpha +b}{1+{\alpha }^2}+\frac{c\alpha +d}{1+u^2{\alpha }^2}....becontinued...$$$$$$$$$$

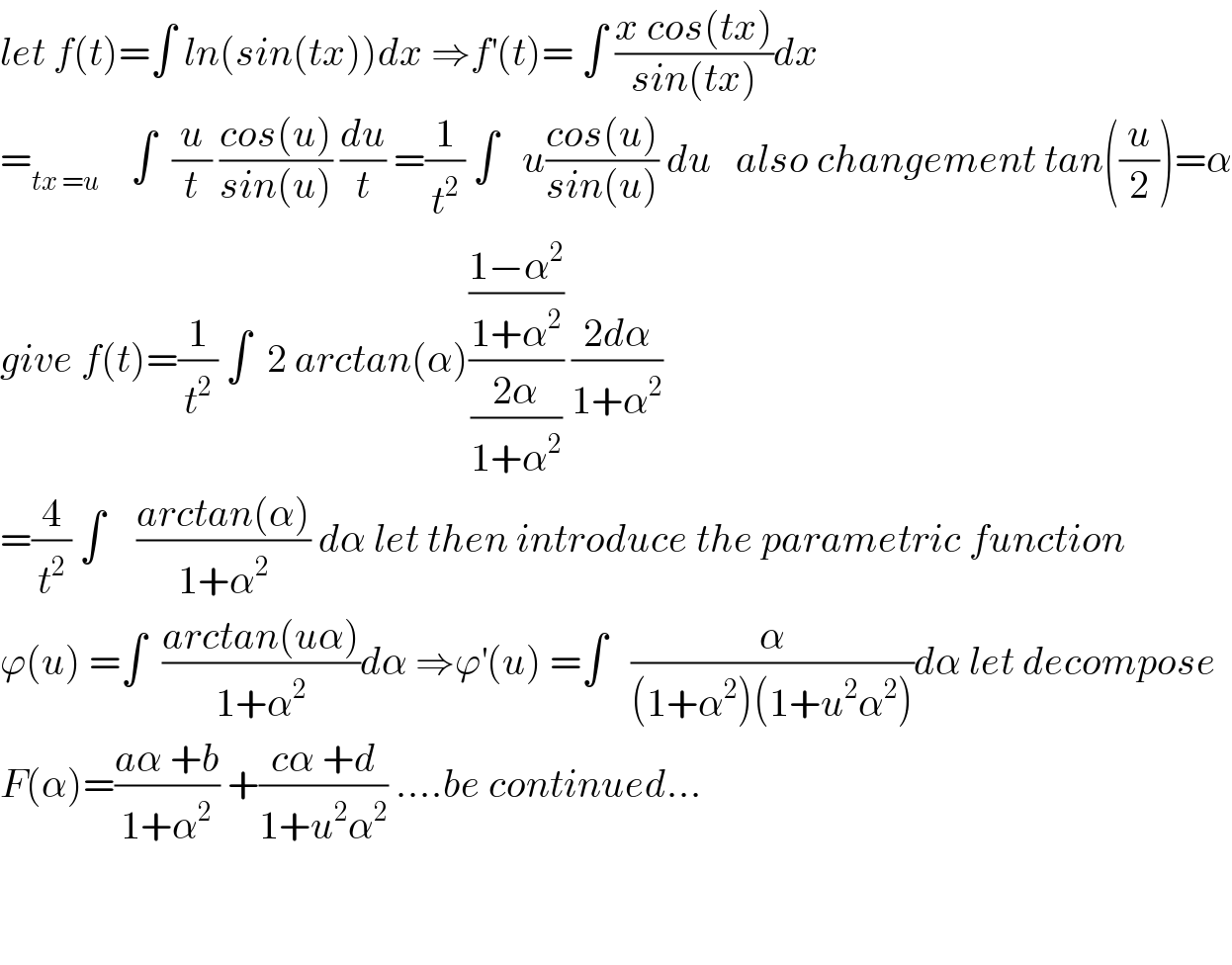
Answered by MJS last updated on 14/Oct/18

$$\int \ln \sin xdx=\int \ln (-\frac{\mathrm{i}}{2}({\mathrm{e}}^{\mathrm{i}x}-{\mathrm{e}}^{-\mathrm{i}x}))dx=$$$$[t=\mathrm{i}x\to dx=-\mathrm{i}dt$$$$=-\mathrm{i}\int \ln (-\frac{\mathrm{i}}{2}({\mathrm{e}}^t-{\mathrm{e}}^{-t}))dt=$$$$=-\mathrm{i}\int \ln (-\frac{\mathrm{i}}{2})dt-\mathrm{i}\int \ln ({\mathrm{e}}^t-{\mathrm{e}}^{-t})dt$$$$\mathrm{the}\mathrm{first}\mathrm{one}\mathrm{is}\mathrm{easy}:$$$$-\mathrm{i}\int \ln (-\frac{\mathrm{i}}{2})dt=-\mathrm{i}\ln (-\frac{\mathrm{i}}{2})\int dt=-\mathrm{i}\ln (-\frac{\mathrm{i}}{2})t=$$$$=\ln (-\frac{\mathrm{i}}{2})x$$$$\mathrm{the}\mathrm{second}\mathrm{one}:$$$$-\mathrm{i}\int \ln ({\mathrm{e}}^t-{\mathrm{e}}^{-t})dt=$$$$\left[\begin{array}{c}\int f^{\prime }g=fg-\int {fg}^{\prime }\\ f^{\prime }=1\to f=t\\ g=\ln ({\mathrm{e}}^t-{\mathrm{e}}^{-t})\to g^{\prime }=\frac{{\mathrm{e}}^t+{\mathrm{e}}^{-t}}{{\mathrm{e}}^t-{\mathrm{e}}^{-t}}\end{array}\right]$$$$=-\mathrm{i}t\ln ({\mathrm{e}}^t-{\mathrm{e}}^{-t})+\mathrm{i}\int t\frac{{\mathrm{e}}^t+{\mathrm{e}}^{-t}}{{\mathrm{e}}^t-{\mathrm{e}}^{-t}}dt$$$$\mathrm{the}\mathrm{first}\mathrm{term}:$$$$-\mathrm{i}t\ln ({\mathrm{e}}^t-{\mathrm{e}}^{-t})=x\ln ({\mathrm{e}}^{\mathrm{i}x}-{\mathrm{e}}^{-\mathrm{i}x})$$$$\mathrm{the}\mathrm{second}\mathrm{term}:$$$$\mathrm{i}\int t\frac{{\mathrm{e}}^t+{\mathrm{e}}^{-t}}{{\mathrm{e}}^t-{\mathrm{e}}^{-t}}dt=\mathrm{i}\int t\frac{{\mathrm{e}}^{2t}+1}{{\mathrm{e}}^{2t}-1}dt=$$$$[u={\mathrm{e}}^{2t}-1\to dt=\frac{{\mathrm{e}}^{-2t}}{2}du]$$$$=\frac{\mathrm{i}}{4}\int \frac{(u+2)\ln (u+1)}{u(u+1)}du=$$$$=\frac{\mathrm{i}}{2}\int \frac{\ln (u+1)}{u}du+\frac{\mathrm{i}}{4}\int \frac{\ln (u+1)}{u+1}du$$$$\mathrm{the}\mathrm{first}\mathrm{one}:$$$$\frac{\mathrm{i}}{2}\int \frac{\ln (u+1)}{u}du=$$$$[v=-u\to du=-dv]$$$$=\frac{\mathrm{i}}{2}\int \frac{\ln (1-v)}{v}dv=-\frac{\mathrm{i}}{2}\int -\frac{\ln (1-v)}{v}dv=$$$$\mathrm{this}\mathrm{is}\mathrm{a}\mathrm{special}\mathrm{integral}\left(\mathrm{dilogarithm}\right)$$$$=-\frac{\mathrm{i}}{2}{\mathrm{Li}}_{2}v=-\frac{\mathrm{i}}{2}{\mathrm{Li}}_2(-u)=-\frac{\mathrm{i}}{2}{\mathrm{Li}}_2(1-{\mathrm{e}}^{2t})=$$$$=-\frac{\mathrm{i}}{2}{\mathrm{Li}}_2(1-{\mathrm{e}}^{2\mathrm{i}x})$$$$\mathrm{the}\mathrm{second}\mathrm{one}:$$$$\frac{\mathrm{i}}{4}\int \frac{\ln (u+1)}{u+1}du=$$$$[w=\ln (u+1)\to du=(u+1)dw]$$$$=\frac{\mathrm{i}}{4}\int wdw=\frac{\mathrm{i}}{8}{w}^2=\frac{\mathrm{i}}{8}{\ln }^2(u+1)=\frac{\mathrm{i}}{8}{\ln }^2\left({\mathrm{e}}^{2t}\right)=$$$$=\frac{\mathrm{i}}{2}{t}^2=-\frac{\mathrm{i}}{2}{x}^2$$$$\mathrm{so}\mathrm{we}\mathrm{have}$$$$\int \ln \sin xdx=$$$$=x\ln (-\frac{\mathrm{i}}{2})+x\ln ({\mathrm{e}}^{\mathrm{i}x}-{\mathrm{e}}^{-\mathrm{i}x})-\frac{\mathrm{i}}{2}{\mathrm{Li}}_2(1-{\mathrm{e}}^{2\mathrm{i}x})-\frac{\mathrm{i}}{2}{x}^2+C$$$$\mathrm{please}\mathrm{check}...$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com